Elastic shocks in one dimension, special cases, exercises

The elastic shocks or elastic collisions consist of brief but intense interactions between objects, in which both the momentum and the kinetic energy are conserved. Crashes are very frequent events in nature: from subatomic particles to galaxies, to billiard balls and bumper cars in amusement parks, they are all objects capable of colliding.

During a collision or collision, the interaction forces between objects are very strong, much more than those that can act externally. In this way it can be stated that during the collision, the particles form an isolated system.

In this case it is true that:

P_or = P_F

The amount of movement P_or before the collision is the same as after the collision. This is true for any type of collision, both elastic and inelastic..

Now consider the following: during a collision objects undergo a certain deformation. When the shock is elastic, objects quickly regain their original shape.

Article index

• 1 Conservation of kinetic energy
• 2 Elastic shocks in one dimension
  • 2.1 -Formulas for elastic collisions
• 3 Special cases in elastic collisions
  • 3.1 Two identical masses
  • 3.2 Two identical masses, one of which was initially at rest
  • 3.3 Two different masses, one of them initially at rest
• 4 Coefficient of restitution or Huygens-Newton rule
• 5 Exercises solved
  • 5.1 -Solved exercise 1
  • 5.2 -Solved exercise 2
  • 5.3 -Solved exercise 3
  • 5.4 -Solved exercise 4
• 6 References

Conservation of kinetic energy

Normally during a crash, part of the energy of objects is spent on heat, deformation, sound and sometimes even on producing light. So the kinetic energy of the system after the collision is less than the original kinetic energy.

When the kinetic energy K is conserved then:

K_or = K_F

Which means that the forces acting during the collision are conservative. During the collision, the kinetic energy is briefly transformed into potential energy and then back to kinetic energy. The respective kinetic energies vary, but the sum remains constant.

Perfectly elastic collisions are rare, although billiard balls are a fairly good approximation, as are collisions that occur between ideal gas molecules..

Elastic shocks in one dimension

Let's examine a collision of two particles of this in a single dimension; that is, the interacting particles move, say, along the x-axis. Suppose they have masses m₁ Y m_two. The initial velocities of each are or₁ Y or_two respectively. Final speeds are v₁ Y v_two.

We can dispense with the vector notation, since the movement is carried out along the x axis, however, the signs (-) and (+) indicate the direction of the movement. On the left is negative and on the right positive, by convention.

-Formulas for elastic collisions

For the amount of movement

m₁or₁ + m_twoor_two = m₁v₁ + m_twov_two

For kinetic energy

½ m₁or^two₁ + ½ m_twoor^two_two = ½ m₁v^two₁ +  ½ m_twov^two_two

Provided the masses and initial velocities are known, the equations can be regrouped to find the final velocities.

The problem is that in principle, it is necessary to carry out a little tedious algebra, since the equations for kinetic energy contain the squares of the speeds, which makes the calculation a bit cumbersome. The ideal would be to find expressions that do not contain them.

The first thing is to do without the factor ½ and rearrange both equations in such a way that a negative sign appears and the masses can be factored:

m₁or₁ - m₁v₁ = M_twov_two - m_twoor_two

m₁or^two₁ - m₁v^two₁  = + M_twov^two_two - m_twoor^two_two

Being expressed in this way:

m₁(or₁ - v₁ ) = m_two(v_two - or_two)

m₁(or^two₁ - v^two₁ ) = m_two (v^two_two - or^two_two)

Simplification to eliminate the squares of the velocities

Now we must make use of the product notable sum by its difference in the second equation, with which we obtain an expression that does not contain the squares, as originally wanted:

m₁(or₁ - v₁ ) = m_two(v_two - or_two)

m₁(or₁ - v₁ ) (or₁ + v₁ ) = m_two (v_two - or_two) (v_two + or_two)

The next step is to substitute the first equation in the second:

m_two(v_two - or_two) (or₁ + v₁ ) = m_two (v_two - or_two) (v_two + or_two)

And when the term is repeated m_two(v_two - or_two) on both sides of the equality, said term is canceled and looks like this:

(or₁ + v₁) = (v_two + or_two)

Or even better:

or₁ - or_two= v_two -  v₁

Final speeds v₁ and V_two of the particles

Now you have two linear equations that are easier to work with. We will put them back one below the other:

m₁or₁ + m_twoor_two = m₁v₁ + m_twov_two

or₁ - or_two= v_two -  v₁

Multiplying the second equation by m₁ and adding term to term is:

m₁or₁ + m_twoor_two = m₁v₁ + m_twov_two

m₁or₁ - m₁or_two= m₁v_two - m₁ v₁

-

2 m₁or₁ + (m_two - m₁) or_two = (m_two + m₁) v_two

And it is already possible to clear v_two. For example:

Special cases in elastic collisions

Now that equations are available for the final velocities of both particles, it is time to analyze some special situations.

Two identical masses

Then m₁ = m_two = m Y:

v₁ = u_two

v_two = u₁

The particles simply exchange their velocities after the collision.

Two identical masses, one of which was initially at rest

Again  m₁ = m_two = m and assuming that or₁ = 0:

v₁ = u_two

v_two = 0

After the collision, the particle that was at rest acquires the same speed as the particle that was moving, and this in turn stops.

Two different masses, one of them initially at rest

In this case suppose that or₁ = 0, but the masses are different:

What if m₁ is much greater than m_two?

It happens that m₁ is still at rest and m_two returns as quickly as it hit.

Coefficient of restitution or Huygens-Newton rule

Previously, the following relationship between the velocities was derived for two objects in elastic collision: or₁ - or_two = v_two -  v₁. These differences are the relative speeds before and after the collision. In general, for a collision it is true that:

or₁ - or_two = - (v₁ -  v_two)

The concept of relative velocity is best appreciated if the reader imagines that he is on one of the particles and from this position he observes the speed with which the other particle is moving. The above equation is rewritten like this:

Solved exercises

-Solved exercise 1

A billiard ball is moving to the left at 30 cm / s, colliding head-on with another identical ball that is moving to the right at 20 cm / s. The two balls have the same mass and the collision is perfectly elastic. Finding the velocity of each ball after impact.

Solution

or₁ = -30 cm / s

or_two = +20 cm / s

It is the special case in which two identical masses collide in one dimension elastically, therefore the speeds are exchanged.

v₁ = +20 cm / s

v_two = -30 cm / s

-Exercise solved 2

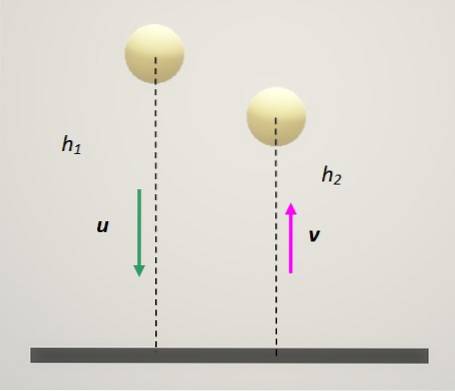
The coefficient of restitution of a ball that bounces off the ground is equal to 0.82. If it falls from rest, what fraction of its original height will the ball reach after bouncing once? And after 3 bounces?

Solution

Soil can be object 1 in the coefficient of restitution equation. And it always remains at rest, so that:

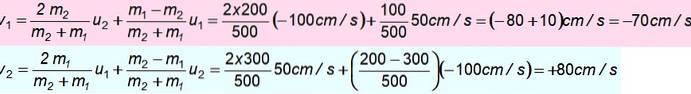
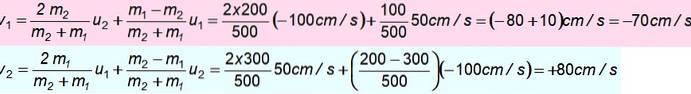
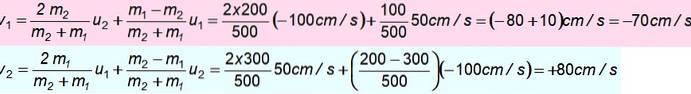
With this speed it bounces:

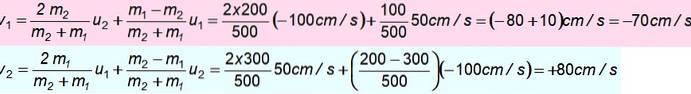
The + sign indicates that it is an ascending speed. And according to it, the ball reaches a maximum height of:

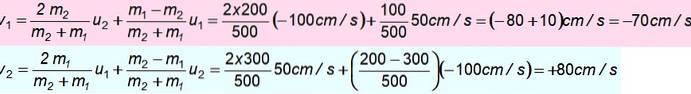
Now it returns to the ground again with a speed of equal magnitude, but opposite sign:

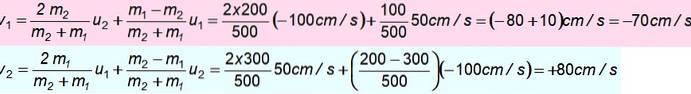
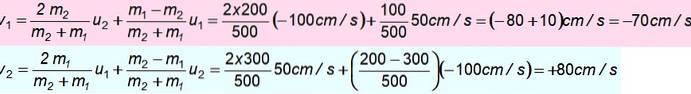
This achieves a maximum height of:

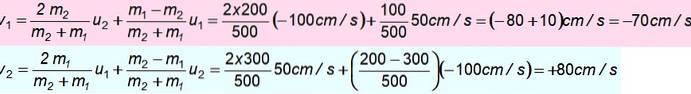
Get back to the ground with:

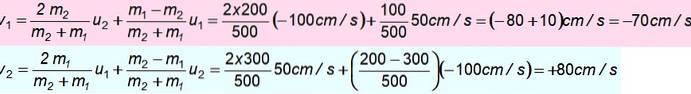
Successive bounces

Every time the ball bounces and rises, multiply the speed again by 0.82:

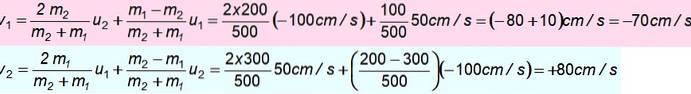
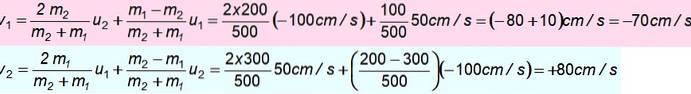
By now h₃ is about 30% of h_or. What would be the height to the 6th bounce without the need to make such detailed calculations as the previous ones?

Would h₆ = 0.82¹² h_or = 0.092h_or or just 9% of h_or.

-Exercise solved 3

A 300-g block is moving north at 50 cm / s and collides with a 200-g block heading south at 100 cm / s. Assume that the shock is perfectly elastic. Find the velocities after impact.

Data

m₁ = 300 g; or₁ = + 50 cm / s

m_two = 200 g; or_two = -100 cm / s

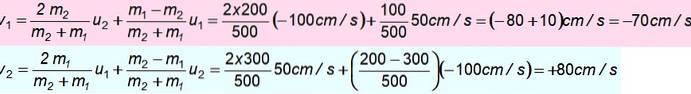
-Exercise solved 4

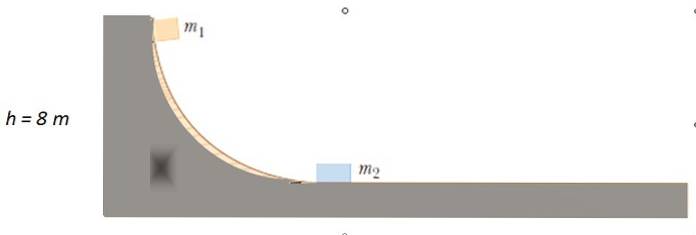
A mass of m is released₁ = 4 kg from the indicated point on the frictionless track, until it collides with m_two = 10 kg at rest. How high does m rise?₁ after the collision?

Solution

Since there is no friction, mechanical energy is conserved to find the velocity or₁ with what m₁ impacts  m_two. Initially the kinetic energy is 0, since m₁ part of rest. When it moves on the horizontal surface it has no height, so the potential energy is 0.

mgh = ½ mu₁ ^two

or_two = 0

Now the velocity of m₁ after the collision:

The negative sign means that it has been returned. With this speed it rises and the mechanical energy is conserved again to find h ', the height to which you can ascend after the crash:

½ mv₁^two = mgh '

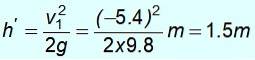
Note that it does not return to the starting point at an altitude of 8 m. It does not have enough energy because the mass gave part of its kinetic energy m_1.

References

1. Giancoli, D. 2006. Physics: Principles with Applications. 6^th. Ed Prentice Hall. 175-181
2. Rex, A. 2011. Fundamentals of Physics. Pearson. 135-155.
3. Serway, R., Vulle, C. 2011. Fundamentals of Physics. 9^na Cengage Learning. 172-182
4. Tipler, P. (2006) Physics for Science and Technology. 5th Ed. Volume 1. Editorial Reverté. 217-238
5. Tippens, P. 2011. Physics: Concepts and Applications. 7th Edition. MacGraw Hill. 185-195