
Dynamic history, what it studies, laws and theories

The dynamic It is the area of mechanics that studies the interactions between bodies and their effects. It deals with describing them qualitatively and quantitatively, as well as predicting how they will evolve over time.
Applying its principles, it is known how the movement of a body is modified when interacting with others, and also if these interactions deform it, since it is perfectly possible that both effects occur at the same time..

The beliefs of the great Greek philosopher Aristotle (384-322 BC) prevailed as the foundation of dynamics in the West for centuries. He thought that objects moved because of some kind of energy that pushed them in one direction or the other..
He also observed that while an object is being pushed, it moves at a constant speed, but when pushing is stopped, it moves more and more slowly until it stops..
According to Aristotle, the action of a constant force was necessary to make something move at constant speed, but what happens is that this philosopher did not have the effects of friction.
Another idea of his was that heavier objects fell faster than lighter ones. It was the great Galileo Galilei (1564-1642) who demonstrated through experiments that all bodies fall with the same acceleration regardless of their mass, neglecting viscous effects..
But it is Isaac Newton (1642-1727), the most remarkable scientist who has ever lived, who is considered the father of modern dynamics and mathematical calculation, along with Gottfried Leibniz.

Its famous laws, formulated during the 17th century, remain as valid and fresh today. They are the foundation of classical mechanics, which we see and affect us every day. These laws will be discussed shortly.
Article index
- 1 What study the dynamics?
- 1.1 Particles, rigid solids and continuous media
- 2 Newton's laws
- 2.1 Newton's First Law
- 2.2 Newton's Second Law
- 2.3 Newton's Third Law
- 3 Conservation principles
- 3.1 Conservation of energy
- 3.2 Conservation of momentum
- 4 Outstanding Concepts in Dynamics
- 4.1 Inertia
- 4.2 Mass
- 4.3 Weight
- 4.4 Reference systems
- 4.5 Fictitious forces
- 4.6 Acceleration
- 4.7 Work and energy
- 5 Related Topics
- 6 References
What does the dynamics study?
Dynamics studies the interaction between objects. When objects interact there are changes in their movement and also deformations. A particular area called static is dedicated to those systems in equilibrium, those that are at rest or with uniform rectilinear motion..
Applying the principles of dynamics it is possible to predict, by means of equations, what will be the changes and the evolution of objects in time. To do this, some assumptions are established depending on the type of system to be studied..
Particles, rigid solids and continuous media
The particle model is the simplest to start applying the principles of dynamics. In it it is assumed that the object to be studied has mass, but no dimensions. Therefore a particle can be as small as an electron or as big as the Earth or the Sun..
When you want to observe the effect of size on dynamics, it is necessary to consider the size and shape of objects. A model that takes this into account is that of the rigid solid, a body with measurable dimensions composed of many particles, but that does not deform under the effects of the forces.
Finally, the mechanics of continuous media takes into account not only the dimensions of the object, but also its particular characteristics, including its ability to deform. Continuous media encompasses rigid and non-rigid solids, as well as fluids.
Newton's laws

The key to understanding how dynamics works is fully understanding Newton's laws, which quantitatively link the forces acting on a body with changes in its state of motion or rest..
Newton's First Law

Says so:
When the net force on an object equals zero, the object will continue at rest if it was at rest. And if it was moving, its movement will be rectilinear and with constant speed.
The first part of the statement seems pretty obvious, since it is evident that an object at rest will stay that way, unless it is disturbed. And for this a force is required.
On the other hand, the fact that an object continues in motion even when the net force on it is zero is a bit more difficult to accept, since it seems that an object could stay in motion indefinitely. And everyday experience tells us that sooner or later things slow down.
The answer to this apparent contradiction is in friction. Indeed, if an object were to move on a perfectly smooth surface, it could do so indefinitely, assuming that no other force causes the motion to vary..
Since it is impossible to completely eliminate friction, the situation in which a body moves indefinitely at a constant speed is an idealization.
Finally, it is important to note that although the net force is zero, this does not necessarily represent a total absence of forces on the object..
Objects on the earth's surface always experience gravitational attraction. A book resting on a table remains that way, because the surface of the table exerts a force that counteracts the weight.
Second law of Newton
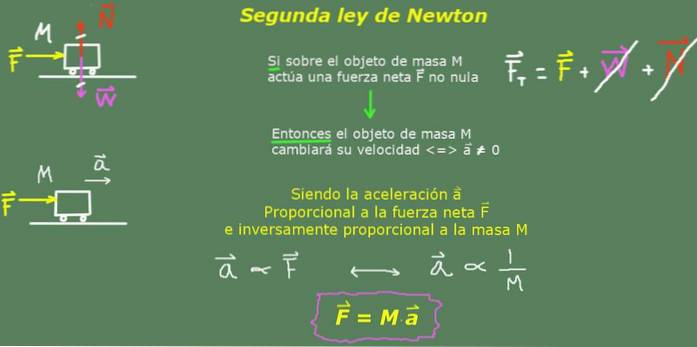
Newton's first law establishes what happens to an object on which the net or resultant force is zero. Now the fundamental law of dynamics or Newton's second law indicates what will happen when the net force does not cancel:
If an external net force F acts on an object of mass m, it will experience an acceleration proportional to the force and in the same direction. Mathematically:
Fnet = mto.
In effect, the greater an applied force, the greater the change in velocity of an object. And if the same force is applied to objects of different masses, the greatest changes will be experienced by objects that are lighter and easier to move. Everyday experience agrees with these assertions.
Newton's third law

Newton's first two laws refer to a single object. But the third law refers to two objects. We will name them object 1 and object 2:
When two objects interact, the forces that they exert on each other are always equal both in magnitude and direction, but in the opposite direction, which in mathematical form is expressed as follows:
F12 = -Ftwenty-one
In fact, whenever a body is affected by a force, it is because another is responsible for causing it. Thus, objects on the Earth have weight, because it attracts them towards its center. An electric charge is repelled by another charge of the same sign, because it exerts a repulsive force on the first, and thus.

Conservation principles
In dynamics there are several quantities that are conserved during movement and whose study is essential. They are like a solid column to which it is possible to attach to solve problems in which the forces vary in very complex ways..
An example: just when two vehicles collide, the interaction between them is very intense but brief. So intense that no other forces need to be taken into account, therefore the vehicles can be considered as an isolated system.
But describing this intense interaction is not an easy task, since it involves forces that vary in time and also in space. However, assuming that the vehicles constitute an isolated system, the forces between the two are internal, and the momentum is conserved..
By conserving the momentum it is possible to predict how the vehicles will move right after the collision.
Here are two of the most important conservation principles in Dynamics:
Energy conservation
In nature there are two types of forces: conservative and non-conservative. Weight is a good example of the former, while friction is a good example of the latter..
Well, conservative forces are characterized because they offer the possibility of storing energy in the configuration of the system. It is the so-called potential energy.
When a body has potential energy thanks to the action of a conservative force such as weight and goes into motion, this potential energy is converted into kinetic energy. The sum of both energies is called the mechanical energy of the system and is the one that is conserved, that is, it remains constant.
Be OR potential energy, K kinetic energy and ANDm mechanical energy. If only conservative forces act on an object, it is true that:
ANDm = U + K = constant
Therefore:
ANDm initial = Em final
Conservation of momentum
This principle is applicable not only when two vehicles collide. It is a law of physics with a scope that goes beyond the macroscopic world.
The momentum is conserved at the level of the solar, stellar and galaxy systems. And it also does it at the scale of the atom and the atomic nucleus, although Newtonian mechanics ceases to be valid there.
Be P the momentum vector given by:
P = m.v
Drifting P with respect to time:
dP / dt = d [m.v] / dt
If the mass remains constant:
dP / dt = m dv/ dt = m.to
Therefore we can write Newton's second law like this:
Fnet = dP / dt
If two bodies m1 and mtwo make up an isolated system, the forces between them are internal and according to Newton's third law, they are equal and opposite F1 = -Ftwo, being fulfilled that:
dP1 / dt = - dPtwo/ dt → d [P1 + Ptwo] / dt = 0
If the derivative with respect to time of a magnitude is zero, it means that the magnitude remains constant. Therefore, in an isolated system, it can be stated that the momentum of the system is conserved:
P1 + Ptwo = constant
Even so, P1 Y Ptwo they may vary individually. The momentum of a system can be redistributed, but what matters is that its sum remains unchanged.
Featured concepts in dynamics
There are many important concepts in dynamics, but two of them stand out: mass and force. On the force already commented previously and below is a list with the most prominent concepts that appear next to it in the study of dynamics:
Inertia
It is the property of objects to resist changes in their state of rest or movement. All objects with mass have inertia and it is experienced very frequently, for example when traveling in an accelerating car, passengers tend to remain at rest, which is perceived as a sensation of sticking to the back of the seat.
And if the car stops abruptly, the passengers tend to fall flat on their faces, following the forward movement they had previously, so it is important to always wear seat belts.

Mass
Mass is the measure of inertia, since the greater the mass of a body, the more difficult it is to move it or cause it to change its motion. Mass is a scalar quantity, this means that to specify the mass of a body it is necessary to give the numerical value plus the selected unit, which can be kilos, pounds, grams and more..
Weight
Weight is the force with which the Earth pulls objects close to its surface toward its center..
Because it is a force, weight has a vector character, therefore it is completely specified when its magnitude or numerical value, its direction and its sense are indicated, which we already know is vertically down.
Thus, although related, weight and mass are not equal, not even equivalent, since the first is a vector and the second a scalar.
Reference systems
The description of a movement may vary depending on the chosen reference. Those who are going up in an elevator are at rest according to a fixed reference frame to it, but seen by an observer on the ground the passengers are moving.
If a body experiences motion about one frame of reference but is at rest in another, Newton's laws cannot apply to both. In fact, Newton's laws are applicable to certain frames of reference: those that are inertial.
In the inertial reference frames, bodies do not accelerate unless disturbed in some way - by applying a force-.
Fictitious forces
The fictitious forces or pseudo-forces appear when the movement of a body in an accelerated reference frame is analyzed. A fictitious force is distinguished because it is not possible to identify the agent responsible for its appearance.
Centrifugal force is a good example of fictitious force. However, the fact that it is does not make it any less real for those who experience it when they turn in their cars and feel that an invisible hand is pushing them out of the curve..
Acceleration
This important vector has already been mentioned before. An object experiences acceleration as long as there is a force that causes its speed to vary.
Work and energy
When a force acts on an object and it changes its position, the force has done work. And this work can be stored in the form of energy. Therefore, the work is carried out on the object, thanks to which it acquires the energy.
The following example clarifies the point: suppose a person raises a pot a certain height above ground level.
To do this, it must apply a force and overcome gravity, therefore it does work on the pot and this work is stored in the form of gravitational potential energy in the pot, proportional to its mass and the height it reached above the floor. :
U = m.g.h
Where m is the mass, g it's gravity and h Is the height. What can the pot do once it is up to par h? Well, it could fall and as it falls, the gravitational potential energy that it has decreases, while the kinetic or motion energy increases..
For a force to do work, it must produce a displacement that must be parallel to the force. If this does not happen, the force still acts on the object, but does not do work on it..
Related topics
Newton's first law.
Second law of Newton.
Newton's third law.
Law of conservation of matter.
References
- Bauer, W. 2011. Physics for Engineering and Sciences. Volume 1. Mc Graw Hill.
- Figueroa, D. 2005. Series: Physics for Sciences and Engineering. Volume 2. Dynamics. Edited by Douglas Figueroa (USB).
- Giancoli, D. 2006. Physics: Principles with Applications. 6th… Ed Prentice Hall.
- Hewitt, Paul. 2012. Conceptual Physical Science. 5th. Ed. Pearson.
- Kirkpatrick, L. 2007. Physics: A Look at the World. 6th abridged edition. Cengage Learning.
- Knight, R. 2017. Physics for Scientists and Engineering: a Strategy Approach. Pearson.
- Wikipedia. Dynamic. Recovered from: es.wikipedia.org.



Yet No Comments