Electric field flux

What is electric field flux?

The electric field flow or simply electric flux is a scalar quantity proportional to the number of electric field lines that pass through a surface. It is denoted by the capital Greek letter Φ (phi).

The electric field does not actually "flow" in the way that a stream of water does, although the streamlines of the fluid resemble those of the electric field..

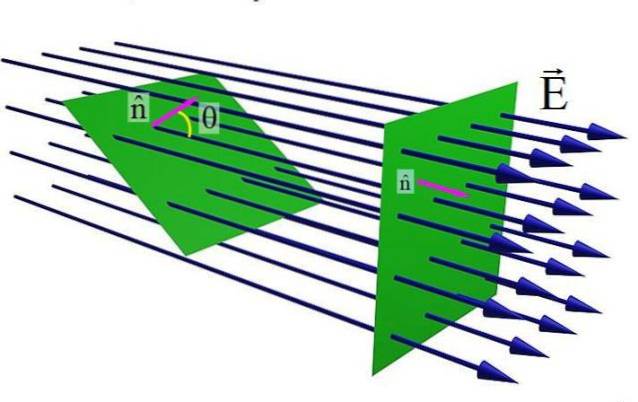
The figure above shows a flat surface traversed by an electric field AND. When the unit vector normal to the surface n and the field AND are parallel, the number of field lines that cross the surface is maximum. But as the angle θ between n Y AND, the number of lines passing through the green surface is less.

On the other hand, the electric field flux also depends on the magnitude of AND, because the greater this, the more field lines cross the surface. And of course, the greater the area S of said surface, the greater the flow, so the following equation is established:

Φ = E ∙ Scosθ

This expression is consistent with the dot product between the vectors AND Y n:

Φ = (AND • n) S

The unit for electric field flux in the SI International System of Units is N.m^two/ C (newton x square meter / coulomb). Alternatively, since the field is also measured in V / m (volt over meter), the electric flux remains in (V ∙ m).

Examples

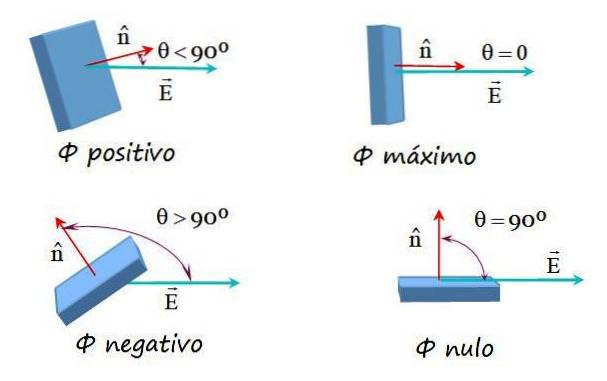
According to the definition, the electric flux can be positive, negative or equal to 0. The electric field flux is:

-Positive when angle θ between AND Y n is less than 90º, since cos θ is greater than zero.

-Negative if said angle is greater than 90º, because then cos θ is less than zero.

-Null when θ is exactly 90º, because cos 90º = 0 and the field lines in this case are tangential to the surface.

-On the other hand, if the angle between AND Y n is equal to 0, the flow acquires its maximum value.

These possibilities are shown in the following image:

Electric field flux on an arbitrary surface

Previously, the electric field flux was determined in the particular case of a uniform field incident on a flat surface. For a surface of arbitrary shape S and / or a non-uniform electric field, the angle between AND Y n may vary from point to point.

In the following figure there are two examples, on the left a curved surface and on the right a closed surface.

In both cases, the surface is divided into much smaller regions, of infinitesimal size, called dS, through which a flow also infinitesimal dΦ passes through:

dΦ = (AND•n) dS = (Ecosθ) dS

The total field is obtained by adding all these infinitesimal contributions:

In the case of closed surfaces, n always points outwards, therefore the flow has a + sign when it is salient to S, since the angle between AND Y n is less than 90º, and sign - when the field is incoming, because then the angle between AND Y n is greater than 90º (see figure 2).

Note that in the closed surface on the right, the number of field lines entering the surface is equal to the number of lines leaving. Therefore the net flow, defined as the algebraic sum of the incoming flow and the outgoing flow, is zero.

The source of the electric field in this case is outside the surface, however, the net flux would be different from 0 if the source of the electric field (the charge distribution) was inside the surface..

Training

Exercise 1

It has an electric field AND = 3.5 kN / C x and a flat rectangular surface 0.35 m wide by 0.7 m long. Find the electric field flux through the rectangle in the following cases:

a) The surface is parallel to the yz plane.

b) The rectangle is parallel to the xy plane.

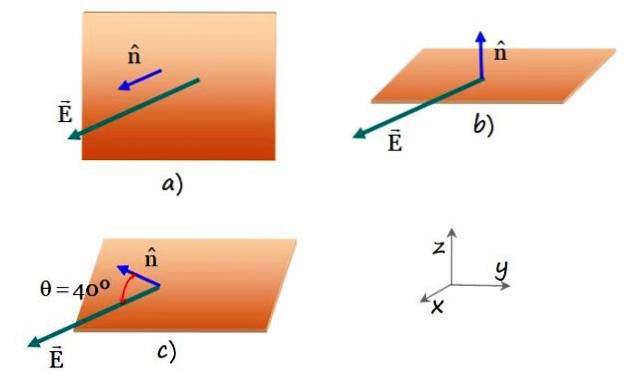
c) The normal of the plane forms an angle of 40º with the x-axis and contains the y-axis.

Solution to

The normal vector and the electric field vector are parallel, therefore the angle θ between them is 0º and the electric flux is:

Φ = (E ∙ S) cos 0 = E ∙ S

The area S of the rectangle is:

S = 0.35 m x 0.7 m = 0.245 m^two

Substituting in Φ:

Φ = E ∙ S = 3.5 x 10³ N / C × 0.245 m^two = 857.5 N ∙ m^two / C.

Solution b

The electric field flux is 0, since the vectors AND Y n are perpendicular to each other.

Solution c

The angle θ between the field AND and the normal vector n is 40º (see figure), therefore:

Φ = E ∙ S ∙ cos θ = 3.5 x 10³ N / C × 0.245 m^two × cos 40º = 656.9 N ∙ m^two / C.

Exercise 2

Calculate the electric field flux that produces a positive point charge q_or = 2μC located in the center of a sphere of radius R = 5 cm.

Solution

The field produced by the charge q_or It is not uniform, but from Coulomb's law it is known that on the surface of the sphere, it has a magnitude of:

The field has a radial direction, and the normal vector n, therefore the angle between both vectors is 0 at every point on the spherical surface. Substituting in:

It has to:

The integral of dS over the entire spherical surface S is its area, which is 4πR^two, Thus:

Its value is:

Φ = 4π × 9 × 10⁹ x 2 × 10^-6 N⋅m^two/ C = 2.3 x 10⁵ N⋅m^two/ C

References

1. Bauer, W. 2011. Physics for Engineering and Sciences. Volume 1. Mc Graw Hill.
2. Figueroa, D. (2005). Series: Physics for Science and Engineering. Volume 5. Electrostatics. Edited by Douglas Figueroa (USB).
3. Giambattista, A. 2010. Physics. 2nd. Ed. McGraw Hill.
4. Giancoli, D. 2006. Physics: Principles with Applications. 6th. Ed prentice hall.
5. Sears, Zemansky. 2016. University Physics with Modern Physics. 14th. Ed. Volume 1. Pearson.