
Homographic function how to graph, solved exercises
The it workedhomographic or rational ion It is a type of mathematical function composed by the division of two polynomial components. It obeys the form P (x) / Q (x), where Q (x) cannot take a null form.
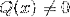
For example the expression (2x - 1) / (x + 3) corresponds to a homographic function with P (x) = 2x - 1 and Q (x) = x + 3.

The homographic functions constitute a section of study of the analytical functions, being treated from the graphing approach and from the study of the domain and range. This is due to the restrictions and grounds that must be applied for their resolutions..
Article index
- 1 What is a homographic function?
- 2 Mixed homographic function
- 2.1 Even nth root of the homographic function
- 2.2 Logarithm of the homographic function
- 3 How to graph a homographic function?
- 3.1 Roots
- 3.2 Vertical asymptote
- 3.3 Horizontal asymptote
- 3.4 Growth interval
- 3.5 Decay interval
- 3.6 Intersection with Y
- 4 Examples
- 4.1 Exercise 1
- 4.2 Exercise 1.2
- 5 Exercise 2
- 6 References
What is a homographic function?
They are rational expressions of a single variable, although this does not mean that there does not exist a similar expression for two or more variables, where it would already be in the presence of bodies in space that obey the same patterns as the homographic function in the plane.
They have real roots in some cases, but the existence of vertical and horizontal asymptotes is always maintained, as well as intervals of growth and decrease. Commonly only one of these trends is present, but there are expressions capable of showing both in their development..
Its domain is restricted by the roots of the denominator, since there is no division by zero of the real numbers.
Mixed homographic function
They are very frequent in the calculation, especially differential and integral, being necessary to derive and antiderivide under particular formulas. Some of the most common are classified below.
Even nth root of the homographic function
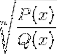
Exclude all elements from the domain that make the argument negative. The roots present in each polynomial yield values of zero when evaluated.
These values are accepted by the radical, although the fundamental restriction of the homographic function must be considered. Where Q (x) cannot receive null values.
The solutions of the intervals must be intercepted:
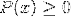
To achieve the solution of the intersections, the method of signs can be used, among others.
Logarithm of the homographic function
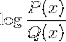

It is also common to find both expressions in one, among other possible combinations.
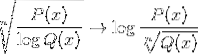
How to graph a homographic function?
Homographic functions correspond graphically to hyperbolas in the plane. Which are transported horizontally and vertically according to the values that define the polynomials.
There are several elements that we must define to graph a rational or homographic function.
Estate
The first will be the roots or zeros of the functions P and Q.

The achieved values will be denoted on the x-axis of the graph. Indicating the intersections of the graph with the axis.
Vertical asymptote
They correspond to vertical lines, which demarcate the graph according to the trends that these present. They touch the x-axis at the values that make the denominator zero and will never be touched by the graph of the homographic function.
Horizontal asymptote
Represented by a horizontal stitch line, it demarcates a limit for which the function will not be defined at the exact point. Trends will be observed before and after this line.
To calculate it we must resort to a method similar to L'Hopital's method, used to solve limits of rational functions that tend to infinity. We must take the coefficients of the highest powers in the numerator and denominator of the function.
For example, the following expression has a horizontal asymptote at y = 2/1 = 2.
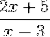
Growth interval
The ordinate values will have trends marked on the graph due to the asymptotes. In the case of growth, the function will increase in values as the elements of the domain are evaluated from left to right.
Decrease interval
The ordinate values will decrease as the domain elements are evaluated from left to right.
The jumps found in the values will not be taken into account as increases or decreases. This occurs when the graph is close to a vertical or horizontal asymptote, where the values can vary from infinity to negative infinity and vice versa..
Y intersection
By setting the value of x to zero, we find the intercept with the ordinate axis. This is a very useful piece of information for obtaining the graph of the rational function.
Examples
Define the graph of the following expressions, find their roots, vertical and horizontal asymptotes, intervals of increase and decrease and intersection with the ordinate axis.
Exercise 1
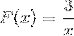
The expression has no roots, because it has a constant value in the numerator. The restriction to apply will be x different from zero. With horizontal asymptote at y = 0, and vertical asymptote at x = 0. There are no points of intersection with the y-axis.
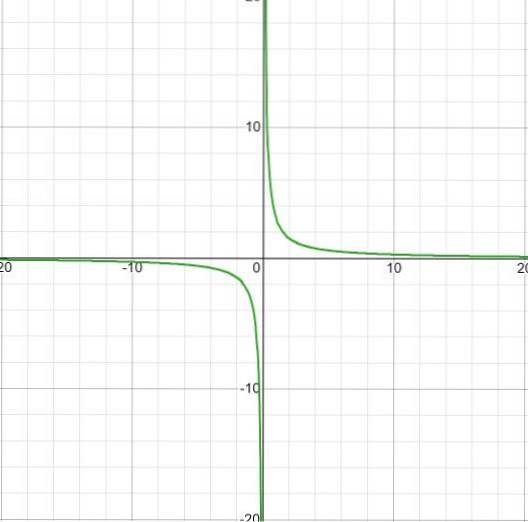
It is observed that there are no growth intervals even with the jump from minus to plus infinity at x = 0.
The decay interval is
ID: (-∞; o) U (0, ∞)
Exercise 1.2
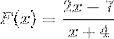
2 polynomials are observed as in the initial definition, so we proceed according to the established steps.
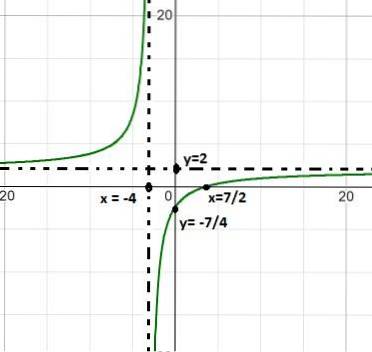
The root found is x = 7/2, which results from setting the function equal to zero.
The vertical asymptote is at x = - 4, which is the value excluded from the domain by the rational function condition.
The horizontal asymptote is at y = 2, this after dividing 2/1, the coefficients of the variables of degree 1.
It has a y-intercept = - 7/4. Found value after equating x to zero.
The function grows constantly, with a jump from plus to minus infinity around the root x = -4.
Its growth interval is (-∞, - 4) U (- 4, ∞).
When the value of x approaches minus infinity, the function takes values close to 2. The same happens when x approaches more infinity.
The expression approaches plus infinity when evaluating to - 4 from the left, and to minus infinity when evaluating to - 4 from the right.
Exercise 2
The graph of the following homographic function is observed:

Describe its behavior, roots, vertical and horizontal asymptotes, intervals of growth and decrease and intersection with the ordinate axis..
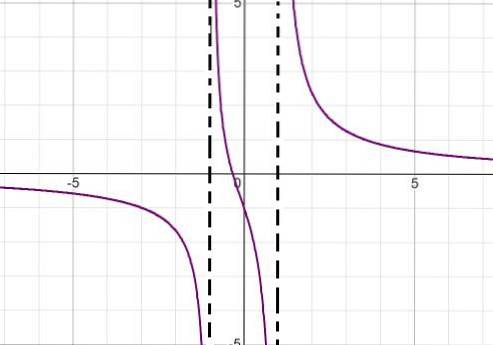
The denominator of the expression tells us by factoring the difference of squares (x + 1) (x - 1) the values of the roots. In this way, both vertical asymptotes can be defined as:
x = -1 and x = 1
The horizontal asymptote corresponds to the abscissa axis because the highest power is in the denominator.
Its only root is defined by x = -1/3.
The expression always decreases from left to right. It approaches zero when approaching infinity. Minus infinity as you approach -1 from the left. A plus infinity as it approaches -1 from the right. Minus infinity when approaching 1 from the left and more infinity when approaching 1 from the right.
References
- Approximation with Rational Functions. Donald J. Newman. American Mathematical Soc., Dec 31. 1979
- Orthogonal Rational Functions. UNIVERSIDAD DE LA LAGUNA TENERIFE ADHEMAR BULTHEEL, Adhemar Bultheel, Pablo Gonzalez-Vera, Erik Hendriksen, Olav Njastad. Cambridge University Press, Feb 13. 1999
- Rational Approximation of Real Functions. P. P. Petrushev, Vasil Atanasov Popov. Cambridge University Press, Mar 3. 2011
- Algebraic Functions. Gilbert Ames Bliss. Courier Corporation, Jan 1 2004
- Journal of the Spanish Mathematical Society, Volumes 5-6. Spanish Mathematical Society, Madrid 1916


Yet No Comments