Heptadecagon properties, diagonals, perimeter, area

The heptadecagon is a regular polygon with 17 sides and 17 vertices. Its construction can be done in the Euclidean style, that is, using only the ruler and the compass. It was the great mathematical genius Carl Friedrich Gauss (1777-1855), barely 18 years old, who found the procedure for its construction in 1796. 

Apparently, Gauss was always very inclined by this geometric figure, to such an extent that from the day he discovered its construction he decided to be a mathematician. It is also said that he wanted the heptadecagon to be engraved on his tombstone.

Gauss also found the formula to determine which regular polygons have the possibility of being constructed with ruler and compass, since some do not have exact Euclidean construction.

Article index

• 1 Characteristics of the heptadecagon
• 2 Diagonals and perimeter
  • 2.1 Perimeter of the heptadecagon 
• 3 Area
  • 3.1 Area given the side
  • 3.2 Area given the radius
• 4 Examples
  • 4.1 Example 1
  • 4.2 Example 2
• 5 References

Characteristics of the heptadecagon

As for its characteristics, like any polygon, the sum of its internal angles is important. In a regular polygon of n sides, the sum is given by:

Sa (n) = (n -2) * 180º.

For the heptadecagon the number of sides n it is 17, which means that the sum of its internal angles is:

Sa (17) = (17 - 2) * 180º = 15 * 180º = 2700º.

This sum, expressed in radians, looks like this:

Sa (17) = (17 - 2) * π = 15 * π = 15π

From the above formulas it can be easily deduced that each internal angle of a heptadecagon has an exact measure α given by:

α = 2700º / 17 = (15/17) π radians

It follows that the internal angle in approximate form is:

α ≈ 158,824º

Diagonals and perimeter

Diagonals and perimeter are other important aspects. In any polygon the number of diagonals is: 

D = n (n - 3) / 2 and in the case of the heptadecagon, as n = 17, it is then that D = 119 diagonals.

On the other hand, if the length of each side of the heptadecagon is known, then the perimeter of the regular heptadecagon is found simply by adding 17 times that length, or what is equivalent to 17 times the length d On each side:

P = 17 d

Perimeter of the heptadecagon 

Sometimes only the radius is known r of the heptadecagon, so it is necessary to develop a formula for this case.

To this end, the concept of apothem. The apothem is the segment that goes from the center of the regular polygon to the midpoint of one side. The apothem relative to a side is perpendicular to that side (see figure 2).

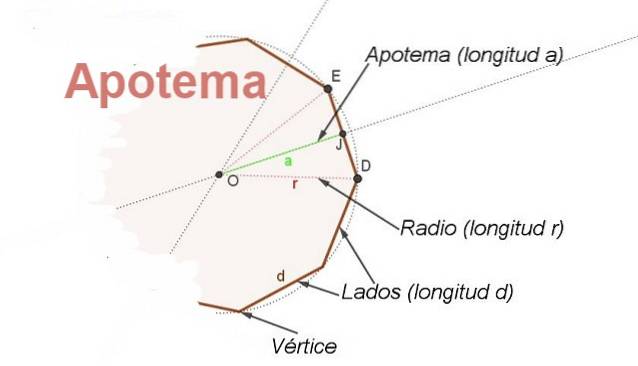
In addition, the apothem is the bisector of the angle with central vertex and sides on two consecutive vertices of the polygon, this allows finding a relationship between the radius r and the side d.

If it is called β to the central angle DOE and taking into account that the apothem OJ is bisector you have EJ = d / 2 = r Sen (β / 2), from where there is a relation to find the length d on the side of a known polygon its radius r and its central angle β:

d = 2 r Sen (β / 2)

In the case of the heptadecagon β = 360º / 17 so you have:

d = 2 r Sen (180º / 17) ≈ 0.3675 r

Finally, the formula for the perimeter of the heptadecagon, known its radius, is obtained:

P = 34 r Sen (180º / 17) ≈ 6.2475 r

The perimeter of a heptadecagon is close to the perimeter of the circumference that surrounds it, but its value is smaller, that is, the perimeter of the circumscribed circle is Pcir = 2π r ≈ 6.2832 r.

Area

To determine the area of ​​the heptadecagon we will refer to Figure 2, which shows the sides and apothem of a regular polygon of n sides. In this figure the triangle EOD has an area equal to the base d (polygon side) times height to (polygon apothem) divide by two:

EOD area = (d x a) / 2

So known the apothem to of the heptadecagon and the side d its area is:

Heptadecagon area = (17/2) (d x a)

Area given the side

To obtain a formula for the area of ​​the heptadecagon knowing the length of its seventeen sides, it is necessary to obtain a relation between the length of the apothem to and the side d.

Referring to figure 2, the following trigonometric relationship is obtained:

Tan (β / 2) = EJ / OJ = (d / 2) / a, being β to the central angle DOE. So the apothem to can be calculated if the length is known d from the side of the polygon and the central angle β:

a = (d / 2) Cotan (β / 2)

If we now substitute this expression for the apothem, in the formula for the area of ​​the heptadecagon obtained in the previous section, we have:

Heptadecagon area = (17/4) (d^two) Cotan (β / 2)

Being β = 360º / 17 for the heptadecagon, so we finally have the desired formula:

Heptadecagon area = (17/4) (d^two) Cotan (180º / 17)

Area given the radius

In the previous sections, a relationship had been found between the side d of a regular polygon and its radius r, this relationship being the following:

d = 2 r Sen (β / 2)

This expression for d is introduced in the expression obtained in the previous section for the area. If the pertinent substitutions and simplifications are made, the formula that allows to calculate the area of ​​the heptadecagon is obtained:

Heptadecagon area = (17/2) (r^two) Sen (β) = (17/2) (r^two) Sen (360º / 17)

An approximate expression for the area is:

Heptadecagon area = 3.0706 (r^two) 

As expected, this area is slightly smaller than the area of ​​the circle that encloses the heptadecagon. TO_circ = π r^two ≈ 3.1416 r^two. To be precise, it is 2% less than that of its circumscribed circle.

Examples

Example 1

For a heptadecagon to have sides of 2 cm, what value must the radius and diameter of the circumscribed circumference have? Also find the value of the perimeter.

To answer the question, it is necessary to remember the relationship between the side and the radius of a regular n-sided polygon:

 d = 2 r Sen (180º / n)

For the heptadecagon n = 17, so that d = 0.3675 r, that is, the radius of the heptadecagon is r = 2 cm / 0.3675 = 5.4423 cm or

10.8844 cm diameter.

The perimeter of a 2 cm side heptadecagon is P = 17 * 2 cm = 34 cm.

Example 2

What is the area of ​​a regular heptadecagon with a side 2 cm?

We must refer to the formula shown in the previous section, which allows us to find the area of ​​a heptadecagon when it has the length d on your side:

Heptadecagon area = (17/4) (d^two) / Tan (180º / 17) 

When substituting d = 2 cm in the above formula you get:

Area = 90.94 cm

References

1. C. E. A. (2003). Elements of geometry: with exercises and geometry of the compass. University of Medellin.
2. Campos, F., Cerecedo, F. J. (2014). Mathematics 2. Grupo Editorial Patria.
3. Freed, K. (2007). Discover Polygons. Benchmark Education Company.
4. Hendrik, V. (2013). Generalized Polygons. Birkhäuser.
5. IGER. (s.f.). Mathematics First Semester Tacaná. IGER.
6. Jr. geometry. (2014). Polygons. Lulu Press, Inc.
7. Miller, Heeren, & Hornsby. (2006). Mathematics: Reasoning And Applications (Tenth Edition). Pearson Education.
8. Patiño, M. (2006). Mathematics 5. Editorial Progreso.
9. Sada, M. 17-sided regular polygon with ruler and compass. Recovered from: geogebra.org
10. Wikipedia. Heptadecagon. Recovered from: es.wikipedia.com