
Grashof's law cases, mechanisms, examples, applications
The Grashof's law states that: In a flat mechanism with four articulated bars with one of them fixed, at least one of the bars can make a complete turn, provided that the sum of the shortest bar and the longest bar is less than or equal to the sum of the other two.
There are five flat four-bar or link mechanisms that comply with Grashof's law (An example is shown in Figure 1). In order for the bars or links of the mechanisms that comply with the law to make a complete turn, it is necessary that in a real arrangement, each bar is occupying different parallel planes.
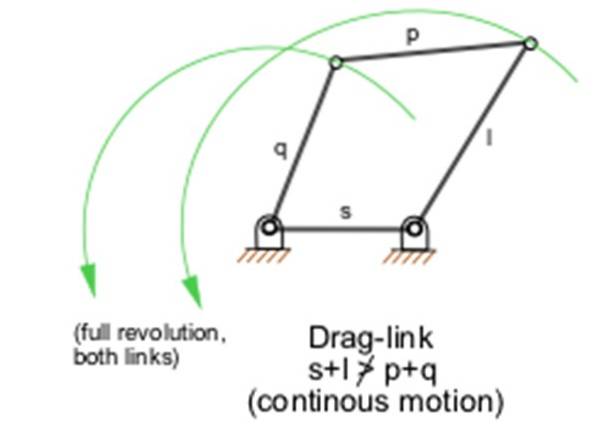
Grashof's law is a simple rule that allows you to design a mechanism in which full rotation is required, either because a motor will be connected or, on the contrary, because you want to transform an oscillatory movement into a rotary one, in such a way that it is mathematical and physically viable.
Article index
- 1 Borderline cases
- 1.1 Types of movement
- 2 Mechanisms that comply with Grashof's law
- 2.1 - Double crank mechanism
- 2.2 - More mechanisms that comply with Grashof's law
- 3 Applications
- 3.1 Crank-rocker mechanism
- 3.2 Articulated parallelogram mechanism
- 3.3 Articulated anti parallelogram mechanism
- 4 References
Borderline cases
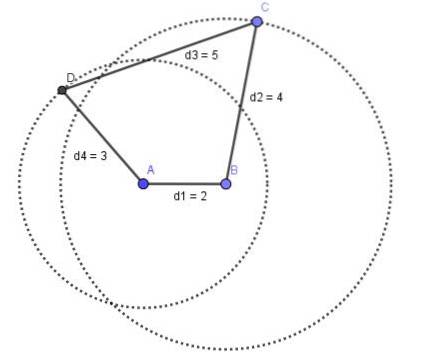
Suppose that the four link bars have the following lengths ordered from least to greatest according to:
s> p> q> l
Grashof's law states that for at least one bar or link to complete a revolution or turn, the condition must be met:
s + l <= p + q
This inequality has the following implications:
- The only bar or link that can give full revolutions with respect to another is the shortest bar.
- If the shorter bar goes full turns with respect to another, then it will also make full turns with respect to all others.
Types of movement
The movement of the articulated quadrilateral that complies with Grashof's law can be of the following types:
- Double turn or crank, if the shortest bar is the fixed one and the adjacent bars make complete turns.
- Back and forth, if the short bar is adjacent to the fixed bar.
- Double rocker, as long as the shortest bar is opposite the fixed one.
When the equality is fulfilled in the Grashof formula, then we are in the limiting case in which the sum of the shortest bar with the longest bar is equal to the sum of the other two.
In this case, the mechanism can take a configuration in which the four bars are aligned. And it is in this position, the non-fixed joints can indifferently go one way or the other, causing the mechanism to lock.
Mechanisms that meet Grashof's condition are more reliable and suffer less stress on their joints and links, to the extent that they are further from the limiting case of equality.
Mechanisms that comply with Grashof's law
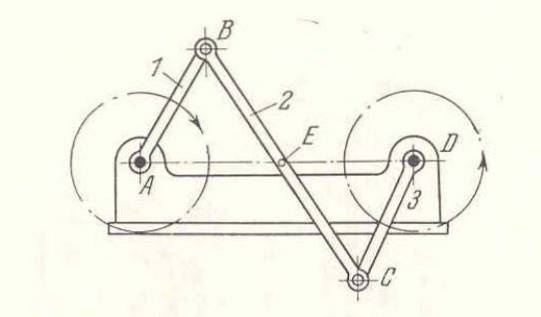
We will denote the consecutive joints with A, B, C and D, then:
- A and B are fixed pivots.
- AB = d1 (fixed bar)
- BC = d2
- CD = d3
- DA = d4
- Double crank mechanism
The bars b2 and b4 rotate completely and Grashof's law is fulfilled:
d1 + d3 <= d2+d4.
- More mechanisms that comply with Grashof's law
The characteristics of other mechanisms that comply with Grashof's law are named and described below:
Crank mechanism - rocker
D2 + d3 is fulfilled <= d1 + d4
The shorter bar d2 rotates fully and the opposite bar d4 makes a rocker movement.
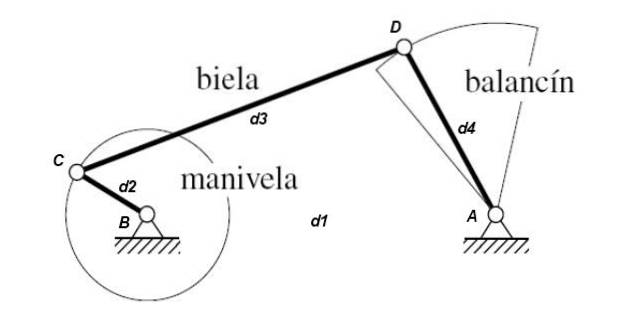
Double rocker mechanism
- The fixed bar AB is greater than the opposite bar CD and fulfills that:
d1 + d3 <= d2 + d3
- For the shorter bar (the opposite of the fixed bar), it is capable of a full turn.
Articulated parallelogram mechanism
- Bars AD and BC are of equal length and always parallel.
- For their part, the bars AB and CD are of equal length and always parallel.
- In the case of opposite bars, they have the same length and d1 + d2 = d3 + d4 is fulfilled, according to Grashof's law.
- Finally, the bars AD and BC turn completely in the same direction.
Articulated Anti-Parallelogram
- Bars AD and BC are of equal length and not parallel.
- For bars AB and CD, they must be of equal length and not parallel.
- On the other hand, the opposite bars have the same length, two of them are crossed.
- In this mechanism, the following condition must be met:
d1 + d2 = d3 + d4
- The rotation of the bars AD and BC is complete but in opposite directions.
Applications
Mechanisms that comply with Grashof's law have multiple applications:
Crank Mechanism - Rocker
It is applied to the pedal sewing machine, useful in the places where there is no electricity, in which the pedal makes a rocking or rocking movement, which is transmitted to a wheel connected by a pulley to the sewing machine.
Another example to mention is the windshield wiper mechanism. In this, a motor is connected to the crank bar that performs complete turns, transmitting a rocker movement to the bar that moves the first brush of the system..
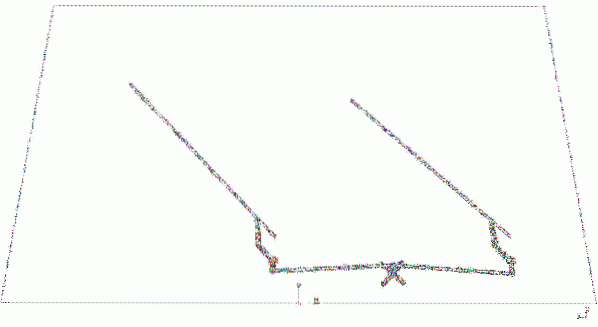
Another application of the crank-rocker mechanism is rocker arms for pumping oil from the ground..

A motor is connected to the crank that rotates completely and transmits the movement to the pumping head or rocker..
Articulated parallelogram mechanism
This mechanism used to be used to connect the wheels of steam locomotives, so that both wheels rotate in the same direction and at the same speed..
The main characteristic of this mechanism is that the bar that connects both wheels has the same length as the separation of their axles..
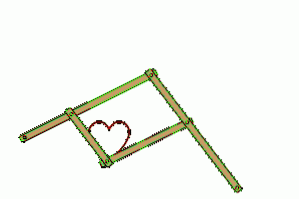
The pantograph is a drawing instrument used to copy and enlarge images. It is based on a four-bar mechanism, in which there are four joints that form the vertices of a parallelogram..
Articulated anti parallelogram mechanism
It is the mechanism used in the tennis ball throwing machine, where the wheels that drive and launch the ball are required to rotate in opposite directions..
References
- Clemente C. Virtual laboratory of a crank - rocker mechanism. Degree work in mechanical engineering. University of Almería. (2014). Recovered from: repositorio.ual.es
- Hurtado F. Grashof's Law. Recovered from: youtube.com
- Mech Designer. Kinematics Grashof criterion. Recovered from: mechdesigner.support.
- Shigley, J. Theory of machines and mechanisms. Mc-Graw Hill.
- We are F1. Four-bar mechanism analysis. Recovered from: youtube.com
- UNAM. Development of a four-bar mechanism for use in teaching. Recovered from: ptolomeo.unam.mx
- Wikipedia. Four-bar linkage. Recovered from: en.wikipedia.com
- Wikipedia. Grashof's law. Recovered from: es.wikipedia.com


Yet No Comments