Laws of Exponents (with Examples and Solved Exercises)

The laws of exponents are those that apply to that number that indicates how many times a base number must be multiplied by itself. The exponents are also known as powers. Empowerment is a mathematical operation formed by a base (a), the exponent (m) and the power (b), which is the result of the operation.

Exponents are generally used when very large quantities are used, because these are nothing more than abbreviations that represent the multiplication of the same number a certain amount of times. Exponents can be both positive and negative.

Article index

• 1 Explanation of the laws of exponents
  • 1.1 First law: power of exponent equal to 1
  • 1.2 Second law: power of exponent equal to 0
  • 1.3 Third law: negative exponent
  • 1.4 Fourth law: multiplication of powers with equal base
  • 1.5 Fifth law: division of powers with equal base
  • 1.6 Sixth law: multiplication of powers with different base
  • 1.7 Seventh law: division of powers with different base
  • 1.8 Eighth law: power of a power
  • 1.9 Ninth law: fractional exponent
• 2 Solved exercises
  • 2.1 Exercise 1
  • 2.2 Exercise 2
• 3 References

Explanation of the laws of exponents

As stated above, exponents are a shorthand form that represents multiplying numbers by themselves multiple times, where the exponent only relates to the number on the left. For example:

two³ = 2 * 2 * 2 = 8

In that case the number 2 is the base of the power, which will be multiplied 3 times as indicated by the exponent, located in the upper right corner of the base. There are different ways to read the expression: 2 raised to 3 or also 2 raised to the cube.

The exponents also indicate the number of times they can be divided, and to differentiate this operation from multiplication the exponent has the minus sign (-) in front of it (it is negative), which means that the exponent is in the denominator of a fraction. For example:

two^{- 4} = 1/2 * 2 * 2 * 2 = 1/16

This should not be confused with the case where the base is negative, as it will depend on whether the exponent is odd or even to determine whether the power will be positive or negative. So you have to:

- If the exponent is even, the power will be positive. For example:

(-7)^two = -7 _* -7 = 49.

- If the exponent is odd, the power will be negative. For example:

(-two)⁵ = (-2) * (- 2) * (- 2) * (- 2) * (- 2) = - 32.

There is a special case in which if the exponent is equal to 0, the power is equal to 1. There is also the possibility that the base is 0; in that case, depending on the exponent, the power will be indeterminate or not.

To perform mathematical operations with exponents, it is necessary to follow several rules or norms that make it easier to find the solution to these operations..

First law: power of exponent equal to 1

When the exponent is 1, the result will be the same value of the base: a¹ = a.

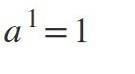
Examples

9¹ = 9.

22¹ = 22.

895¹ = 895.

Second law: power of exponent equal to 0

When the exponent is 0, if the base is nonzero, the result will be: a⁰ = 1.

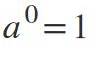
Examples

1⁰ = 1.

323⁰= 1.

1095⁰ = 1.

Third law: negative exponent

Since the exponte is negative, the result will be a fraction, where the power will be the denominator. For example, if m is positive, then a^-m = 1 / a^m.

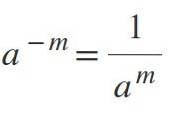
Examples

- 3^-1 = 1/3.

- 6^-two = 1/6^two = 1/36.

- 8^-3 = 1/8³ = 1/512.

Fourth law: multiplication of powers with equal base

To multiply powers where the bases are equal to and different from 0, the base remains and the exponents are added: a^m _* toⁿ = a^{m + n}.    

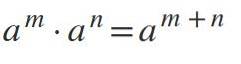
Examples

- 4⁴ _* 4³ = 4^{4 + 3} = 4⁷

- 8¹ _* 8⁴ = 8^{1 + 4} = 8⁵

- two^two _* two⁹ = 2^{2 + 9} = 2^eleven

Fifth law: division of powers with equal base

To divide powers in which the bases are equal to and different from 0, the base is kept and the exponents are subtracted as follows: a^m / toⁿ = a^m-n.    

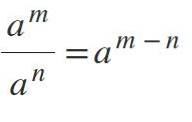
Examples

- 9^two / 9¹ = 9 ^(twenty-one) = 9¹.

- 6^fifteen / 6¹⁰ = 6 ^{(15 - 10)} = 6⁵.

- 49¹² / 49⁶ = 49 ^{(12 - 6)} = 49⁶.

Sixth law: multiplication of powers with different base

In this law there is the opposite of what is expressed in the fourth; that is, if you have different bases but with the same exponents, multiply the bases and keep the exponent: a^m _* b^m = (a_*b) ^m.

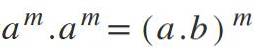
Examples

- 10^two _* twenty^two = (10 _* twenty)^two = 200^two.

- Four. Five^eleven _* 9^eleven = (45 * 9)^{11 =} 405^eleven.

Another way to represent this law is when a multiplication is raised to a power. Thus, the exponent will belong to each of the terms: (a_*b)^m= a^m_* b^m.

Examples

- (5_*8)⁴ = 5⁴ _* 8⁴ = 40⁴.

- (23 * 7)⁶ = 23⁶ _* 7⁶ = 161⁶.

Seventh law: division of powers with different base

If you have different bases but with the same exponents, divide the bases and keep the exponent: a^m / b^m = (a / b)^m.

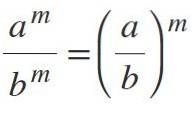
Examples

- 30³ / two³ = (30/2)³ = 15³.

- 440⁴ / 80⁴ = (440/80)⁴ = 5.5⁴.

Similarly, when a division is raised to a power, the exponent will belong in each of the terms: (a / b) ^m = a^m / b^m.

Examples

- (8/4)⁸ = 8⁸ / 4⁸ = 2⁸.

- (25/5)^two = 25^two / 5^two = 5^two.

There is the case where the exponent is negative. Then, to be positive, the value of the numerator is inverted with that of the denominator, as follows:

- (a / b)^-n = (b / a)ⁿ = bⁿ / toⁿ.

- (4/5) ^-9 = (5/4) ⁹ = 5⁹ / 4⁴.

Eighth law: power of a power

When you have a power that is raised to another power -that is, two exponents at the same time-, the base is maintained and the exponents are multiplied: (a^m)ⁿ= a^{m *n}.

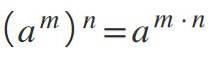
Examples

- (8³)^two = 8 ^{(3 * 2)} = 8⁶.

- (13⁹)³ = 13 ^{(9 * 3)} = 13²⁷.

- (238¹⁰)¹² = 238^{(10 * 12)} = 238¹²⁰.

Ninth law: fractional exponent

If the power has a fraction as an exponent, this is solved by transforming it into an nth root, where the numerator remains as an exponent and the denominator represents the index of the root:

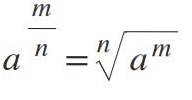
Example

Solved exercises

Exercise 1

Calculate the operations between the powers that have different bases:

two⁴ _* 4⁴ / 8^two.

Solution

Applying the rules of exponents, the bases are multiplied in the numerator and the exponent is maintained, like this:

two⁴ _* 4⁴ / 8^two= (2_*4)⁴ / 8^two  = 8⁴ / 8^two

Now, since we have the same bases but with different exponents, the base is kept and the exponents are subtracted:

 8⁴ / 8^two = 8^{(4 - 2)} = 8^two

Exercise 2

Calculate the operations between the powers raised to another power:

(3^two)³ _* (two _* 6⁵)^-two _* (two^two)³

Solution

Applying the laws, you have to:

(3^two)³ _* (two _* 6⁵)^-two _* (two^two)³

= 3⁶ _* two^-two _* two^-10 _* two⁶

= 3⁶ _* two^{(-2) + (- 10)} _* two⁶

= 3⁶ _*  two^-12 _* two⁶

= 3⁶ _* two^{(-12) + (6)}

= 3⁶ _* two⁶

= (3_*two)⁶

= 6⁶

= 46,656

References

1. Aponte, G. (1998). Basic Mathematics Fundamentals. Pearson Education.
2. Corbalán, F. (1997). Mathematics applied to everyday life.
3. Jiménez, J. R. (2009). Math 1 SEP.
4. Max Peters, W. L. (1972). Algebra and Trigonometry.
5. Rees, P. K. (1986). Reverte.