Heisenberg atomic model

What is Heisenberg's atomic model?

The Heisenberg atomic model (1927) introduces the uncertainty principle in the electron orbitals surrounding the atomic nucleus. The prominent German physicist laid the foundations of quantum mechanics to estimate the behavior of the subatomic particles that make up an atom.

Werner Heisenberg's uncertainty principle indicates that it is not possible to know with certainty the position and the linear momentum of an electron at the same time. The same principle applies to the variables time and energy; that is, if we have a clue about the position of the electron, we will not know the linear momentum of the electron, and vice versa.

In short, it is not possible to simultaneously predict the value of both variables. The foregoing does not imply that any of the aforementioned quantities cannot be precisely known. As long as it is separately, there is no impediment to obtain the interest value.

However, uncertainty occurs when it comes to knowing simultaneously two conjugate quantities, such as position and linear momentum, and time together with energy..

This principle arises due to a strictly theoretical reasoning, as the only viable explanation to give reason for scientific observations.

Characteristics of the Heisenberg atomic model

In March 1927 Heisenberg published his work On the perceptual content of kinematics and quantum theoretical mechanics, where you detailed the uncertainty or indeterminacy principle.

This principle, fundamental in the atomic model proposed by Heisenberg, is characterized by the following:

• The uncertainty principle emerges as an explanation that complements the new atomic theories about the behavior of electrons. Despite using measuring instruments with high precision and sensitivity, indeterminacy is still present in any experimental test.
• Due to the uncertainty principle, when analyzing two related variables, if you have an accurate knowledge of one of these, then the uncertainty about the value of the other variable will be increasing..
• The momentum and position of an electron, or other subatomic particle, cannot be measured at the same time.
• The relationship between both variables is given by an inequality. According to Heisenberg, the product of the variations of the linear momentum and the position of the particle is always greater than the quotient between the Plank constant (6.62606957 (29) × 10 ^{-3. 4} Jules x seconds) and 4π, as detailed in the following mathematical expression:

The legend corresponding to this expression is the following:

∆p: indeterminacy of the linear moment.

∆x: indeterminacy of position.

h: Plank's constant.

π: number pi 3.14.

• In view of the above, the product of the uncertainties has as a lower limit the ratio h / 4π, which is a constant value. Therefore, if one of the magnitudes tends to zero, the other must increase in the same proportion.
• This relationship is valid for all pairs of conjugate canonical quantities. For example: Heisenberg's uncertainty principle is perfectly applicable to the energy-time pair, as detailed below:

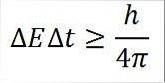
In this expression:

∆E: indeterminacy of energy.

∆t: indeterminacy of time.

h: Plank's constant.

π: number pi 3.14.

• From this model it follows that absolute causal determinism in conjugated canonical variables is impossible, since in order to establish this relationship one should have knowledge about the initial values ​​of the study variables..
• Consequently, the Heisenberg model is based on probabilistic formulations, due to the randomness that exists between the variables at subatomic levels..

Experimental tests

The Heisenberg uncertainty principle emerges as the only possible explanation for the experimental tests that took place during the first three decades of the 21st century..

Before Heisenberg enunciated the uncertainty principle, the precepts in force at that time suggested that the variables linear momentum, position, angular momentum, time, energy, among others, for subatomic particles were defined operationally.

This meant that they were treated as if they were classical physics; that is, an initial value was measured and the final value was estimated according to the pre-established procedure.

This implied defining a reference system for the measurements, the measuring instrument and the way of using said instrument, in accordance with the scientific method..

Accordingly, the variables described by subatomic particles had to behave in a deterministic way. That is, their behavior had to be predicted accurately and precisely..

However, each time a test of this nature was carried out, it was impossible to obtain the theoretically estimated value in the measurement.. 

The measurements were distorted due to the natural conditions of the experiment, and the result obtained was not useful to enrich the atomic theory.

Example

For example: if it is a question of measuring the speed and position of an electron, the set-up of the experiment must contemplate the collision of a photon of light with the electron.

This collision induces a variation in the speed and the intrinsic position of the electron, with which the object of the measurement is altered by the experimental conditions..

Therefore, the researcher encourages the occurrence of an unavoidable experimental error, despite the accuracy and precision of the instruments used..

Quantum mechanics other than classical mechanics

In addition to the above, Heisenberg's principle of indeterminacy states that, by definition, quantum mechanics works differently from classical mechanics.

Consequently, it is assumed that precise knowledge of measurements at the subatomic level is limited by the fine line that separates classical and quantum mechanics..

Limitations of the Heisenberg model

Despite explaining the indeterminacy of subatomic particles and establishing the differences between classical and quantum mechanics, Heisenberg's atomic model does not establish a single equation to explain the randomness of this type of phenomena..

Furthermore, the fact that the relationship is established through an inequality implies that the range of possibilities for the product of two conjugate canonical variables is indeterminate. Consequently, the uncertainty inherent in subatomic processes is significant..

Articles of interest

Schrödinger's atomic model.

Atomic de Broglie model.

Atomic model of Chadwick.

Atomic model of Perrin.

Thomson's atomic model.

Dalton's atomic model.

Dirac Jordan atomic model.

Atomic model of Democritus.

Atomic model of Leucippus.

Bohr atomic model.

Sommerfeld atomic model.

Current atomic model.

References

1. Beyler, R. (1998). Werner Heisenberg. Encyclopædia Britannica, Inc. Recovered from: britannica.com
2. The Heisenberg Uncertainty Principle (s.f.). Recovered from: hiru.eus
3. García, J. (2012). Heisenberg's uncertainty principle. Recovered from: hiberus.com
4. Atomic models (s.f.). National Autonomous University of Mexico. Mexico DF, Mexico. Recovered from: asesorias.cuautitlan2.unam.mx
5. Werner Heisenberg (s.f.). Recovered from: the-history-of-the-atom.wikispaces.com
6. Wikipedia, The Free Encyclopedia (2018). Plank's constant. Recovered from: es.wikipedia.org
7. Wikipedia, The Free Encyclopedia (2018). Heisenberg's indeterminacy relation. Recovered from: es.wikipedia.org