Uniform circular motion (M.C.U.) formulas, characteristics

A particle has circular movement uniform (M.C.U.) when its trajectory is a circumference and it also travels it with constant speed. Many objects such as parts of machinery and motors, for example, have this kind of movement, among which computer hard drives, fan blades, shafts and many other things stand out..

Uniform circular motion is also a good approximation for the motion of some celestial bodies such as the Earth. Actually the Earth's orbit is elliptical, as Kepler's laws indicate. However, the eccentricity of the orbit is small and as a first approximation it can be considered circular, which simplifies some calculations, such as finding the speed of the Earth when it moves around the Sun..

The description of uniform circular motion uses the same parameters as in rectilinear motion, namely: position, displacement, time, velocity, and acceleration..

Acceleration? Yes, indeed, uniform circular motion is accelerated, even though its speed v be constant. This is because the speed v, which is a vector and that is why it is bold, it continually changes its direction as the object or particle rotates. Any change in v is produced by an acceleration, which, as will be seen, is directed towards the center of the circular path.

Uniform circular motion is motion in the plane xy, therefore it is a movement in two dimensions. However, it is possible to express it more conveniently by the angle θ that the particle sweeps, measured with respect to the horizontal axis or another suitable reference axis..

Even if it is an extended object, its particles always sweep the same angle, even if they have different coordinates. (x, y).

Article index

• 1 Characteristics of uniform circular motion
• 2 Formulas of uniform circular motion
  • 2.1 Position vector
  • 2.2 Angular velocity and linear velocity
  • 2.3 Centripetal acceleration
  • 2.4 Period and frequency
• 3 Examples of uniform circular motion
  • 3.1 The movement of the Earth
  • 3.2 Particles on the edge of a disk
  • 3.3 Hubble Space Telescope
  • 3.4 Centrifuges
  • 3.5 Garden sprinklers
  • 3.6 Sports
• 4 Exercise resolved
• 5 References

Characteristics of uniform circular motion

The characteristics of uniform circular motion can be summarized as follows:

-The trajectory is a circumference, therefore it is a movement in the plane.

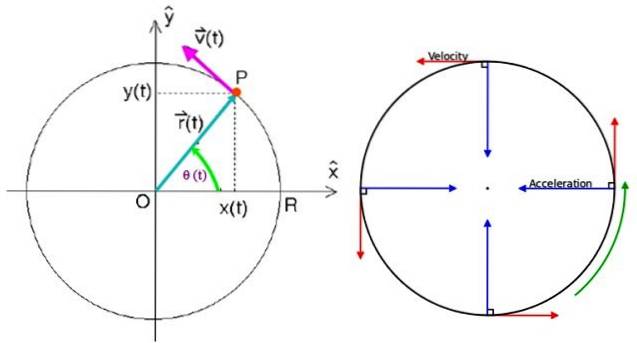
-The speed v is constant, but the speed v no, because it continually changes direction and direction to accommodate the turn of the mobile.

-The velocity vector v is always tangential to the circumference and perpendicular to the radial direction.

-The angular velocity ω is constant.

-Despite being uniform, there is an acceleration to explain these changes in the direction of velocity. This acceleration is the centripetal acceleration.

-Centripetal acceleration and velocity are perpendicular to each other.

-It is a periodic or repetitive movement, therefore the period and frequency magnitudes are defined for it.

Uniform circular motion formulas

In this scheme there is a particle P spinning counterclockwise with MCU, according to the direction and the sense of the velocity vector v drawn.

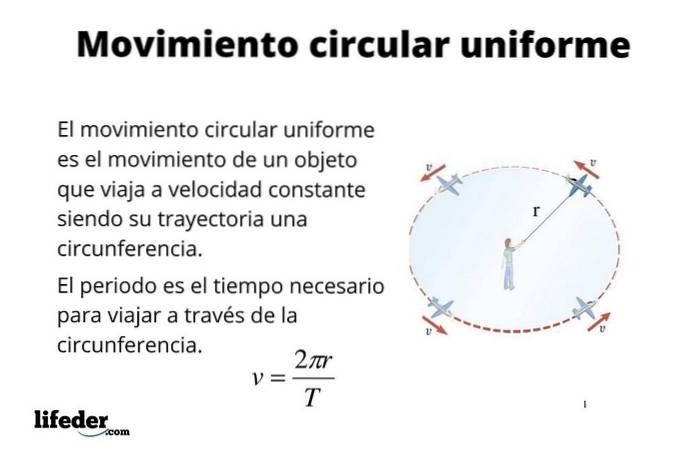
To specify the position vector it is necessary to have a reference point and the ideal point is the center of the circumference O that coincides with the center of the Cartesian coordinate system in the xy plane.

Position vector

It is denoted as r (t) and is directed from the origin to the point P where the particle is located. At a given instant t, in Cartesian coordinates, it is written as:

r (t) = x (t) i + and (t) j

Where i Y j are the unit vectors perpendicular in the directions x and Y respectively. From the graph it can be seen that the vector modulus r (t) always worth R, the radius of the circumference. If θ is the angle formed r with the horizontal axis, the position is also equal to:

r (t) = [Rcos θ(t)] i +[Rsen θ(t)] j

The angle it forms r (t) with the horizontal axis is a central angle and its value is:

θ = s / R

Where s is the arc of circumference traveled and R the radius. Said angle θ is a function of time, so it can be written θ = θ (t), call angular position.

Since the speed is constant, the particle describes equal angles in equal times and in analogy with the uniform rectilinear motion, it is written:

θ = θ (t) = θ_or + ωt

Here θ_or is the initial angle measured in radians with respect to the reference axis, it can be 0 or any value and ω is the angular speed.

Angular velocity and linear velocity

Angular velocity is the first derivative of angular position and is denoted as ω. Its value is constant for uniform circular motion, since equal angles are swept in equal times. In other words:

The units of linear speed in uniform circular motion are the same as for linear motion: m / s (in the SI International System), km / h, cm / s, and others..

Centripetal acceleration

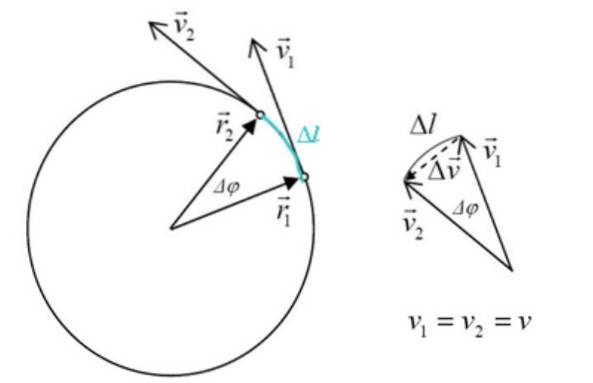
In the figure below there is a particle moving clockwise around the circumference with constant speed. This means that the velocity vector always has the same modulus, but it changes direction to accommodate the circumference.

Any change in velocity results in acceleration, which by definition is:

The triangle formed by v_two, v₁ and Δv is similar to the triangle of sides r_two, r₁ and Δl, where Δφ is the central angle. The magnitudes of r_two Y r₁ they are the same, so:

r_two = r₁ = r

Then, of both triangles we have these relations for the angle:

Δφ = Δr / r; Δφ = Δv / v

The bold type is not necessary, since the measure of the angle depends on the magnitudes of these vectors. Equating the previous expressions, it follows that:

Period and frequency

Since the circular motion is repetitive, the period is defined T the same as the time it takes for the mobile to make a complete turn. Since the length of the circumference of radius R is 2πR, the swept angle in radians going around the entire turn is 2π radians and it takes time T, the angular velocity is:

ω = 2π / T

T = 2π / ω

The period of uniform circular motion is measured in seconds in the International System.

For its part, the frequency F is the number of turns per unit of time and is the reciprocal or inverse of the period:

f = n / t = 1 / T

The unit of frequency in the International System is s^-1.

Examples of uniform circular motion

Many objects rotate to produce various effects: wheels, discs, and turbines. Once the operating speed is reached, the rotation is usually carried out at a constant speed. Circular motion is so common in everyday life that you hardly ever think about it, so here are some close examples that illustrate it very well:

The movement of the Earth

The Earth and the other planets of the Solar System move in elliptical trajectories of small eccentricity, except Mercury, which means that in first approximation, it can be assumed that their movement is uniform circular.

With this, you have a good idea of ​​the speed of translation around the Sun, since in the case of the Earth, the period of the movement is known: one year or 365 days..

Particles on the edge of a disk

The particles that rotate on the edge of an old record player or the blade of a fan, follow a uniform circular motion, once the device reaches its speed of reproduction.

Hubble space telescope

The Hubble Space Telescope circles the Earth at about 7550 m / s.

Centrifuges

The washing machines carry out a spinning process to squeeze the clothes, which consists of rotating the container drum at high speed. The dryers also rotate for a period of time in a uniform circular motion..

Centrifugation is also used in laboratories to separate compounds, for example, and thus separate their constituents by difference in densities. Whenever we talk about centrifugation, there is a circular motion that is uniform, at least for a time.

Garden watering cans

Many garden sprinklers rotate at a constant speed so that the ground is watered evenly..

sports

In the hammer throw for example, which is an Olympic discipline, the athlete forcefully spins a metal ball using a steel cable attached to the grip. The objective is to send the ball as far as possible, but without leaving a certain area.

Exercise resolved

A particle moves in a circle of radius 2m with a constant speed v = 8 m / s, counterclockwise. Initially the particle was in r = +2 j m. Calculate:

a) The angular velocity ω

b) Its angular position θ (t)

c) The period of movement

d) Centripetal acceleration.

e) Particle position after passing t = π / 4 s

Solution to

From the formula v = Rω it follows that:

ω = v / R = (8 m / s) / 2m = 4rad ∙ s^-1

Solution b

Taking the positive x axis as the reference axis, the particle is initially at 90º = π / 2 radians with respect to said axis, since the statement says that the initial position is +2 j m, that is, the particle is at y = 2m when the movement begins to follow.

θ = θ (t) = θ_or + ωt = π / 2 + 4t

Solution c

T = 2π / ω = 2π / 4 s = 0.5 π s

Solution d

a = v^two / R = (8 m / s)^two / 2 m = 32 m / s^two

Solution e

θ (t) = π / 2 + 4t → θ (π / 4) = π / 2 + 4 ∙ (π / 4) = 3π / 2 radians

This means that after that time, the particle is in the position y = -2m j. It makes sense because t = π / 4 s is half the period, therefore the particle traveled a 180º angle counterclockwise from its initial position and has to be just in the opposite position.

References

1. Figueroa, D. (2005). Series: Physics for Science and Engineering. Volume 1. Kinematics. Edited by Douglas Figueroa (USB).
2. Giambattista, A. 2010. Physics. 2nd. Ed. McGraw Hill.
3. Sears, Zemansky. 2016. University Physics with Modern Physics. 14th. Ed. Volume 1. Pearson.
4. Serway, R., Jewett, J. (2008). Physics for Science and Engineering. Volume 1. 7th. Ed. Cengage Learning.
5. Zapata, F. Circular Movement. Recovered from: francesphysics.blogspot.com.