
Angles in the circumference types, properties, solved exercises
Called circumference angles those in which some of its elements are or intersect at a given circumference. Among them are the following:
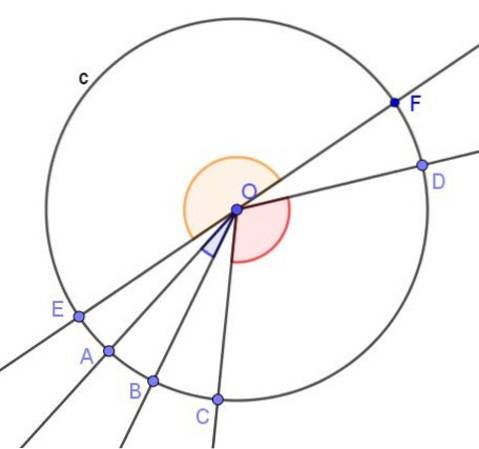
1.- The central angle, whose vertex is in the center of the circumference and its sides are secant to it, as we see in the following image:

2.- The inscribed angle, whose vertex is on the circumference and its sides are secant or tangent to the circumference.
3.- Outside angle, whose vertex is outside the circumference but its sides are secant or tangent to the circumference.
4.- The interior angle, with the vertex inside the circumference and its sides secant to it.
All these angles have certain relationships with each other and this leads us to important properties between the angles belonging to a given circle.
Article index
- 1 Properties
- 1.1 - Central angle
- 1.2 - Inscribed angle
- 1.3 - Exterior angle
- 1.4 - Interior angle
- 2 Solved exercises
- 2.1 - Exercise 1
- 2.2 - Exercise 2
- 3 References
Properties
- Central angle
The central angle is defined as that whose vertex is in the center of the circumference and its sides intersect the circumference.
The measure in radians of a central angle is the quotient between the subtending arc, that is, the arc of circumference between the sides of the angle, and the radius of the circumference.
If the circumference is unitary, that is, of radius 1, then the measure of the central angle is the length of the arc, which corresponds to the number of radians.
If you want the measure of the central angle in degrees, then multiply the measure in radians by the factor 180º / π.
Angle measuring instruments, such as the protractor and goniometer, always use a central angle and the length of the subtended arc.
They are calibrated in sexagesimal degrees, which means that whenever an angle is measured with them, in the background what is measured is the length of the arc subtended by the central angle.
Property
The measure of a central angle in radians is equal to the length of the subtending or intercepting arc divided by the length of the radius.
- Inscribed angle
The inscribed angle of a circle is one that has its vertex on the circumference and its rays are secant or tangent to it..
Its properties are:
Properties
-The inscribed angle is convex or plane.
-When an inscribed angle intersects the same arc as the central angle, the measure of the first angle will be half that of the second..

Figure 3 shows two angles ∠ABC and ∠AOC that intersect the same arc of circumference A⌒C.
If the measure of the inscribed angle is α, then the measure β of the central angle is twice the measure of the inscribed angle (β = 2 α) because both subtend the same arc of measure d.
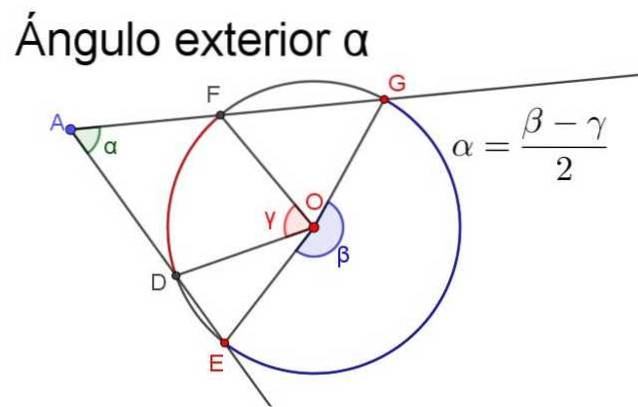
- Outside angle
It is the angle whose vertex is outside the circumference and each of its sides cuts the circumference at one or more points.
Property
-Its measure is equal to the half-difference (or difference divided by 2) of the central angles that intercept the same arcs.
To ensure that the measurement is positive, the semi-difference must always be that of the largest central angle minus the measure of the smallest central angle, as illustrated in the following figure.
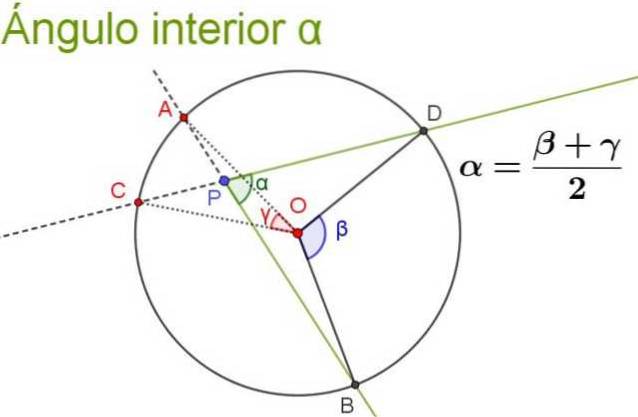
- Interior angle
The interior angle is the one whose vertex is inside the circumference and its sides intersect the circumference.
Property
Its measure is equal to the semi-sum of the central angle that subtends the same arc, plus the central angle that subtends the same arc as its extension angle (this is the interior angle formed by the rays complementary to those of the original interior angle).
The following figure illustrates and clarifies the property of the interior angle.
Solved exercises
- Exercise 1
Suppose an inscribed angle in which one of its sides passes through the center of the circle, as shown in Figure 6. The radius of the circle is OA = 3 cm and the arc d has a length of π / 2 cm. Determine the value of the angles α and β.
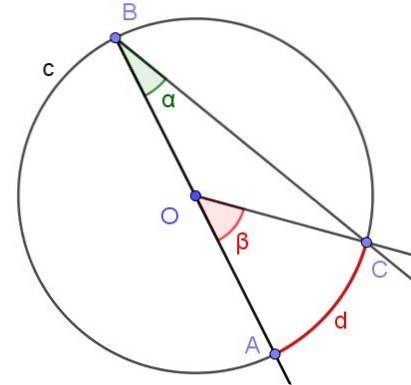
Solution
In this case, the isosceles triangle COB is formed, since [OC] = [OB]. In an isosceles triangle the angles adjacent to the base are equal, therefore ∠BCO = ∠ABC = α. On the other hand ∠COB = 180º - β. Considering the sum of the internal angles of the triangle COB, we have:
α + α + (180º - β) = 180º
From which it follows that 2 α = β, or what is equivalent α = β / 2, with which the property (3) of the previous section is confirmed, that the measure of the inscribed angle is half the central angle, when both angles subtend the same chord [AC].
Now we proceed to determine the numerical values: the angle β is central and its measure in radians is the quotient between the arc d and the radius r = OA, so its measure is:
β = d / r = (π / 2 cm) / (3 cm) = π / 6 rad = 30º.
On the other hand, it had already been stated that α = β / 2 = (π / 6 rad) / 2 = π / 12 rad = 15º.
- Exercise 2
In figure 7 the angles α1 and βtwo they have the same measure. Furthermore, the angle β1 measures 60º. Determine the angles β and α.
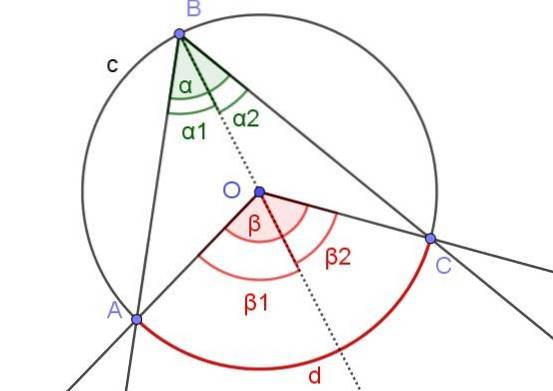
Solution
In this case we have an inscribed angle ∠ABC in which the center O of the circumference is within the angle.
Due to the property (3) we have αtwo = βtwo / 2 and α1 = β1 /two. What:
α = α1 + αtwo and β = β1 + βtwo
Therefore, it follows that:
α = α1 + αtwo = β1 / 2 + βtwo / 2 = (β1 + βtwo) / 2 = β / 2.
That is, according to the properties:
α = β / 2
Since we are told that β1 = 60º then:
α1 = β1 / 2 = 60º / 2 = 30º.
They also tell us that α1 = βtwo so it follows that:
βtwo = 30º.
The angle β results:
β1 + βtwo = 60º + 30º = 90º.
And since α = β / 2, then:
α = 90º / 2 = 45º.
In conclusion:
β = 90º and α = 45º.
References
- Baldor, A. 1973. Geometry and trigonometry. Central American Cultural Publishing House.
- E. A. 2003. Elements of geometry: with exercises and compass geometry. University of Medellin.
- Geometry 1st ESO. Angles on the circumference. Recovered from: edu.xunta.es.
- All Science. Solved problems of angles in the circumference. Recovered from: francesphysics.blogspot.com
- Wikipedia. Inscribed angle. Recovered from: es.wikipedia.com


Yet No Comments