The magnetic permeability is the physical quantity of the property of matter to generate its own magnetic field, when it is permeated by another external magnetic field.
Both fields: the external and the own, are superimposed giving a resulting field. The outer field, independent of the material, is called magnetic field strength H, while the superposition of the outer field plus the induced one in the material is the magnetic induction B.
When it comes to homogeneous and isotropic materials, the fields H Y B they are proportional. And the constant of proportionality (scalar and positive) is the magnetic permeability, denoted by the Greek letter μ:
B = μ H
In the SI International System the magnetic induction B is measured in Tesla (T), while the magnetic field strength H is measured in Ampere over meter (A / m).
Given the μ must guarantee dimensional homogeneity in the equation, the unit of μ in the SI system it is:
[μ] = (Tesla ⋅ meter) / Ampere = (T ⋅ m) / A
Article index
Let's see how magnetic fields are produced, whose absolute values we denote by B Y H, on a coil or solenoid. From there, the concept of magnetic permeability of the vacuum will be introduced..
The solenoid consists of a spirally wound conductor. Each turn of the spiral is called turn. If current is passed i by the solenoid, then you have an electromagnet that produces a magnetic field B.
Also, the value of magnetic induction B is greater, to the extent that the current i is increase. And also when the density of turns increases n (number N of turns between length d solenoid).
The other factor that affects the value of the magnetic field produced by a solenoid is the magnetic permeability μ of the material that is inside. Finally, the magnitude of said field is:
B = μ. i .n = μ. i. (N / a)
As stated in the previous section, the magnetic field intensity H it is:
H = i. (N / d)
That field of magnitude H, that only depends on the circulating current and the density of turns of the solenoid, "permeates" the material of magnetic permeability μ, causing it to become magnetized.
Then a total field of magnitude B, it does depend on the material that is inside the solenoid.
Similarly, if the material inside the solenoid is a vacuum, then the field H "permeates" the vacuum producing a resultant field B. The quotient between the field B in the void and the H produced by the solenoid defines the permeability of the vacuum, whose value is:
μor = 4π x 10-7 (T⋅m) / A
It turns out that the previous value was an exact definition until May 20, 2019. As of that date, a revision of the International System was made, which leads to μor be measured experimentally.
However, measurements made so far indicate that this value is extremely accurate..
Materials have a characteristic magnetic permeability. Now, it is possible to find the magnetic permeability with other units. For example, let's take the unit of inductance, which is henry (H):
1H = 1 (T ⋅ mtwo)/TO.
Comparing this unit with the one that was given at the beginning, it is seen that there is a similarity, although the difference is the square meter that Henry owns. For this reason, magnetic permeability is considered an inductance per unit length:
[μ] = H / m.
The magnetic permeability μ is closely related to another physical property of materials, called the magnetic susceptibility χ, which is defined as:
μ = μor (1 + χ)
In the above expression μor, is the magnetic permeability of vacuum.
The magnetic susceptibility χ is the proportionality between the external field H and the magnetization of the material M.
It is very common to express the magnetic permeability in relation to the permeability of the vacuum. It is known as relative permeability and it is nothing more than the quotient between the permeability of the material and that of the vacuum.
According to this definition, relative permeability is unitless. But it is a useful concept to classify materials.
For example, the materials are ferromagnetic, as long as its relative permeability is much greater than unity.
In the same way, substances paramagnetic have relative permeability just above 1.
And finally, diamagnetic materials have relative permeabilities just below unity. The reason is that they are magnetized in such a way that they produce a field that opposes the external magnetic field..
It is worth mentioning that ferromagnetic materials present a phenomenon known as "hysteresis", in which they keep memory of the fields previously applied. By virtue of this characteristic they can form a permanent magnet.
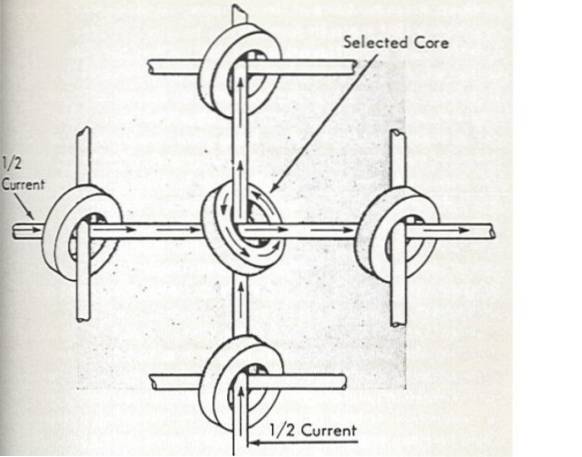
Due to the magnetic memory of ferromagnetic materials, the memories of early digital computers were small ferrite toroids traversed by conductors. There they saved, extracted or erased the content (1 or 0) of the memory.
Here are some materials, with their magnetic permeability in H / m and their relative permeability in parentheses:
Iron: 6.3 x 10-3 (5000)
Cobalt-iron: 2.3 x 10-two (18000)
Nickel-iron: 1.25 x 10-1 (100000)
Manganese-zinc: 2.5 x 10-two (20000)
Carbon steel: 1.26 x 10-4 (100)
Neodymium magnet: 1.32 x 10-5 (1.05)
Platinum: 1.26 x 10-6 1,0003
Aluminum: 1.26 x 10-6 1.00002
Air 1,256 x 10-6 (1.0000004)
Teflon 1,256 x 10-6 (1.00001)
Dry wood 1,256 x 10-6 (1.0000003)
Copper 1.27 x10-6 (0.999)
Pure water 1.26 x 10-6 (0.999992)
Superconductor: 0 (0)
Looking at the values in this table, it can be seen that there is a first group with magnetic permeability relative to that of vacuum with high values. These are the ferromagnetic materials, very suitable for the manufacture of electromagnets for the production of large magnetic fields.
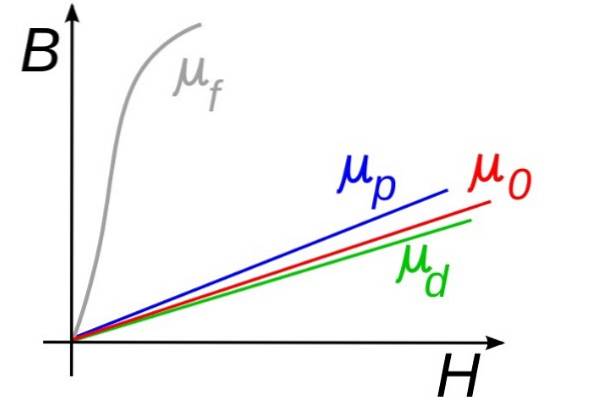
Then we have a second group of materials, with relative magnetic permeability just above 1. These are the paramagnetic materials..
Then you can see materials with relative magnetic permeability just below unity. These are diamagnetic materials such as pure water and copper.
Finally we have a superconductor. Superconductors have zero magnetic permeability because it completely excludes the magnetic field inside them. Superconductors are useless to be used in the core of an electromagnet.
However, superconducting electromagnets are often built, but the superconductor is used in the winding to establish very high electrical currents that produce high magnetic fields..
Yet No Comments