
Gage pressure explanation, formulas, equations, examples
The gauge pressure Pm is the one that is measured in relation to a reference pressure, which in most cases is chosen as the atmospheric pressure Patm at sea level. It is then a relative pressure, another term by which it is also known.
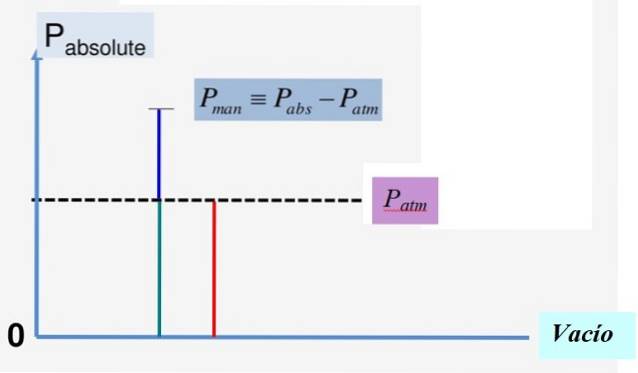
The other way in which pressure is usually measured is by comparing it with absolute vacuum, whose pressure is always zero. In such a case we speak of the absolute pressure, which we will denote as Pto.
The mathematical relationship between these three quantities is:
Pto = Patm + Pm
Therefore:
Pm = Pto - Patm
Figure 1 conveniently illustrates this relationship. Since the vacuum pressure is 0, the absolute pressure is always positive and the same happens with the atmospheric pressure Patm.
Gauge pressure is often used to denote pressures above atmospheric pressure, such as that found in tires or that at the bottom of the sea or a swimming pool, which is exerted by the weight of the water column. . In these cases Pm > 0, since Pto > Patm.
However, there are absolute pressures below Patm. In these cases Pm < 0 y recibe el nombre de Pressure from empty and not to be confused with pressure of vacuum already described, which is the absence of particles capable of exerting pressure.
Article index
- 1 Formulas and equations
- 1.1 Variation of pressure with depth
- 2 Examples
- 2.1 Pascal's principle
- 2.2 Stevin's hydrostatic paradox
- 3 Exercises
- 3.1 Exercise 1
- 3.2 Exercise 2
- 4 References
Formulas and equations
The pressure in a fluid -liquid or gas- is one of the most significant variables in its study. In a stationary fluid, the pressure is the same at all points at the same depth regardless of orientation, while the movement of fluids in the pipes is caused by changes in pressure..
Mean pressure is defined as the quotient between the force perpendicular to a surface F⊥ and the area of said surface A, which is expressed mathematically as follows:
P = F⊥ /TO
Pressure is a scalar quantity, the dimensions of which are force per unit area. The units of its measurement in the International System of Units (SI) are newton / mtwo, called Pascal and abbreviated as Pa, in honor of Blaise Pascal (1623-1662).
Multiples as kilo (103) Y mega (106) are frequently used, since the atmospheric pressure is usually in the range of 90,000 - 102,000 Pa, which is equal to: 90 - 102 kPa. Pressures of the order of megapascals are not uncommon, so it is important to familiarize yourself with the prefixes.
In Anglo-Saxon units, pressure is measured in pounds / foottwo, however, it is usually done in pounds / inchtwo or psi (pounds-force per square inch).
Variation of pressure with depth
The more we immerse ourselves in the water in a pool or in the sea, the more pressure we experience. On the contrary, as the height increases, the atmospheric pressure decreases.
The mean atmospheric pressure at sea level is set at 101,300 Pa or 101.3 kPa, while in the Mariana Trench in the Western Pacific - the deepest known depth - it is about 1000 times greater and at the top of Everest it is just 34 kPa.
It is clear that pressure and depth (or height) are related. To find out in the case of a fluid at rest (static equilibrium), a disk-shaped portion of fluid is considered, confined in a container, (see figure 2). The disc has cross sectional area TO, weight dW and height dy.
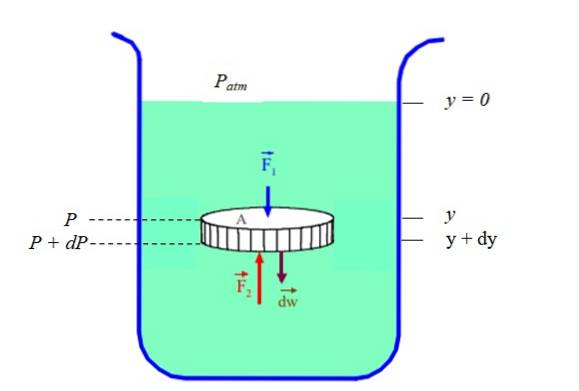
We will call P to the pressure that exists at depth "Y" Y P + dP to the pressure that exists at depth (y + dy). Since the density ρ of the fluid is the ratio of its mass dm and its volume dV, you have to:
ρ = dm / dV ⇒ dm = ρ.dV
Therefore the weight dW of the element is:
dW = g. dm = ρ.g.dV
And now Newton's second law applies:
Σ FY = Ftwo - F1 - dW = 0
(P + dP) .A - P.A - ρ.g.dV = 0
(P + dP) .A - P.A - ρ.g. A. dy = 0
dP = ρ.g.dy
Solution of the differential equation
Integrating both sides and considering that the density ρ, as well as gravity g are constants, the searched expression is found:
Ptwo - P1 = ΔP = ρ.g. (andtwo - Y1)
ΔP = ρ.g. ΔY
If in the previous expression you choose P1 as atmospheric pressure and Y1 as the surface of the liquid, then Ytwo it is located at a depth h Y ΔP = Ptwo - Patm is the gauge pressure as a function of depth:
Pm = ρ.g.h
In case of needing the absolute pressure value, simply add the atmospheric pressure to the previous result.
Examples
To measure the gauge pressure, a device called pressure gauge, which generally offer pressure differences. At the end the working principle of a U-tube manometer will be described, but now let's look at some important examples and consequences of the previously deduced equation.
Pascal's principle
The equation ΔP = ρ.g. (andtwo - Y1) can be written as P = Po + ρ.g.h, where P is the pressure at depth h, while Por is the pressure at the surface of the fluid, usually Patm.
Obviously every time it increases Po, increases P in the same quantity, as long as it is a fluid whose density is constant. It is precisely what was assumed when considering ρ constant and place it outside the integral solved in the previous section.
Pascal's principle states that any increase in the pressure of a confined fluid in equilibrium is transmitted without any variation to all points of said fluid. Through this property, it is possible to multiply the force F1 applied to the small plunger on the left, and obtain Ftwo on the right.
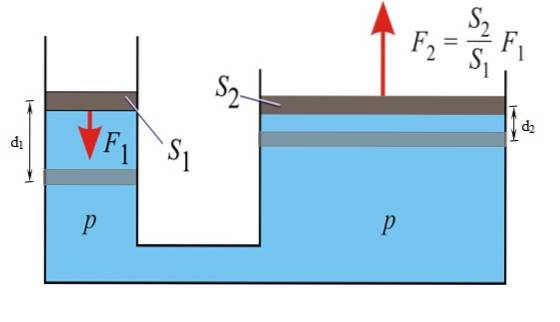
Car brakes work on this principle: a relatively small force is applied on the pedal, which is converted into a greater force on the brake cylinder at each wheel, thanks to the fluid used in the system..
Stevin's hydrostatic paradox
The hydrostatic paradox states that the force due to the pressure of a fluid at the bottom of a container can be equal to, greater or less than the weight of the fluid itself. But when you put the container on top of the scale, it will normally register the weight of the fluid (plus the container of course). How to explain this paradox?
We start from the fact that the pressure at the bottom of the container depends exclusively on the depth and is independent of the shape, as it was deduced in the previous section.

Let's look at a few different containers. Being communicated, when they fill with liquid they all reach the same height h. The highlights are at the same pressure, since they are at the same depth. However, the force due to pressure at each point may differ from the weight, (see example 1 below).
Training
Exercise 1
Compare the force exerted by the pressure on the bottom of each of the containers with the weight of the fluid, and explain the reasons for the differences, if any.
Container 1
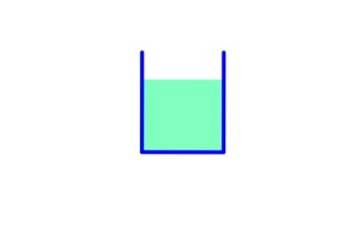
In this container the area of the base is A, therefore:
Fluid weight: mg = ρ.V.g = ρ . A .h. g
Pressure on the bottom: ρ. g. h
Force due to pressure: F = P.A = ρ. g. h. TO
Weight and force due to pressure are equal.
Container 2
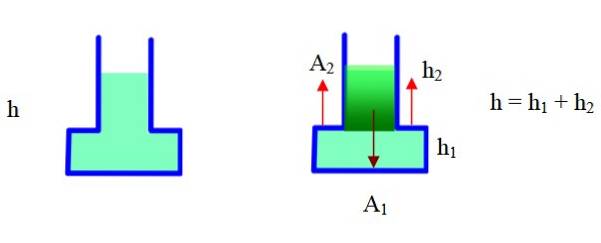
The container has a narrow part and a wide part. In the diagram on the right, it has been divided into two parts and geometry will be used to find the total volume. Area Atwo it is external to the container, htwo is the height of the narrow part, h1 is the height of the wide part (base).
The full volume is the volume of the base + the volume of the narrow part. With these data we have:
Fluid weight: m. g = ρ . g. V = ρ . g. [TO1 .h1+ (TO1 -TOtwo) .htwo] =
= ρ . g (A1.hatwohtwo) = ρ . g. TO1.h - ρ . g. TO.. htwo (Use has been made of h = h1 +htwo)
Pressure on the bottom: P = ρ. g. h
Force on the bottom due to pressure: F = P. A1 = ρ. g. h. TO1
Comparing the weight of the fluid with the force due to pressure shows that this is greater than the weight.
What happens is that the fluid also exerts force on the part of the step in the container (see the arrows in red in the figure) that are included in the above calculation. This upward force counteracts those exerted downward and the weight registered by the scale is the result of these. According to this, the magnitude of the weight is:
W = Force on the bottom - Force on the stepped part = ρ . g. TO1.h - ρ . g. TO.. htwo
Exercise 2
An open tube manometer is shown in the figure. It consists of a U-shaped tube, in which one end is at atmospheric pressure and the other is connected to S, the system whose pressure is to be measured..
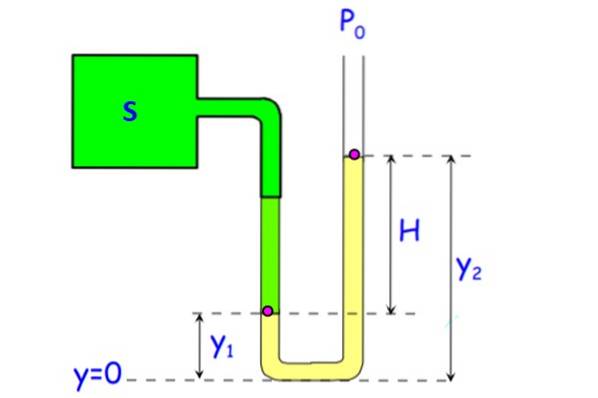
The liquid in the tube (shown in yellow in the figure) may be water, although mercury is preferably used to reduce the size of the device. (A difference of 1 atmosphere or 101.3 kPa requires a 10.3 meter water column, nothing portable).
It asks to find the gauge pressure Pm in system S, as a function of the height H of the liquid column.
Solution
The pressure at the bottom for both branches of the tube is the same, as they are at the same depth. Let PTO the pressure at point A, located at y1 And pB those of point B that is at the height andtwo. Since point B is at the interface of liquid and air, the pressure there is Por. In this branch of the manometer, the pressure at the bottom is:
Po + ρ.g.ytwo
For its part, the pressure at the bottom for the branch on the left is:
P + ρ.g.y1
Where P is the absolute pressure of the system and ρ is the density of the fluid. Equalizing both pressures:
Po + ρ.g.ytwo = P + ρ.g.y1
Clearing out P:
P = Po + ρ.g.ytwo - ρ.g.y1 = Po + ρ.g (andtwo - Y1) = Po + ρ.g. H
Therefore, the gauge pressure Pm is given by P - Por = ρ.g. H and to have its value, it is enough to measure the height to which the manometric liquid rises and multiply it by the value of g and the density of the fluid.
References
- Cimbala, C. 2006. Fluid Mechanics, Fundamentals and Applications. Mc. Graw Hill. 66-74.
- Figueroa, D. 2005. Series: Physics for Sciences and Engineering. Volume 4. Fluids and Thermodynamics. Edited by Douglas Figueroa (USB). 3-25.
- Mott, R. 2006. Fluid Mechanics. 4th. Edition. Pearson Education. 53-70.
- Shaugnessy, E. 2005. Introduction to Fluid Mechanics. Oxford University Press. 51 - 60.
- Stylianos, V. 2016. A simple explanation of the classic hydrostatic paradox. Recovered from: haimgaifman.files.wordpress.com



Yet No Comments