The Newton's first law, also known as the law of inertia, It was first proposed by Isaac Newton, an English physicist, mathematician, philosopher, theologian, inventor, and alchemist. This law establishes the following: “If an object is not subjected to any force, or if the forces acting on it cancel each other, then it will continue to move with constant speed in a straight line. "
In this statement the keyword is to be continue. If the premises of the law are fulfilled, then the object will continue with its movement as it had. Unless an unbalanced force appears and changes the state of motion.
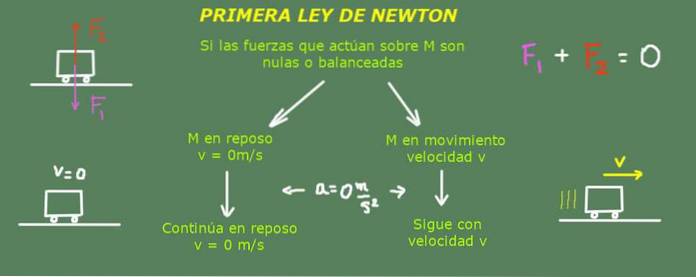
This means that if the object is at rest it will continue to rest, except if a force takes it out of that state. It also means that if an object is moving with a fixed speed in a straight direction, it will continue to move that way. It will only change when some external agent exerts a force on it and changes its speed..
Article index
Isaac Newton was born in Woolsthorpe Manor (United Kingdom) on January 4, 1643 and died in London in 1727.
The exact date that Sir Isaac Newton discovered his three laws of dynamics, including the first law, is not known with certainty. But it is known that it was long before the publication of the famous book Mathematical principles of natural philosophy, July 5, 1687.
The dictionary of the Royal Spanish Academy defines the word inertia as follows:
"Property of bodies to maintain their state of rest or movement if not by the action of a force".
This term is also used to affirm that any situation remains unchanged because no effort has been made to achieve it, therefore sometimes the word inertia has a connotation of routine or laziness..
Before Newton, the predominant ideas were those of the great Greek philosopher Aristotle, who stated that for an object to keep moving, a force must act on it. When the force ceases, then so will the movement. Not so, but even today many think so.
Galileo Galilei, a brilliant Italian astronomer and physicist who lived between 1564 and 1642, experimented and analyzed the movement of bodies.
One of Galileo's observations was that a body that slides on a smooth and polished surface with a certain initial impulse, takes longer to stop and has greater travel in a straight line, as the friction between the body and the surface is less.
It is evident that Galileo handled the idea of inertia, but he did not come to formulate a statement as precise as Newton.
Below we propose some simple experiments, which the reader can carry out and corroborate the results. The observations will also be analyzed according to the Aristotelian vision of movement and the Newtonian vision..
A box is propelled onto the floor and then the driving force is suspended. We observe that the box travels a small path until it stops.
Let us interpret the previous experiment and its result, in the framework of the theories before Newton and then according to the first law.
In the Aristotelian vision the explanation was very clear: the box stopped because the force that moved it was suspended.
In the Newtonian view, the box on the floor / ground cannot continue to move with the speed it had at the moment the force was suspended, because between the floor and the box there is an unbalanced force, which causes the speed to decrease until the box stops. It is about the frictional force.
In this experiment the premises of Newton's first law are not fulfilled, so the box stopped.
Again it is the box on the floor / ground. In this opportunity, the force on the box is maintained, in such a way that it compensates or balances the friction force. This happens when we get the box to follow with constant speed and in a straight direction.
This experiment does not contradict the Aristotelian view of motion: the box moves with constant speed because a force is exerted on it.
It also does not contradict Newton's approach, since all the forces acting on the box are balanced. Let's see:
Also in the vertical direction the forces are balanced, because the weight of the box, which is a force that points vertically downwards, is exactly compensated by the contact (or normal) force that the ground exerts on the box vertically upwards..
By the way, the weight of the box is due to the gravitational attraction of the Earth.
We continue with the box resting on the floor. In the vertical direction the forces are balanced, that is, the net vertical force is zero. It would certainly be very surprising if the box moved upwards. But in the horizontal direction there is friction force.
Now, for the premise of Newton's first law to be fulfilled, we need to reduce friction to its minimum expression. We can achieve this in a fairly approximate way if we look for a very smooth surface to which we spray silicone oil.
Since silicone oil reduces friction to almost zero, so when this box is thrown horizontally, it will maintain its speed and direction for a long time..
It is the same phenomenon that occurs with a skater on an ice rink, or with the ice hockey puck when they are propelled and released on their own..
In the situations described, in which the friction is reduced almost to zero, the resultant force is practically zero and the object maintains its speed, according to Newton's first law.
In the Aristotelian view this could not happen, because according to this naive theory, motion only occurs when there is a net force on the moving object..
Mass is a physical quantity that indicates the amount of matter that a body or object contains.
Mass then is an intrinsic property of matter. But matter is made up of atoms, which have mass. The mass of the atom is concentrated in the nucleus. It is the protons and neutrons of the nucleus that practically define the mass of the atom and matter..
Mass is generally measured in kilograms (kg), it is the basic unit of the International System of Units (SI).
The prototype or reference of kg is a platinum and iridium cylinder that is kept in the International Office of Weights and Measures in Sèvres in France, although in 2018 it was linked to the Planck constant and the new definition comes into effect as of May 20, 2019.
Well, it happens that inertia and mass are related. The greater the mass, the greater inertia an object has. It is much more difficult or costly in terms of energy to change the state of motion of a more massive object than a less massive one..
For example, it takes a lot more force and a lot more work to lift a one-ton (1000 kg) box from rest than a one kilogram (1 kg) box. That is why it is often said that the first has more inertia than the second.
Due to the relationship between inertia and mass, Newton realized that speed alone is not representative of the state of motion. That is why he defined a quantity known as momentum or momentum which is denoted by the letter p y is the product of the mass m for speed v:
p = m v
The bold in the p and in the v indicate that they are vector physical quantities, that is, they are quantities with magnitude, direction and sense.
Instead the mass m is a scalar quantity, to which a number is assigned that can be greater than or equal to zero, but never negative. So far no object of negative mass has been found in the known universe..
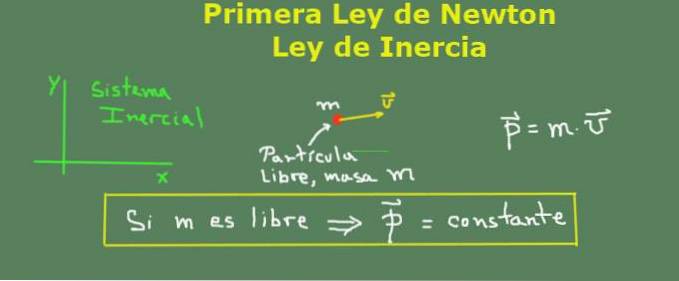
Newton took his imagination and abstraction to the extreme, defining the call free particle. A particle is a material point. That is, it is like a mathematical point but with mass:
A free particle is that particle that is so isolated, so far from another object in the universe that nothing can exert any interaction or force on it.
Later Newton went on to define the inertial reference systems, which will be those in which his three laws of motion apply. Here are the definitions according to these concepts:
Any coordinate system attached to a free particle, or that moves at constant speed with respect to the free particle, will be an inertial reference system.
If a particle is free, then it has a constant momentum with respect to an inertial reference frame.
A 160 gram hockey puck goes on the ice rink at 3 km / h. Find your momentum.
The mass of the disk in kilograms is: m = 0.160 kg.
Velocity in meters over second: v = (3 / 3.6) m / s = 0.8333 m / s
The momentum p is calculated as follows: p = m * v = 0.1333 kg * m / s,
Friction in the anterior disc is considered zero, so momentum is preserved as long as nothing alters the straight course of the disc. However, it is known that two forces act on the disc: the weight of the disc and the contact or normal force that the floor exerts on it..
Calculate the value of the normal force in newtons and its direction.
Since momentum is conserved, the resultant force on the hockey puck must be zero. The weight points vertically downwards and is equal to: P = m * g = 0.16 kg * 9.81 m / s²
The normal force must necessarily counteract the weight, so it must point vertically upwards and its magnitude will be 1.57 N.
Examples of Newton's law in real life.
Yet No Comments