A Square prism It is one whose surface is formed by two equal bases that are quadrilaterals and by four lateral faces that are parallelograms. They can be classified according to their angle of inclination, as well as the shape of their base.
A prism is an irregular geometric body that has flat faces and these enclose a finite volume, which is based on two polygons and lateral faces that are parallelograms. According to the number of sides of the polygons of the bases, the prisms can be: triangular, quadrangular, pentagonal, among others.
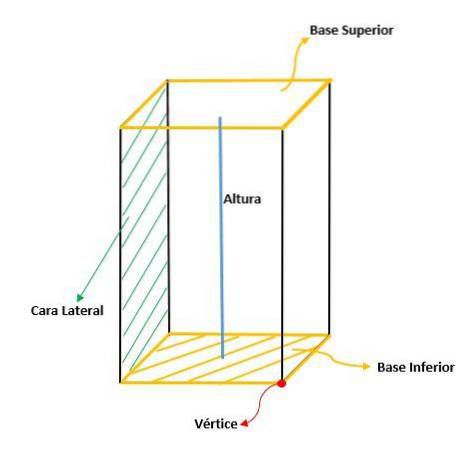
A prism with a quadrangular base is a polyhedral figure that has two equal and parallel bases, and four rectangles that are the lateral faces that join the corresponding sides of the two bases..
The quadrangular prism can be differentiated from the other types of prisms, because it has the following elements:
They are two polygons formed by four sides (quadrilateral), which are equal and parallel.
In total, this type of prism has six faces:
They are those points where three faces of the prism coincide, in this case there are 8 vertices in total.
They are segments where two faces of the prism meet and these are:
The number of edges of a polyhedron can also be calculated using Euler's theorem, if the number of vertices and faces are known; thus for the quadrangular prism it is calculated as follows:
Number of Edges = Number of faces + number of vertices - 2.
Number of Edges = 6 + 8 - 2.
Number of Edges = 12.
The height of the quadrangular prism is measured as the distance between its two bases.
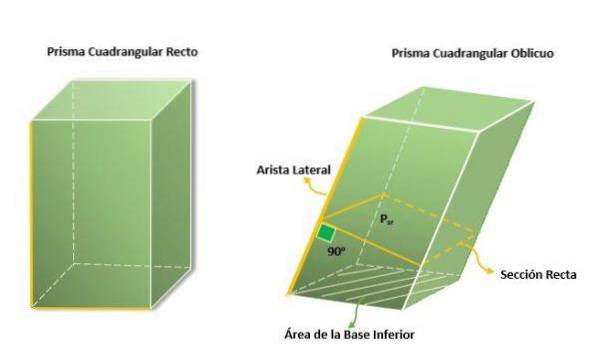
Quadrangular prisms can be classified according to their angle of inclination, which can be straight or oblique:
They have two equal and parallel faces, which are the bases of the prism, their lateral faces are formed by squares or rectangles, in this way their lateral edges are all equal and their length will be equal to the height of the prism.
The total area is determined by the area and perimeter of its base, by the height of the prism:
At = Aside + 2Abase.
This type of prism is characterized because its lateral faces form oblique dihedral angles with the bases, that is, its lateral faces are not perpendicular to the base, since they have a degree of inclination that can be less or more than 90or.
Its lateral faces are generally parallelograms with a rhombus or rhomboid shape, and may have one or more rectangular faces. Another characteristic of these prisms is that their height is different from the measurement of their lateral edges..
The area of an oblique quadrangular prism is calculated almost the same as the previous ones, adding the area of the bases with the lateral area; the only difference is the way its lateral area is calculated.
The area of the laterals is calculated with a lateral edge and the perimeter of the straight section of the prism, which is just where an angle of 90 is formedor with each of the sides.
TOtotal = 2 * Areabase + PerimeterMr * Edgeside
The volume of all types of prisms is calculated by multiplying the area of the base by the height:
V = Areabase * height = Ab * h.
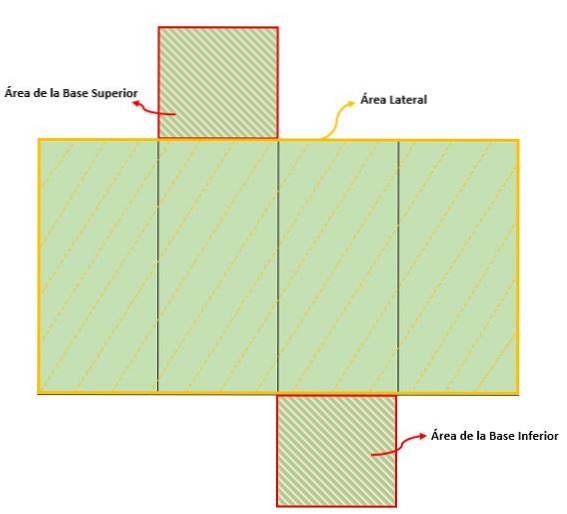
In the same way, quadrangular prisms can be classified according to the type of quadrilateral that the bases form (regular and irregular):
It is one that has two squares as a base, and its lateral faces are equal rectangles. Its axis is an ideal line that runs parallel to its faces and ends in the center of its two bases..
To determine the total area of a quadrangular prism, the area of its base and the lateral area must be calculated in such a way that:
At = Aside + 2Abase.
Where:
The lateral area corresponds to the area of a rectangle; namely:
TO side = Base * Height = B * h.
The area of the base corresponds to the area of a square:
TO base = 2 (Side * Side) = 2Ltwo
To determine the volume, multiply the area of the base by the height:
V = A base * Height = Ltwo* h
This type of prism is characterized because its bases are not square; can have bases consisting of unequal sides, and five cases are presented where:
to. The bases are rectangular
Its surface is formed by two rectangular bases and by four lateral faces that are also rectangles, all equal and parallel..
To determine its total area, each area of the six rectangles that form it, two bases, two small lateral faces and the two large lateral faces is calculated:
Area = 2 (a* b + a*h + b*h)
b. The bases are rhombuses:
Its surface is formed by two rhombus-shaped bases and by four rectangles that are the lateral faces, to calculate its total area, it must be determined:
Thus, the total area is: AT = Aside + 2Abase.
c. The bases are rhomboid
Its surface is formed by two rhomboid-shaped bases, and by four rectangles that are the lateral faces, its total area is given by:
d. The bases are trapezoids
Its surface is formed by two bases in the shape of trapezoids, and by four rectangles that are the lateral faces, its total area is given by:
and. The bases are trapezoids
Its surface is formed by two trapezoid-shaped bases, and by four rectangles that are the lateral faces, its total area is given by:
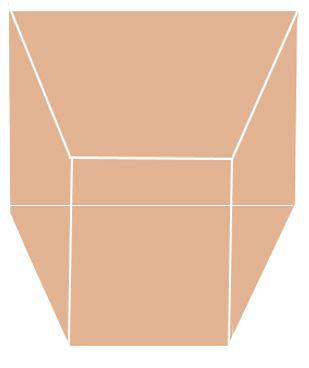
In summary, to determine the area of any regular quadrangular prism, it is only necessary to calculate the area of the quadrilateral that is the base, its perimeter and the height that the prism will have, in general, its formula would be:
Area Total = 2* Areabase + Perimeterbase * height = A = 2Ab + Pb * h.
To calculate the volume for these types of prisms, the same formula is used which is:
Volume = Areabase * height = Ab * h.
Yet No Comments