Polytropic process characteristics, applications and examples

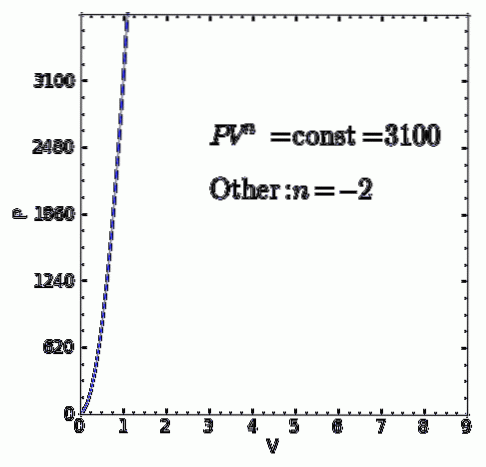
A polytropic process is a thermodynamic process that occurs when the relationship between the pressure P and the volume V given by P.Vⁿ it remains constant. The exponent n is a real number, generally between zero and infinity, but in some cases it can be negative.

The value of n receives the name of polytropy index and it is important to highlight that during a polytropic thermodynamic process said index must maintain a fixed value, otherwise the process will not be considered polytropic.

Article index

• 1 Characteristics of polytropic processes
• 2 Applications
  • 2.1 Work on polytropic processes for different values ​​of n
• 3 Examples of polytropic processes
  • 3.1 - Example 1
  • 3.2 - Example 2
• 4 References

Characteristics of polytropic processes

Some characteristic cases of polytropic processes are: 

- The isothermal process (at constant temperature T), in which the exponent is n = 1.

- An isobaric process (at constant pressure P), in this case n = 0.

- The isochoric process (at constant volume V), for which n = + ∞.

- Adiabatic processes (at constant S entropy), in which the exponent is n = γ, where γ is the adiabatic constant. This constant is the quotient between the heat capacity at constant pressure Cp divided by the heat capacity at constant volume Cv:

γ = Cp / Cv

- Any other thermodynamic process that is not one of the previous cases. but that complies P.Vⁿ = ctte with real and constant polytropic index n it will also be a polytropic process.

Applications

One of the main applications of the polytropic equation is to calculate the work done by a closed thermodynamic system, when it passes from an initial state to a final state in a quasi-static way, that is, following a succession of equilibrium states.

Work on polytropic processes for different values ​​of n

For n ≠ 1

The mechanical work W performed by a closed thermodynamic system is calculated by the expression:

W = ∫P.dV

Where P is the pressure and V the volume.

As in the case of a polytropic process, the relationship between pressure and volume is:

P.V ⁿ = constant = C

Solving for P from the previous expression to replace it in the work expression:

P = C /V ⁿ

We have the mechanical work done during a polytropic process, which begins in an initial state 1 and ends in the final state 2. All this appears in the following expression:

C = P₁ V₁ⁿ = P_two V_twoⁿ

By substituting the value of the constant in the work expression, we obtain:

W = (P_two V_two - P₁ V₁) / (1-n)

In the case that the working substance can be modeled as an ideal gas, we have the following equation of state:

P.V = m.R.T

Where m is the number of moles of the ideal gas and R is the universal gas constant.

For an ideal gas that follows a polytropic process with a polytropy index different from unity and that passes from a state with initial temperature T₁ to another state with temperature T_two we have that the work done is given by the following formula:

W = m R (T_two - T₁) / (1-n)

For n → ∞

According to the formula for the work obtained in the previous section, we have that the work of a polytropic process with n = ∞ is null, because the expression of the work is divided by infinity and therefore the result tends to zero.

Another way to arrive at this result is from the relation P₁ V₁ⁿ = P_two V_twoⁿ, which can be rewritten as follows:

(P₁/ P_two) = (V_two/ V1)ⁿ

Taking the nth root in each member, we obtain:

(V_two/ V1) = (P₁/ P_two)^{(1 / n)}

In the case that n → ∞, we have (V_two/ V1) = 1, which means that:

V_two = V₁

That is, the volume does not change in a polytropic process with n → ∞. Therefore, the volume differential dV in the integral of mechanical work is 0. These types of polytropic processes are also known as processes isochoric, or constant volume processes.

For n = 1

Again we have the expression the expression for work:

W = ∫P dV

In the case of a polytropic process with n = 1, the relationship between pressure and volume is:

P V = constant = C

By solving for P from the previous expression and substituting, we have the work done to go from initial state 1 to final state 2:

Namely:

W = C ln (V_two/ V₁).

As the initial and final states are well determined, so will the ctte. Namely:

C = P₁ V₁ = P_two V_two

Finally, we have the following useful expressions to find the mechanical work of a polytropic closed system in which n = 1.

W = P₁ V₁ ln (V_two/ V₁) = P_two V_two ln (V_two/ V₁)

If the working substance consists of m moles of ideal gas, then the ideal gas equation of state can be applied: P V = m.R.T.

In this case, as P.V₁ = ctte, we have that a polytropic process with n = 1 is a process at constant temperature T (isothermal), so that the following expressions for the work can be obtained:

W = m R T₁ ln (V_two/ V₁) = m R T_two ln (V_two/ V₁)

Examples of polytropic processes

- Example 1

Suppose a cylinder with a movable piston filled with one kilogram of air. Initially the air occupies a volume V₁= 0.2 m³ at pressure P₁= 400 kPa. A polytropic process is followed with n = γ = 1.4, whose final state has pressure P_two = 100 kPa. Determine the work done by the air on the piston.

Solution

When the polytropy index equals the adiabatic constant, there is a process in which the working substance (air) does not exchange heat with the environment, and therefore the entropy does not change.

For air, a diatomic ideal gas, we have:

γ = Cp / Cv, with Cp = (7/2) R and Cv = (5/2) R

Then:

γ = 7/5 = 1.4

Using the expression of the polytropic process, the final volume of the air can be determined:

V_two = [(P_two V₁^1.4) / P_two]^{(1 / 1.4)} = 0.54 m³.

Now we have the conditions to apply the formula for work done in a polytropic process for n ≠ 1 obtained above:

W = (P_two V_two - P1 V1) / (1-n)

Substituting the appropriate values ​​we have:

W = (100 kPa 0.54 m³ - 400 kPa 0.2 m³) / (1 - 1.4) = 65.4 kJ

- Example 2

Assume the same cylinder as in Example 1, with a movable piston filled with one kilogram of air. Initially the air occupies a volume V1 = 0.2 m³ at a pressure P1 = 400 kPa. But unlike the previous case, the air expands isothermally to reach a final pressure P2 = 100 kPa. Determine the work done by the air on the piston.

Solution

As seen previously, isothermal processes are polytropic processes with index n = 1, so it is true that:

P1 V1 = P2 V2

In this way, the final volume can be easily peeled off to obtain:

V2 = 0.8 m³

Then, using the work expression obtained previously for the case n = 1, we have that the work done by the air on the piston in this process is:

W = P1 V1 ln (V2 / V1) = 400000 Pa × 0.2 m³ ln (0.8 / 0.2) = 110.9 kJ.  

References

1. Bauer, W. 2011. Physics for Engineering and Sciences. Volume 1. Mc Graw Hill.
2. Cengel, Y. 2012. Thermodynamics. 7th Edition. Mcgraw hill.
3. Figueroa, D. (2005). Series: Physics for Science and Engineering. Volume 4. Fluids and Thermodynamics. Edited by Douglas Figueroa (USB).
4. López, C. The First Law of Thermodynamics. Recovered from: culturacientifica.com.
5. Knight, R. 2017. Physics for Scientists and Engineering: a Strategy Approach. Pearson.
6. Serway, R., Vulle, C. 2011. Fundamentals of Physics. 9th Ed. Cengage Learning.
7. Sevilla University. Thermal Machines. Recovered from: laplace.us.es.
8. Wikiwand. Polytropic process. Recovered from: wikiwand.com.