
Lock Property of Algebra Proof, Examples
The lock property of algebra is a phenomenon that relates two elements of a set with an operation, where the necessary condition is that, after the 2 elements are processed under said operation, the result also belongs to the initial set.
For example, if we take the even numbers as a set and a sum as an operation, we obtain a lock of that set with respect to the sum. This is because the sum of 2 even numbers will always yield another even number, thus fulfilling the lock condition.

Article index
- 1 Features
- 2 Number algebra
- 3 Demo
- 3.1 Sum
- 3.2 Multiplication
- 4 Special cases in R
- 4.1 Division
- 4.2 Filing
- 4.3 Logarithm
- 5 Examples
- 5.1 Sum in N
- 5.2 Subtraction in N
- 6 Proposed exercises
- 7 References
Characteristics
There are many properties that determine algebraic spaces or bodies, such as structures or rings. However, the lock property is one of the best known within basic algebra.
Not all applications of these properties are based on numerical elements or phenomena. Many everyday examples can be worked from a pure algebraic-theoretical approach.
An example can be the citizens of a country who assume a legal relationship of any kind, such as a commercial partnership or marriage among others. After this operation or management is carried out, they remain citizens of the country. In this way citizenship and management operations with respect to two citizens represent a lock.
Numerical algebra
With regard to numbers, there are many aspects that have been the subject of study in different currents of mathematics and algebra. A large number of axioms and theorems have emerged from these studies that serve as the theoretical basis for contemporary research and work..
If we work with the numerical sets we can establish another valid definition for the lock property. A set A is said to be the lock of another set B if A is the smallest set that contains all the sets and operations that B houses..
Demonstration
The lock proof is applied for elements and operations present in the set of real numbers R.
Let A and B be two numbers that belong to the set R, define the lock of these elements for each operation contained in R.
Sum
- Sum: ∀ A ˄ B ∈ R → A + B = C ∈ R
This is the algebraic way of saying that For all A and B that belong to the real numbers, we have that the sum of A plus B is equal to C, which also belongs to the real numbers.
It is easy to check if this proposition is true; it is enough to carry out the sum between any real number and check if the result also belongs to the real numbers.
3 + 2 = 5 ∈ R
-2 + (-7) = -9 ∈ R
-3 + 1/3 = -8/3 ∈ R
5/2 + (-2/3) = 11/6 ∈ R
It is observed that the lock condition is fulfilled for the real numbers and the sum. In this way it can be concluded: The sum of real numbers is an algebraic lock.
Multiplication
- Multiplication: ∀ A ˄ B ∈ R → A. B = C ∈ R
For all A and B that belong to the reals, we have that the multiplication of A by B is equal to C, which also belongs to the reals.
When verifying with the same elements of the previous example, the following results are observed.
3 x 2 = 6 ∈ R
-2 x (-7) = 14 ∈ R
-3 x 1/3 = -1 ∈ R
5/2 x (-2/3) = -5/3 ∈ R
This is enough evidence to conclude that: Multiplication of real numbers is an algebraic lock.
This definition can be extended to all operations on real numbers, although we will find certain exceptions.

Special cases in R
Division
As the first special case, the division is observed, where the following exception is observed:
∀ A ˄ B ∈ R → A / B ∉ R ↔ B = 0
For all A and B that belong to R we have that A among B does not belong to the reals if and only if B is equal to zero.
This case refers to the restriction of not being able to divide by zero. Since zero belongs to the real numbers, then it is concluded that: lThe division is not a lock on the reals.
Filing
There are also potentiation operations, more specifically those of radicalization, where exceptions are presented for radical powers of even index:
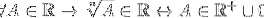
For all A that belongs to the reals, the nth root of A belongs to the reals, if and only if A belongs to the positive reals joined to a set whose only element is zero.
In this way it is denoted that the even roots only apply to the positive reals and it is concluded that the potentiation is not a lock in R.
Logarithm
In a homologous way, it can be seen for the logarithmic function, which is not defined for values less than or equal to zero. To check if the logarithm is a lock of R, proceed as follows:

For all A that belongs to the reals, the logarithm of A belongs to the reals, if and only if A belongs to the positive reals.
By excluding negative values and zero that also belong to R, it can be stated that:
The logarithm is not a lock of the real numbers.
Examples
Check the lock for addition and subtraction of natural numbers:
Sum in N
The first thing is to check the lock condition for different elements of the given set, where if it is observed that any element breaks with the condition, the existence of a lock can be automatically denied.
This property is true for all possible values of A and B, as observed in the following operations:
1 + 3 = 4 ∈ N
5 + 7 = 12 ∈ N
1000 + 10000 = 11000 ∈ N
There are no natural values that break the lock condition, so it is concluded:
The sum is a lock in N.
Subtract in N
Natural elements capable of breaking the condition are sought; A - B belongs to the natives.
Operating it is easy to find pairs of natural elements that do not meet the lock condition. For example:
7 - 10 = -3 ∉ a N
In this way we can conclude that:
Subtraction is not a lock of the set of natural numbers.
Proposed exercises
1-Show if the lock property is fulfilled for the set of rational numbers Q, for the operations addition, subtraction, multiplication and division.
2-Explain if the set of real numbers is a lock of the set of integers.
3-Determine which numerical set can be lock of the real numbers.
4-Prove the lock property for the set of imaginary numbers, with respect to addition, subtraction, multiplication and division.
References
- Panorama of pure mathematics: the Bourbakist choice. Jean Dieudonné. Reverte, 1987.
- Algebraic number theory. Alejandro J. Díaz Barriga, Ana Irene Ramírez, Francisco Tomás. National Autonomous University of Mexico, 1975.
- Linear Algebra and its Applications. Sandra Ibeth Ochoa García, Eduardo Gutiérrez González.
- Algebraic structures V: body theory. Hector A. Merklen. Organization of American States, General Secretariat, 1979.
- Introduction to commutative algebra. Michael Francis Atiyah, I. G. MacDonald. Reverte, 1973.



Yet No Comments