
What is yield effort and how to obtain it?
The Yielding effort It is defined as the effort necessary for an object to begin to permanently deform, that is, to undergo plastic deformation without breaking or fracturing.
As this limit can be a bit imprecise for some materials and the precision of the equipment used is a weight factor, in engineering it has been determined that the yield stress in metals such as structural steel is that which produces 0.2% permanent deformation in the object.

Knowing the value of the yield stress is important to know if the material is appropriate for the use that you want to give to the parts manufactured with it. When a part has deformed beyond the elastic limit, it may not be able to perform its intended function correctly and must be replaced.
To obtain this value, tests are usually carried out on samples made with the material (test tubes or specimens), which are subjected to various stresses or loads, while the elongation or stretch they experience with each one is measured. These tests are known as tensile tests.
To perform a tensile test, start by applying a force from zero and gradually increase the value until the sample breaks..
Article index
- 1 Stress-strain curves
- 1.1 Elastic zone
- 1.2 Elastic-plastic zone
- 1.3 Plastic zone and fracture
- 2 How to get the yield effort?
- 2.1 Yield stress from the stress-strain curve
- 2.2 Important details to keep in mind
- 3 References
Stress-strain curves
The data pairs obtained by the tensile test are plotted by placing the load on the vertical axis and the deformation on the horizontal axis. The result is a graph like the one shown below (figure 2), called the stress-strain curve for the material.
From it many important mechanical properties are determined. Each material has its own stress-strain curve. For example, one of the most studied is structural steel, also called mild or low carbon steel. It is a material widely used in construction.
The stress-strain curve has distinctive areas in which the material has a certain behavior according to the applied load. Their exact shape can vary considerably, but nevertheless they have some characteristics in common which are described below..
For what follows see figure 2, which corresponds in very general terms to structural steel.
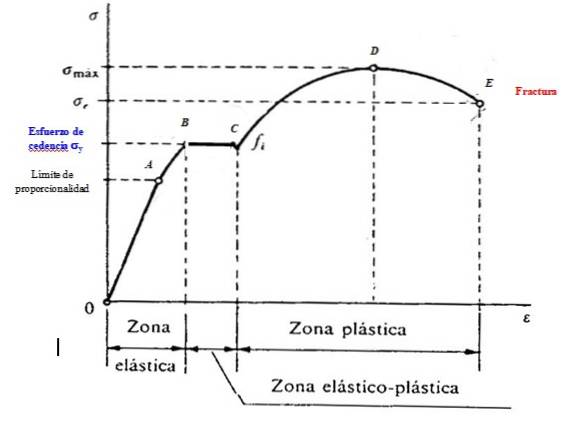
Elastic zone
The area from O to A is the elastic area, where Hooke's Law is valid, in which the stress and strain are proportional. In this zone the material is fully recovered after application of the stress. Point A is known as the limit of proportionality.
In some materials, the curve that goes from O to A is not a straight line, but nevertheless they are still elastic. The important thing is that they regain their original shape when charging ceases..
Elastic-plastic zone
Next we have the region from A to B, in which the deformation increases more rapidly with the effort, leaving both of them not proportional. The slope of the curve decreases and at B it becomes horizontal.
From point B, the material no longer recovers its original shape and the value of the stress at that point is considered to be that of the yield stress.
The zone from B to C is called the yield zone or creep of the material. There the deformation continues even though the load is not increasing. It could even decrease, that is why it is said that the material in this condition is perfectly plastic.
Plastic zone and fracture
In the region from C to D, strain hardening occurs, in which the material presents alterations in its structure at the molecular and atomic level, which require greater efforts to achieve deformations..
For this reason, the curve experiences a growth that ends when reaching the maximum stress σmax.
From D to E there is still deformation possible but with less load. A kind of thinning forms in the sample (test tube) called stricture, which finally leads to the fracture being observed at point E. However, already at point D the material can be considered to be broken.
How to get the yield effort?
The elastic limit Land of a material is the maximum effort it can withstand without losing its elasticity. It is calculated by the quotient between the magnitude of the maximum force Fm and the cross-sectional area of sample A.
Land = Fm / TO
The units of the elastic limit in the International System are N / mtwo o Pa (Pascals) since it is an effort. The elastic limit and the proportionality limit at point A are very close values.
But as said at the beginning, it may not be easy to determine them. The yield stress obtained through the stress-strain curve is the practical approximation to the elastic limit used in engineering.
Yield stress from the stress-strain curve
To obtain it, a line is drawn parallel to the line that corresponds to the elastic zone (the one that obeys Hooke's law) but displaced approximately 0.2% on the horizontal scale or 0.002 inch per inch of deformation..
This line extends to intersect the curve at a point whose vertical coordinate is the desired yield stress value, denoted as σY, as shown in figure 3. This curve belongs to another ductile material: aluminum.
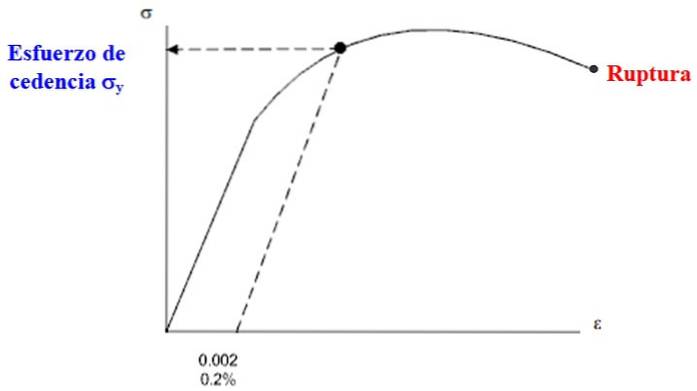
Two ductile materials like steel and aluminum have different stress-strain curves. Aluminum, for example, does not have the approximately horizontal section of steel seen in the preceding section..
Other materials considered fragile, such as glass, do not go through the stages described above. Rupture occurs long before appreciable deformations occur.
Important details to keep in mind
- The forces considered in principle do not take into account the change that undoubtedly occurs in the cross-sectional area of the specimen. This induces a small error that is corrected by graphing the actual efforts, those that take into account the reduction of the area as the deformation of the specimen increases.
- The temperatures considered are normal. Some materials are ductile at low temperatures, while other brittle materials behave as ductile at higher temperatures..
References
- Beer, F. 2010. Mechanics of materials. McGraw Hill. 5th. Edition. 47-57.
- Engineers Edge. Yield Strength. Recovered from: engineersedge.com.
- Creep stress. Recovered from: instron.com.ar
- Valera Negrete, J. 2005. Notes on General Physics. UNAM. 101-103.
- Wikipedia. Creep. Recovered from: Wikipedia.com



Yet No Comments