What is the modulus of shear, stiffness or shear? (Exercises solved)

The cutting module describes the response of a material to the application of a shear stress that deforms it. Other frequently used names for shear modulus are shear modulus, shear modulus, transverse elasticity, or tangential elasticity..

When the efforts are small, the deformations are proportional to them, according to Hooke's law, the shear modulus being the constant of proportionality. Therefore:

Shear modulus = Shear stress / Strain

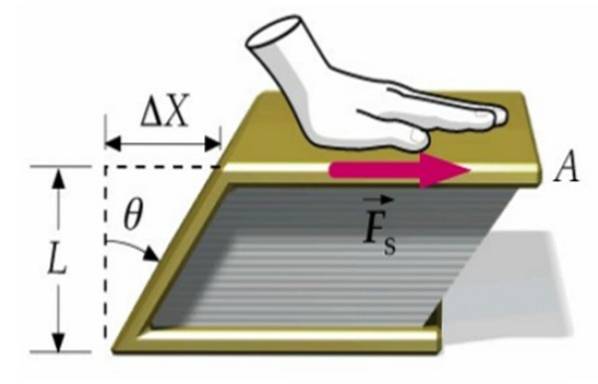
Suppose that one force is applied to the cover of a book, the other being fixed on the surface of the table. In this way, the book as a whole does not move, but deforms when the upper cover moves with respect to the lower one by the amount Δx.

The book goes from having a rectangular cross section to a parallelogram-shaped section, as we see in the image above.

Be:

τ = F / A

The shear stress or tension, being F the magnitude of the applied force and TO the area on which it acts.

The deformation caused is given by the quotient:

δ = Δx / L

Therefore the shear modulus, which we will denote as G, is:

And since Δx / L has no dimensions, the units of G are the same as the shear stress, which is the ratio of force to area..

In the International System of Units, these units are Newton / square meter or pascal, abbreviated Pa. And in Anglo-Saxon units it is pound / square inch, abbreviated psi.

Article index

• 1 Cutting module for various materials
  • 1.1 Experimental measurement of shear modulus
  • 1.2 How to find G?
• 2 Exercises with solution
  • 2.1 - Exercise 1
  • 2.2 - Exercise 2
• 3 References

Cutting module for various materials

Under the action of shear forces such as those described, the objects offer a resistance similar to that of the book, in which the inner layers slide. This type of deformation can only occur in solid bodies, which have sufficient rigidity to resist being deformed..

On the other hand, liquids do not offer this kind of resistance, but they can undergo volume deformations.

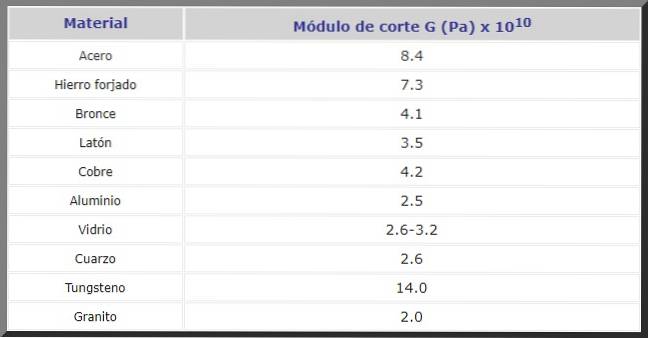
Below is the G in Pa cutting module for various materials frequently used in construction and in the manufacture of machinery and spare parts of all kinds:

Experimental measurement of shear modulus

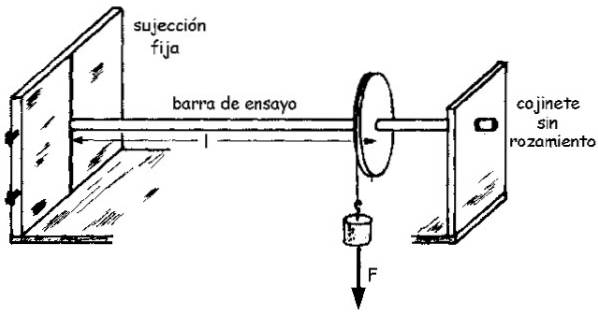
To find the value of the shear modulus, samples of each material must be tested and their response to the application of a shear stress examined..

The sample is a rod made of the material, with radius R and length L known, which is fixed at one end, while the other is connected to the shaft of a pulley free to rotate.

A rope is attached to the pulley, at the free end of which a weight is hung that exerts a force F on the rod through the rope. And this force in turn produces a moment M on the rod, which then rotates a small angle θ.

A diagram of the assembly can be seen in the following figure:

The magnitude of the moment M, which we denote as M (no bold) is related to the rotated angle θ through the shear modulus G according to the following equation (deduced by a simple integral):

Since the magnitude of the moment is equal to the product of the module of the force F and the radius of the pulley R_p:

M = F.R_p

And strength is the weight that hangs W, then:

M = W.R_p

Substituting in the equation for the magnitude of the moment:

There is the relationship between weight and angle:

How to find G?

This relationship between the variables W Y θ is linear, so the different angles produced by hanging different weights are measured.

The weight and angle pairs are plotted on graph paper, the best line passing through the experimental points is fitted and the slope is calculated m of said line.

Exercises with solution

- Exercise 1

A rod 2.5 meters long and radius 4.5 mm is fixed at one end. The other is connected to a 75 cm radius pulley that has a hanging weight W of 1.3 kg. The angle turned is 9.5º.

With these data, it is requested to calculate the shear modulus G of the rod.

Solution

From the equation:

G is cleared:

And the values ​​given in the statement are substituted, taking care to express all the data in the International System of Units SI:

R = 4.5 mm = 4.5 x 10 ^-3 m

R_p = 75 cm = 0.075

To go from kilograms (which are actually kilograms - force) to newton multiply by 9.8:

W = 1.3 kg-force = 1.3 x 9.8 N = 12.74 N

And finally, the degrees must be in radians:

9.5 º = 9.5 x2π / 360 radians = 0.1658 radians.

With all this you have:

= 2,237 x 10¹⁰ Pa

- Exercise 2

A cube made of gel has a side of 30 cm. One of its faces is fixed, but at the same time, a parallel force of 1 N is applied to the opposite face, which thanks to it moves 1 cm (see the example of the book in figure 1).

It is requested to calculate with these data:

a) The magnitude of the shear stress

b) Strain δ

c) The value of the shear modulus

Solution to

The magnitude of the shear stress is:

τ = F / A

With:

A = side^two = (30 x 10^-two cm)^two = 0.09 m^two

Therefore:

τ = 1 N / 0.09 m^two = 11.1 Pa

Solution b

Strain is none other than the value of δ, given by:

δ = Δx / L

The displacement of the face subjected to the force is 1 cm, then:

δ = 1/30 = 0.0333

Solution c

The shear modulus is the quotient between the shear stress and the strain:

G = Shear stress / Strain

Therefore:

G = 11.1 Pa / 0.033 = 336.4 Pa

References

1. Beer, F. 2010. Mechanics of materials. McGraw Hill. 5th. Edition.
2. Franco García, A. Solid Rigid. Shear modulus measurement. Recovered from: sc.ehu.es.
3. Giancoli, D. 2006. Physics: Principles with Applications. 6th. Ed prentice hall.
4. Resnick, R. (1999). Physical. Vol. 1. 3rd Ed. In Spanish. Compañía Editorial Continental S.A. by C.V.
5. University of Valladolid. Department of Physics of Condensed Matter. Selection of problems. Recovered from: www4.uva.es.