What is an icosagon? Characteristics and Properties

A icosagon or isodecagon is a polygon that has 20 sides. A polygon is a plane figure formed by a finite sequence of line segments (more than two) which enclose a region of the plane.

Each line segment is called a side and the intersection of each pair of sides is called a vertex. According to the number of sides, the polygons are given particular names.

The most common are the triangle, quadrilateral, pentagon and hexagon, which have 3, 4, 5 and 6 sides respectively, but can be built with the desired number of sides..

Characteristics of an icosagon

Below are some characteristics of polygons and their application in an icosagon.

1- Classification

An icosagon, being a polygon, can be classified as regular and irregular, where the word regular refers to the fact that all the sides have the same length and the interior angles all measure the same; otherwise it is said that the icosagon (polygon) is irregular.

2- Isodecagon

The regular icosagon is also called a regular isodecagon, because to obtain a regular icosagon what you must do is bisect (divide into two equal parts) each side of a regular decagon (10-sided polygon).

3- Perimeter

To calculate the perimeter "P" of a regular polygon, the number of sides is multiplied by the length of each side.

In the particular case of an icosagon, the perimeter is equal to 20xL, where "L" is the length of each side.

For example, if you have a regular icosagon with a 3cm side, its perimeter is equal to 20x3cm = 60cm.

It is clear that, if the isogon is irregular, the above formula cannot be applied.

In this case, the 20 sides must be added separately to obtain the perimeter, that is, the perimeter “P” is equal to ∑Li, with i = 1,2,…, 20.

4- Diagonals

The number of diagonals "D" that a polygon has is equal to n (n-3) / 2, where n represents the number of sides.

In the case of an icosagon, it follows that it has D = 20x (17) / 2 = 170 diagonals.

5- Sum of the internal angles

There is a formula that helps to calculate the sum of the internal angles of a regular polygon, which can be applied to a regular icosagon.

The formula consists of subtracting 2 from the number of sides of the polygon and then multiplying this number by 180º.

The way this formula is obtained is that we can divide a polygon with n sides into n-2 triangles, and using the fact that the sum of the internal angles of a triangle is 180º we obtain the formula.

The following image illustrates the formula for a regular enegon (9-sided polygon).

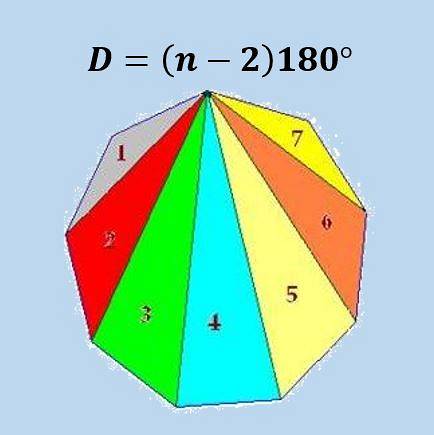
Using the previous formula it is obtained that the sum of the internal angles of any icosagon is 18 × 180º = 3240º or 18π.

6- Area

To calculate the area of ​​a regular polygon it is very useful to know the concept of apothem. The apothem is a perpendicular line that goes from the center of the regular polygon to the midpoint of any of its sides.

Once the length of the apothem is known, the area of ​​a regular polygon is A = Pxa / 2, where "P" represents the perimeter and "a" the apothem..

In the case of a regular icosagon, its area is A = 20xLxa / 2 = 10xLxa, where “L” is the length of each side and “a” is its apothem.

On the other hand, if you have an irregular polygon with n sides, to calculate its area, divide the polygon into n-2 known triangles, then calculate the area of ​​each of these n-2 triangles and finally add all these areas.

The method described above is known as triangulation of a polygon.

References

1. C., E. Á. (2003). Elements of geometry: with numerous exercises and compass geometry. University of Medellin.
2. Campos, F. J., Cerecedo, F. J., & Cerecedo, F. J. (2014). Math 2. Patria Editorial Group.
3. Freed, K. (2007). Discover Polygons. Benchmark Education Company.
4. Hendrik, v. M. (2013). Generalized Polygons. Birkhäuser.
5. IGER. (s.f.). Mathematics First Semester Tacaná. IGER.
6. jrgeometry. (2014). Polygons. Lulu Press, Inc.
7. Mathivet, V. (2017). Artificial Intelligence for Developers: Concepts and Implementation in Java. ENI editions.
8. Miller, Heeren, & Hornsby. (2006). Mathematics: Reasoning And Applications 10 / e (Tenth Edition ed.). Pearson Education.
9. Oroz, R. (1999). Dictionary of the Spanish language. University Publishing House.
10. Patiño, M. d. (2006). Mathematics 5. Editorial Progreso.
11. Rubió, M. d.-M. (1997). The forms of urban growth. Univ. Politèc. from Catalunya.