
What is a Perigonal Angle? 5 Examples to Understand
The perigonal angles, also known as complete and integers, are those in which the sides of their angle coincide, equivalent to four right angles that measure 360º.
In plane geometry, an angle is a figure made up of two line segments, called rays, which meet at one of their ends: the vertex.

To distinguish between these lines, they are marked with the letters A and B, with A being the origin point (the ray that remains fixed) and B the extreme point (the ray that moves to form the opening).
The gap between the line segments that are part of an angle is measured in degrees (°) and is called the amplitude; This measure allows the angles to be classified into four types:
1 - Acute angles: they have an amplitude less than 90 °.
2 - Right angles: they have an amplitude of exactly 90 °.
3 - obtuse angles: have an amplitude greater than 90 ° and less than 180 °.
4 - Concave angles:
-Flat angles: have a width of 180 °.
-Reflex angles: have an amplitude greater than 180 ° but less than 360 °.
-Perigonal angles: they have an amplitude of 360 °. They are also called complete angles and whole angles..
In this sense, it is observed that the perigonal angle, when measuring 360 °, forms a circumference. Likewise, the perigonal angles can result from the sum of other angles of smaller amplitude, for example, four right angles form one perigonal.
The perigonal angle is a concave angle
Concave angles are those that have an amplitude between 180 ° and 360 °.
In this sense, there are three types of concave angles: flat (180 °), reflections (greater than 180 ° but less than 360 °) and perigonal (360 °).
The perigonal angle and the circumferences
The perigonal angle is equal to the width of a circle, that is, 2 radians (360 °). This means that the perigonal angles are formed when one of the rays makes a complete turn with respect to the other ray, positioning itself on it. For example, clock hands form perigonal angles.
In this sense, like the circumferences, the perigonal angles can be subdivided into quadrants (1/4 of the circumference), radians (1/2 of the circumference), among other divisions.

The perigonal angle: end and origin
As explained above, at every angle there is a ray that is called the extreme and another that is called the origin. AND
n the perigonal angles, the endpoint and the origin are in the same position, since the endpoint has made one complete turn with respect to the origin.
The perigonal angle and consecutive angles
Consecutive angles are those that share a side in common, that is, the ray of one is the same ray of the other.
Perigonal angles can be made up of a series of consecutive angles that, added together, complete the 360 °.
For example:
- Two 180 ° angles = one perigonal angle
- Three 120 ° angles = one perigonal angle
- Four 90 ° angles = one perigonal angle
- Five 72 ° angles = one perigonal angle
- Six 60 ° angles = one perigonal angle
And so on.
It should be noted that the angles that make up the perigonal do not necessarily have to have the same amplitude.
For example, a series of four consecutive angles having amplitudes of 30 °, 80 °, 100 °, and 150 ° (360 ° in total) is also a perigonal angle..
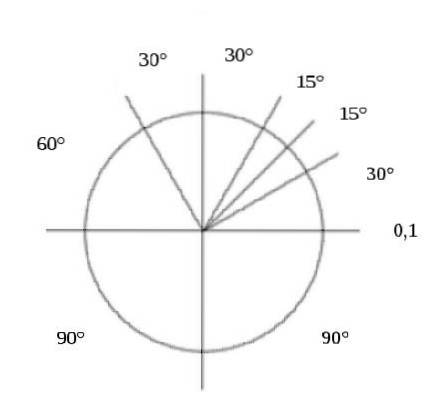
Examples of perigonal angles
In our day to day, we are surrounded by objects that measure 360 ° and, therefore, can be perigonal angles. Here are some examples of these:
1- The wheels
The wheels of bicycles, automobiles, and other vehicles are examples of perigonal angles. In addition, the rims of bicycles and automobiles present dividing lines that could be understood as series of consecutive angles.
2- A clock with hands
Analog clocks feature hands that rotate to mark the time. Let us take into account a second hand and a minute hand when they are positioned on the number 12 of the clock, indicating the first second of a minute..
The seconds move at a rate of 6 ° per second, which means that, after the minute, the hand will have traveled 360 °.
In this example, the minute hand and the second hand are the two rays of an angle: the minute hand has remained in position, while the second hand has made one complete turn, creating a perigonal angle.
For its part, a minute hand takes 60 minutes to complete a perigonal angle with respect to the hand that marks the hour..
3- Steering wheels and rudders
The steering wheels of automobiles and the rudders of ships are also examples of perigonal angles..
As with a bicycle wheel, some steering wheels and rudders feature segments that could serve as consecutive angles..

4- The blades of a fan or a windmill
These systems generally have three or four blades. If there are three blades, they are three consecutive angles of 120 °; if it has four, they will be consecutive 90 ° angles.
5- The reels of a video camera
The reels of a video camera have three radial divisions of 120 ° each. The sums of the angles created by these divisions give rise to a perigonal angle.
References
- Turn (geometry). Retrieved on June 2, 2017, from en.wikipedia.org.
- Perigon. Retrieved on June 2, 2017, from memidex.com.
- Perigon. Retrieved on June 2, 2017, from thefreedictionary.com.
- Angle. Retrieved on June 2, 2017, from en.wikipedia.org.
- Full angle. Retrieved on June 2, 2017, from mathworld.wolfram.com.
- Angles. Retrieved on June 2, 2017, from mathteacher.com.au.
- Perigon. Retrieved on June 2, 2017, from merriam-webster.com.
- Perigon. Retrieved on June 2, 2017, from dictionary.com.

Yet No Comments