
What are degenerate orbitals?
The degenerate orbitals they are all those who are at the same energy level. According to this definition, they must have the same principal quantum number n. Thus, the 2s and 2p orbitals are degenerate, since they belong to energy level 2. However, it is known that their angular and radial wave functions are different.
As the values of n, the electrons begin to occupy other energy sublevels, such as the d and f orbitals. Each of these orbitals have their own characteristics, which at first glance can be seen in their angular shapes; These are the spherical (s), dumbbell (p), cloverleaf (d) and globular (f) figures.
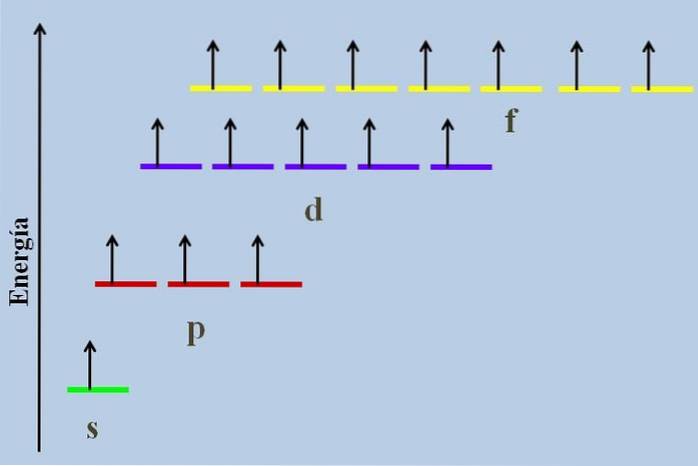
Between them, there is an energetic difference, even though they belong to the same level n.
For example, the image above shows an energy scheme with the orbitals occupied by unpaired electrons (an abnormal case). It can be seen that of all the most stable (the one with the lowest energy) is the ns (1s, 2s, ...) orbital, while the nf is the most unstable (the one with the highest energy).
Article index
- 1 Degenerate orbitals of an isolated atom
- 1.1 Orbitals p
- 1.2 Orbitals d
- 1.3 Orbitals f
- 2 Degenerate Hybrid Orbitals
- 3 References
Degenerate orbitals of an isolated atom
Degenerate orbitals, with the same value of n, they are on the same line in an energy scheme. For this reason the three red stripes that symbolize the p orbitals are located on the same line; as do the purple and yellow stripes in the same way.
The diagram in the image violates Hund's rule: the higher-energy orbitals are filled with electrons without pairing them first in the lower-energy ones. As the electrons mate, the orbital loses energy, and exerts a greater electrostatic repulsion on the unpaired electrons of the other orbitals..
However, such effects are not considered in many energy diagrams. If so, and obeying Hund's rule without completely filling the d orbitals, it would be seen that they stop being degenerate.
As previously mentioned, each orbital has its own characteristics. An isolated atom, with its electronic configuration, has its electrons arranged in the precise number of orbitals to accommodate them. Only those equal in energy can be considered degenerate.
Orbitals p
The three red stripes for the degenerate p orbitals in the image indicate that both px, pY And pz they have the same energy. There is an unpaired electron in each, described by four quantum numbers (n, l, ml Y ms), while the first three describe the orbitals.
The only difference between them is denoted by the magnetic moment ml, which draws the path of px on an x axis, pY on the y-axis, and pz on the z axis. All three are the same, but differ only in their spatial orientations. For this reason they are always drawn aligned in energy, that is, degenerate.
Since they are the same, an isolated nitrogen atom (with 1s configurationtwo2stwo2 P3) must keep its three p orbitals degenerate. However, the energy scenario changes abruptly if one considers an N atom within a molecule or chemical compound..
Why? Because although px, pY And pz they are equal in energy, this can vary in each one of them if they have different chemical environments; that is, if they bind to different atoms.
Orbitals d
There are five purple stripes that denote the d orbitals. In an isolated atom, even if they have paired electrons, these five orbitals are considered degenerate. However, unlike the p orbitals, this time there is a marked difference in their angular shapes.
Therefore, its electrons travel directions in space that vary from one d orbital to another. This causes, according to crystal field theory, that a minimum disturbance causes a energy splitting of the orbitals; that is, the five purple stripes separate, leaving an energy gap between them:
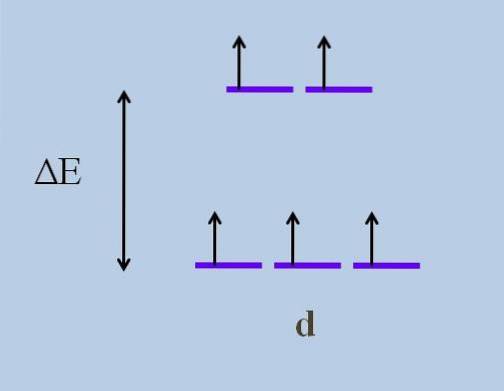
What are the top orbitals and what are the bottom orbitals? Those above are symbolized as andg, and those below t2 g. Note how initially all the purple stripes were aligned, and now a set of two orbitals was formed andg more energetic than the other set of three orbitals t2 g.
This theory allows us to explain the d-d transitions, to which many of the colors observed in the compounds of the transition metals (Cr, Mn, Fe, etc.) are attributed. And what is the cause of this electronic disturbance? To the coordination interactions of the metal center with other molecules called ligands.
Orbitals f
And with the f orbitals, the felt yellow stripes, the situation becomes even more complicated. Their spatial directions vary a lot between them, and the display of their links becomes too complex.
In fact, the f orbitals are considered to have such an internal shell that they do not “participate appreciably” in bond formation..
When the isolated atom with f orbitals surrounds itself with other atoms, interactions begin and unfolding occurs (the loss of degeneracy):

Note that now the yellow stripes form three sets: t1g, t2 g Y to1g, and that they are no longer degenerate.
Degenerate Hybrid Orbitals
It has been seen that the orbitals can unfold and lose degeneration. However, while this explains electronic transitions, it pales in elucidating how and why there are different molecular geometries. This is where hybrid orbitals come in..
What is its main characteristics? That they are degenerate. Thus, they arise from the mixture of characters of orbitals s, p, d and f, to originate degenerate hybrids.
For example, three p orbitals mix with one s to give four sp orbitals3. All sp orbitals3 are degenerate, and therefore have the same energy.
If additionally two d orbitals are mixed with the four sp3, you will get six sp orbitals3dtwo.
And how do they explain molecular geometries? As there are six, with equal energies, they must therefore be oriented symmetrically in space to generate equal chemical environments (for example, in an MF compound6).
When they do, a coordination octahedron is formed, which is equal to an octahedral geometry around a center (M).
However, geometries are often distorted, which means that even hybrid orbitals are not really completely degenerate. Therefore, by way of conclusion, degenerate orbitals only exist in isolated atoms or in highly symmetric environments..
References
- Chemicool Dictionary. (2017). Definition of Degenerate. Recovered from: chemicool.com
- SparkNotes LLC. (2018). Atoms and Atomic Orbitals. Recovered from: sparknotes.com
- Pure chemistry. (s.f.). Electronic configuration. Recovered from: es-puraquimica.weebly.com
- Whitten, Davis, Peck & Stanley. (2008). Chemistry. (8th ed.). CENGAGE Learning.
- Moreno R. Esparza. (2009). Coordination chemistry course: Fields and orbitals. [PDF]. Recovered from: depa.fquim.unam.mx
- Shiver & Atkins. (2008). Inorganic chemistry. (Fourth edition). Mc Graw Hill.



Yet No Comments