The mathematical relationship is the link that exists between the elements of a subset with respect to the product of two sets. A function involves the mathematical operation to determine the value of a dependent variable based on the value of an independent variable. Every function is a relation but not every relation is a function.
| Relationship | Function | |
|---|---|---|
| Definition | Subset of ordered pairs that correspond to the Cartesian product of two sets. | Mathematical operation to be performed with the variable x to get the variable Y. |
| Notation | x R Y; x it is related to Y. | Y= ƒ (x); Y is a function of x. |
| Characteristics |
|
|
| Examples |
|
|
It is called the binary relation of a set A in a set B or the relation between elements of A and B to every subset C of the Cartesian product A x B.
That is, if set A is made up of elements 1, 2 and 3, and set B is made up of elements 4 and 5, the Cartesian product of A x B will be the ordered pairs:
A x B = (1,4), (2,4), (3, 4), (1,5), (2,5), (3,5).
The subset C = (2,4), (3,5) will be a relation of A and B since it is composed of the ordered pairs (2,4) and (3, 5), the result of the Cartesian product of A x B.
"Let A and B be any two non-empty sets, let A x B be the product set of both, that is: A x B is formed by the ordered pairs (x, y) such that x is the element of A and Y it is of B. If in A x B any subset C is defined, a binary relation in A and B is automatically determined as follows:
x R Y if and only if (x, y) ∈ C
(the notation x R Y Means "x it's related to Y").
We will call set A starting set and we will call set B arrival set.
The relationship domain are the elements that make up the starting set, while the ratio range are the elements of the arrival set.
Set TO from x elements of men in a population and B is the set of Y elements of women from the same population. A relationship is established when "x is married to Y".
When we talk about a mathematical function of a set A in a set B we refer to a rule or mechanism that relates the elements of set A with an element of set B.
"Sean x Y Y two real variables, it is then said that y is a function of x yes to each value I take x corresponds to a value of Y."
The independent variable is x while Y is the dependent variable or function:
y = ƒ (x)
The set in which the x it is called domain of the function (original) and the variation of Y function range (picture).
The set of pairs (x, Y) such that Y= ƒ (x) is called function graph; if they are represented in Cartesian axes, a family of points is obtained called function graph.
In mathematics we get many examples of functions. Here are examples of flagship functions.
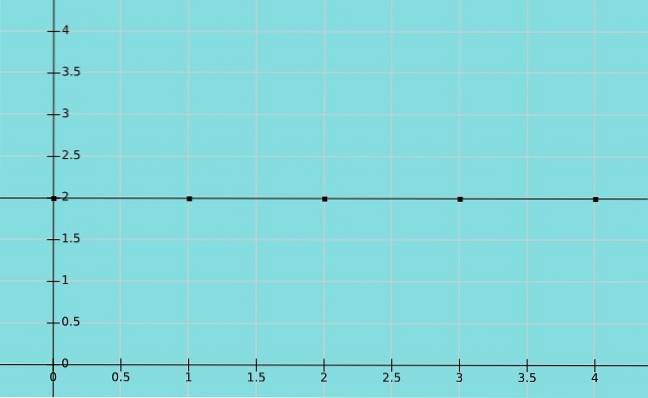
A function is called constant if the element of set B that corresponds to set A is the same. In this case, all the values of x correspond to the same value of y. Thus, the domain is the real numbers while the range is a constant value.
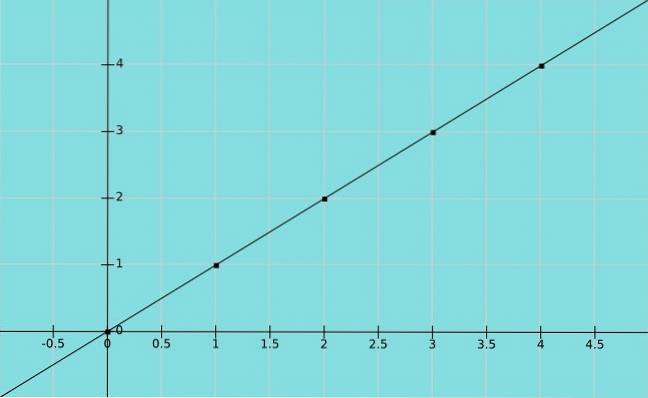
Let's suppose x is a variable and that Y takes the same value as x. We then have an identity function y = x, where the pairsx, y) in the graph are (1,1), (2,2), (3,3) and so on.
A polynomial function fulfills the form y = anxn+ton-1+xn-1+… + Atwoxtwo+to1x + a0. The graph above shows the function ƒ (x) = xtwo+x-2.
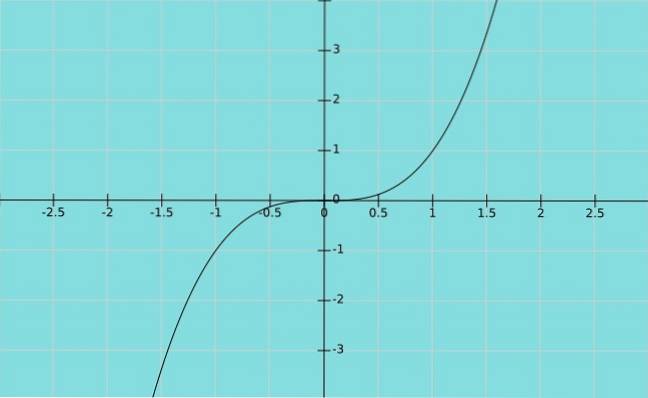
Now suppose that the dependent variable Y equals the independent variable x raised to the cube. We have the function y = x3, whose graph is shown below:
Yet No Comments