The molecular kinetic theory It is one that seeks to explain the experimental observations of gases from a microscopic perspective. That is to say, it tries to associate the nature and behavior of the gaseous particles, with the physical characteristics of the gas as a fluid; explain the macroscopic from the microscopic.
Gases have always been of interest to scientists because of their properties. They occupy the entire volume of the container in which they are found, being able to be completely compressed without their content opposing the least resistance; and if the temperature increases, the container begins to expand, and may even crack.
Many of these properties and behaviors are summarized in the ideal gas laws. However, they consider the gas as a whole and not as a collection of millions of particles scattered in space; Furthermore, it does not provide, based on pressure, volume and temperature data, further information regarding how these particles move.
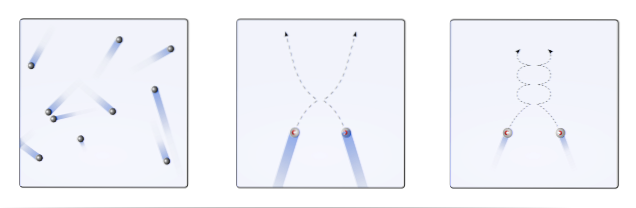
It is thus then that the molecular kinetic theory (TCM), proposes to visualize them as mobile spheres (upper image). These spheres collide with each other and the walls arbitrarily, and maintain a linear trajectory. However, when the temperature decreases and the pressure increases, the trajectory of the spheres becomes curved..
A gas, according to TCM, should behave like the spheres in the first frame of the image. But by cooling and increasing the pressure on them, their behavior is far from ideal. They are then real gases, close to undergoing liquefaction and thus pass to the liquid phase.
Under these conditions, the interactions between the spheres become more important, to the point that their speeds momentarily slow down. The closer they are to liquefaction, the more curved their trajectories become (inset on the right), and their collisions are less energetic.
Article index
The idea of these spheres, better called atoms, had already been considered by the Roman philosopher Lucretius; not for gases, but for solid, static objects. On the other hand, in 1738 Daniel Bernoulli applied the atomic vision to gases and liquids by imagining them as disordered spheres moving in all directions..
His work, however, violated the laws of physics at the time; a body could not move eternally, so it was impossible to think that a set of atoms and molecules would collide with each other without losing their energy; that is, the existence of elastic collisions was not possible.
A century later, other authors reinforced TCM with a model where the gaseous particles moved in only one direction. Rudolf Clausius, however, compiled his results and put together a more complete TCM model with which he sought to explain the ideal gas laws demonstrated by Boyle, Charles, Dalton, and Avogadro..
In 1859, James Clerk Maxwell stated that gaseous particles exhibit a range of velocities at a given temperature, and that a set of them can be considered by means of an average molecular velocity..
Then in 1871 Ludwig Boltzmann connected existing ideas with entropy, and how gas thermodynamically always tends to occupy as much space as possible homogeneously and spontaneously.
To consider the gas from its particles, a model is necessary in which certain postulates or assumptions are fulfilled; postulates that logically must be able to predict and explain (as accurately as possible) macroscopic and experimental observations. That said, the TCM postulates are mentioned and described.
In a container filled with gaseous particles, these disperse and move away from each other in all corners. If for a moment they could all be brought together at a specific point in the container, without liquefaction, it would be observed that they only occupy a negligible portion of the volume of the container..
It means that the container, even if it contains millions of gaseous particles, is actually more empty than full (volume-void ratio much less than 1); therefore, if its barriers allow it, it and the gas inside it can be compressed abruptly; since at the end of the day the particles are very small, as is their volume.
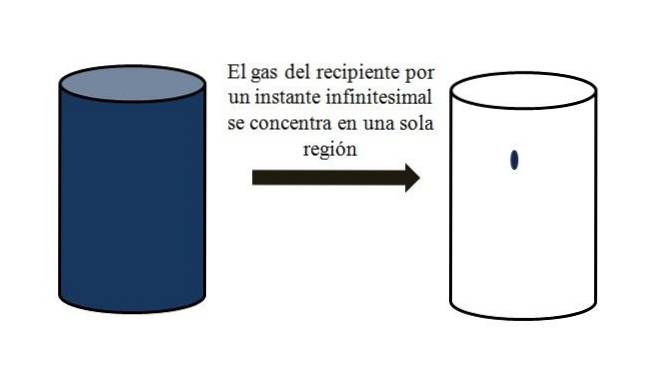
The upper image accurately illustrates the above, using a bluish-colored gas.
The gaseous particles inside the container collide with each other without enough time for their interactions to gain strength; even less when what mainly surrounds them is molecular vacuum. An immediate consequence of this is that their linear paths allow them to completely encompass the volume of the container..
If this were not the case, a container with a "bizarre" and "labyrinthine" shape would have humid regions as a result of gas condensation; instead, the particles travel around the container with full freedom, without the force of their interactions stopping them.
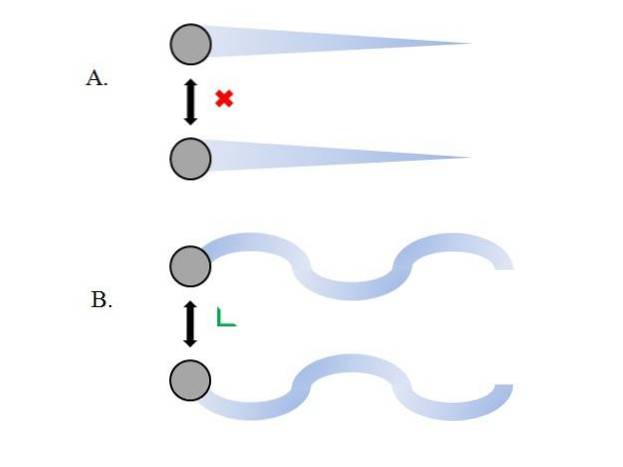
The linear trajectories of the upper image (A.) demonstrate this postulate; while if the trajectories are curved (B.), it shows that there are interactions that cannot be ignored between the particles.
From the first two postulates, the fact that the gas particles never stop moving also converges. Once blurred in the container, they collide with each other and with its walls, with a force and speed directly proportional to the absolute temperature; this force is, pressure.
If the gaseous particles stopped moving for an instant, "tongues of smoke" would be witnessed inside the container, emerging from nowhere, with enough time to arrange themselves in a vacuum and give random shapes.
If only elastic collisions between the gaseous particles and the walls of the container predominate inside the container, condensation of the gas will never occur (as long as the physical conditions do not change); or what is the same as saying that they never rest and are always colliding.
This is because in elastic collisions there is no net loss of kinetic energy; a particle collides with the wall and bounces at the same speed. If a particle when colliding slows down, the other accelerates, without producing heat or sound that dissipates the kinetic energy of either of them.
The movement of the particles is random and chaotic, so that they do not all have the same speed; just as it happens, for example, on a highway or in a crowd. Some are more energetic and travel faster, while others are slow, waiting for a collision to speed them up.
To describe its speed, it is then necessary to calculate an average; and with this, the average kinetic energy of the gaseous particles or molecules is obtained in turn. As the kinetic energy of all the particles is constantly changing, the average allows a better control of the data and can be worked with greater reliability..
Average molecular kinetic energy (ECmp) in a container changes with temperature. The higher the temperature, the higher the energy will be. Because it is an average, there may be particles or gases that have higher or lower energy than this value; some faster and some slower, respectively.
Mathematically it can be shown that ECmp it depends exclusively on the temperature. This means that no matter what the gas is, its mass or molecular structure, its ECmp it will be the same at a temperature T and will only vary if it increases or decreases. Of all the postulates, this is perhaps the most relevant..
And what about the average molecular speed? Unlike ECmp, molecular mass does influence speed. The heavier the gaseous particle or molecule, it is natural to expect it to move more slowly..
Here are some brief examples of how TCM has managed to explain the ideal gas laws. Although not addressed, other phenomena, such as diffusion and effusion of gases, can also be explained with TCM.
If the volume of the container is compressed at constant temperature, the distance that the gaseous particles must travel to collide with the walls decreases; which is equal to an increase in the frequency of said collisions, resulting in greater pressure. As the temperature remains constant, ECmp it is also constant.
If you increase T, ECmp will increase. The gaseous particles will move faster and collide a greater number of times with the walls of the container; so the pressure increases.
If the walls are flexible, able to expand, their area will become larger and the pressure will drop until it becomes constant; and as a result, the volume will also increase.
If several liters of different gases were added to a spacious container, coming from smaller containers, its total internal pressure would be equal to the sum of the partial pressures exerted by each type of gas separately..
Why? Because all gases begin to collide with each other and disperse homogeneously; the interactions between them are null, and the vacuum predominates in the container (TCM postulates), so it is as if each gas were alone, exerting its pressure individually without the interference of the other gases.
Yet No Comments