Parabolic shooting characteristics, formulas and equations, examples

The parabolic shot It consists of throwing an object or projectile at a certain angle and letting it move under the action of gravity. If air resistance is not considered, the object, regardless of its nature, will follow a parabola arc path.

It is a daily movement, since among the most popular sports are those in which balls or balls are thrown, either with the hand, with the foot or with an instrument such as a racket or a bat for example.

For its study, the parabolic shot is broken down into two superimposed movements: one horizontal without acceleration, and the other vertical with constant downward acceleration, which is gravity. Both movements have initial speed.

Let's say that the horizontal motion runs along the x-axis and the vertical motion along the y-axis. Each of these movements is independent of the other.

Since determining the position of the projectile is the main objective, it is necessary to choose an appropriate reference system. Details are below.

Article index

• 1 Parabolic shot formulas and equations
  • 1.1 - Trajectory, maximum height, maximum time and horizontal reach
• 2 Examples of parabolic shooting
  • 2.1 Parabolic shooting in human activities
  • 2.2 The parabolic shot in nature
• 3 Exercise
• 4 References

Parabolic shot formulas and equations

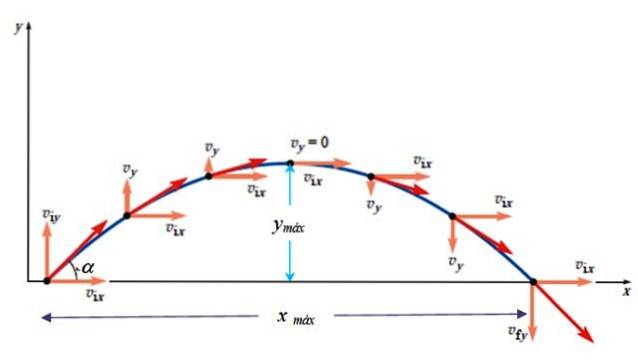
Suppose the object is thrown with angle α with respect to the horizontal and initial velocity v_or as shown in the figure below left. The parabolic shot is a movement that takes place on the plane xy and in that case the initial velocity breaks down like this:

v_ox = v_or cos α

v_hey = v_or sin α

The position of the projectile, which is the red dot in figure 2, right image, also has two time-dependent components, one in x and the other in Y. Position is a vector denoted as r and its units are of length.

In the figure, the initial position of the projectile coincides with the origin of the coordinate system, therefore x_or = 0, and_or = 0. This is not always the case, you can choose the origin anywhere, but this choice greatly simplifies the calculations.

As for the two motions in x and in y, these are:

-x (t): is a uniform rectilinear motion.

-y (t): corresponds to a uniformly accelerated rectilinear motion with g = 9.8 m / s^two and pointing vertically down.

In mathematical form:

x (t) = v_or cos α.t

y (t) = v_or .sin α.t - ½g.t^two

The position vector is:

r (t) = [v_or cos α.t]i + [v_or .sin α.t - ½g.t^two] j

In these equations the attentive reader will notice that the minus sign is due to gravity pointing towards the ground, the direction chosen as negative, while upwards is taken as positive..

Since velocity is the first derivative of position, simply derive r (t) with respect to time and obtain:

v (t) = v_or cos α i + (v_or .sin α - gt) j

Finally, the acceleration is expressed vectorially as:

to (t) = -g j

- Trajectory, maximum height, maximum time and horizontal reach

Trajectory

To find the explicit equation of the trajectory, which is the curve y (x), we must eliminate the time parameter, solving in the equation for x (t) and substituting in y (t). The simplification is somewhat laborious, but finally you get:

Maximum height

The maximum height occurs when v_Y = 0. Knowing that there is the following relationship between position and the square of the velocity:

v_Y^two = v_hey ^two- 2gy

Doing v_Y = 0 just when reaching the maximum height:

0 = v_hey ^two- 2g. And_max → and_max = v_hey ^two/2 g

With:

v_hey = v_or senα

Maximum time

The maximum time is the time it takes for the object to reach and_max. To calculate it is used:

v_Y = v_or .sin α - gt

Knowing that v_Y becomes 0 when t = t_max, result:

v_or .sin α - g.t_max = 0

t_max = v_hey / g

Maximum horizontal reach and flight time

The range is very important, because it signals where the object will fall. This way we will know whether or not it hits the target. To find it we need the flight time, total time or t_v.

From the above illustration it is easy to conclude that t_v = 2.t_max. But beware! This is only true if the launch is level, that is, the height of the starting point is the same as the height of the arrival. Otherwise time is found by solving the quadratic equation that results from substituting the final position Y_final:

Y_final = v_or .sin α.t_v - ½g.t_v^two

In any case, the maximum horizontal reach is:

x_max = v_ox. t_v

Examples of parabolic shooting

Parabolic shooting is part of the movement of people and animals. Also of almost all sports and games where gravity intervenes. For example:

Parabolic shooting in human activities

-The stone thrown by a catapult.

-The goalkeeper's goal kick.

-The ball thrown by the pitcher.

-The arrow that comes out of the bow.

-All kinds of jumps

-Throw a stone with a sling.

-Any thrown weapon.

The parabolic shot in nature

-The water that gushes from natural or artificial jets such as those of a fountain.

-Stones and lava gushing out of a volcano.

-A ball that bounces off the pavement or a stone that bounces on water.

-All kinds of jumping animals: kangaroos, dolphins, gazelles, cats, frogs, rabbits or insects, to name a few.

Exercise

A grasshopper jumps at an angle of 55º with the horizontal and lands 0.80 meters ahead. Find:

a) The maximum height reached.

b) If he jumped with the same initial speed, but forming an angle of 45º, would he go higher??

c) What can be said about the maximum horizontal reach for this angle?

Solution to

When the data supplied by the problem do not contain the initial velocity v_or the calculations are somewhat more laborious, but from the known equations, a new expression can be derived. Starting from:

x_max = v_ox . t_flight = v_or.cos α. t_v

When it lands later, the height returns to 0, so:

v_or .sin α.t_v - ½g.t_v^two= 0

What t_v is a common factor, it is simplified:

v_or .sin α - ½g.t_v= 0

We can clear t_v from the first equation:

t_v = x_max / v_or.cos α

And replace in the second:

v_or .sin α - (½g.x_max / v_or.cos α) = 0

By multiplying all the terms by v_or.cos αthe expression is not altered and the denominator disappears:

(v_or .sin α.) (v_or.cos α) - ½g.x_max = 0

v_or^two sin α. cos α = ½g.x_max

It can already be cleared v_or or also substitute the following identity:

sin 2α = 2 sin α. cos α → v_or^two sin 2α = g.x_max

Is calculated v_or^two:

v_or^two = g.x_max / sin 2α = (9.8 x 0.8 / sin 110) m^two/ s^two = 8.34 m^two/ s^two

And finally the maximum height:

Y_max= v_hey ^two/ 2g = (8.34 x sin^two 55) / (2 x 9.8) m = 0.286 m = 28.6 cm

Solution b

The lobster manages to maintain the same horizontal speed, but by decreasing the angle:

Y_max= v_hey ^two/ 2g = (8.34 x sin^two 45) / (2 x 9.8) m = 0.213 m = 21.3 cm

Reaches a lower height.

Solution c

The maximum horizontal reach is:

x_max = v_or^two sen 2nd / g

By varying the angle, the horizontal reach also changes:

x_max = 8.34 sen 90 / 9.8  m = 0.851 m = 85.1 cm

The jump is longer now. The reader can verify that it is maximum for the angle of 45º since:

sin 2α = sin 90 = 1.

References

1. Figueroa, D. 2005. Series: Physics for Sciences and Engineering. Volume 1. Kinematics. Edited by Douglas Figueroa (USB).
2. Giambattista, A. 2010. Physics. Second Edition. Mcgraw hill.
3. Giancoli, D. 2006. Physics: Principles with Applications. 6th. Ed prentice hall.
4. Resnick, R. 1999. Physics. Vol. 1. 3rd Ed. In Spanish. Compañía Editorial Continental S.A. by C.V.
5. Sears, Zemansky. 2016. University Physics with Modern Physics. 14th. Ed. Volume 1.