
Formula work, units, examples, exercises

The job In Physics it is the transfer of energy carried out by a force when the object on which it acts moves. Mathematically it takes the form of the scalar product between the force vectors F and displacement s.
And since the scalar product between two perpendicular vectors is zero, it happens that the forces that form 90º with the displacement do not do work, according to the definition, since:
W = F ● s = F⋅ s⋅ cos θ
Where W denotes work, from the English word work.
The advantage of defining the work is that it is a scalar, that is, it has no direction or sense, only module and the respective unit. This makes it easier to carry out calculations involving energy changes caused by the action of forces..
Gravity and kinetic friction are examples of forces that often do work on moving objects. Another common force is the normal one exerted by a surface, but unlike those, it never does work on objects, as it is perpendicular to the displacement..
When a body falls freely, gravity does positive work on the mobile, causing it to increase in speed as it falls. On the other hand, kinetic friction has a completely opposite effect, since as it always opposes movement, it carries out negative work that does not favor it..
Article index
- 1 Formulas and particular cases
- 1.1 Work done by variable forces
- 2 work units
- 2.1 Units in the British system
- 2.2 Other units for work
- 3 Examples of work
- 3.1 Ascent and descent of objects
- 3.2 Point charges in electric fields
- 3.3 Friction between surfaces
- 3.4 Push and pull
- 3.5 Force in a pulley
- 3.6 Normal forces or supports
- 3.7 Magnetic force
- 3.8 Objects tied to a rope
- 3.9 Satellites in orbit
- 3.10 Mass-spring system
- 4 Exercise resolved
- 5 References
Formulas and particular cases
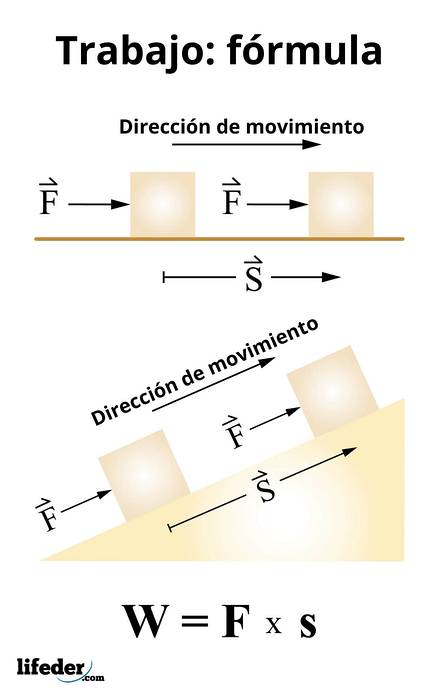
The work is calculated by:
W = F ● s
This expression is valid for constant forces and according to the definition of a scalar product, it is equivalent to:
W = F. s. cos θ
Where θ is the angle between the force and the displacement. It follows, then, that only those forces that have a component in the direction of displacement can do work on a body.
And it also becomes clear that if there is no movement, there is no work either.
As for the sign, the work can be positive, negative or zero. In case the force has a component parallel to the motion, the sign of the work depends on the value of cos θ.
There are some particular cases that are worth considering:
- When the force is parallel to the displacement, the angle between F Y s is 0º, therefore the work done by the force is positive and its value is maximum:
W = F⋅s cos 0º = F⋅s
- If the force opposes the displacement then the angle between F Y s is 180º, the work done by F is negative and it is minimal:
W = F⋅s cos 180º = -F⋅s
- Finally, there is the case mentioned before: if the angle formed by F Y s is 90º, since cos 90º = 0, the work is null:
W = F⋅s cos 90º = 0
Work done by variable forces
Sometimes the applied force is not constant; in that case, you have to appeal to the calculation to find the work done. First, a work differential dW is determined, performed on an infinitesimal displacement ds:
dW = F⋅ds
To find the value of the total work done by this force when the object goes from point A to point B, it is necessary to integrate both sides, like this:
Work units
The unit for work in the International System is the joule, abbreviated J. The unit takes its name from the English physicist James Prescott Joule, a pioneer in the study of Thermodynamics..
From the equation of work, the joule is defined as 1 newton per meter:
1 J = 1 N⋅m
Units in the British system
Work corresponds as a unit pound-force x foot, sometimes call pound-force foot. It is also a unit for energy, but it must be remembered that work done on a body changes its energy state and that therefore work and energy are equivalent. No wonder they have the same drives.
The equivalence between the pound-force foot and the joule is as follows:
1 foot-pound-force = 1.35582 J
A well-known unit for work and energy, especially for the field of refrigeration and air conditioning is the BTU or British Thermal Unit.
1 BTU equals 1055 J and 778,169 foot-pound-force.
Other units for work
There are other units for work that are used in specific areas of physics and engineering. Among them we have:
Erg
Denoted as erg, is the unit of work in the cegesimal system and is equal to 1 dyna⋅cm or 1 x 10-7 J.
Electron-volt
Abbreviated eV, it is commonly used in particle physics and is defined as the energy that an electron acquires when it moves through a potential difference of 1 V.
Kilowatt-hour (kWh)
It appears frequently on utility bills. It is the work carried out during 1 hour by a source whose power is 1 kW, equivalent to 3.6 x 106 J.
Calorie
It is usually related to the energy of food, although in fact in this context reference is made to a kilocalorie, that is, 1000 calories. Actually there are several units that receive this name, so the context must be specified very well.
The equivalence between the joule and 1 thermochemical calorie it is:
1 calorie = 4.1840 J
Work examples
Ascent and descent of objects
When bodies descend, either vertically or down a ramp, the weight does positive work, favoring movement. Instead, whenever an object ascends, gravity does negative work.
Point charges in electric fields
A uniform electric field does work on a point charge moving inside it. Depending on the field and the sign of the charge, this work can be negative or positive.
Friction between surfaces
Kinetic friction between surfaces always does negative work on the moving object.
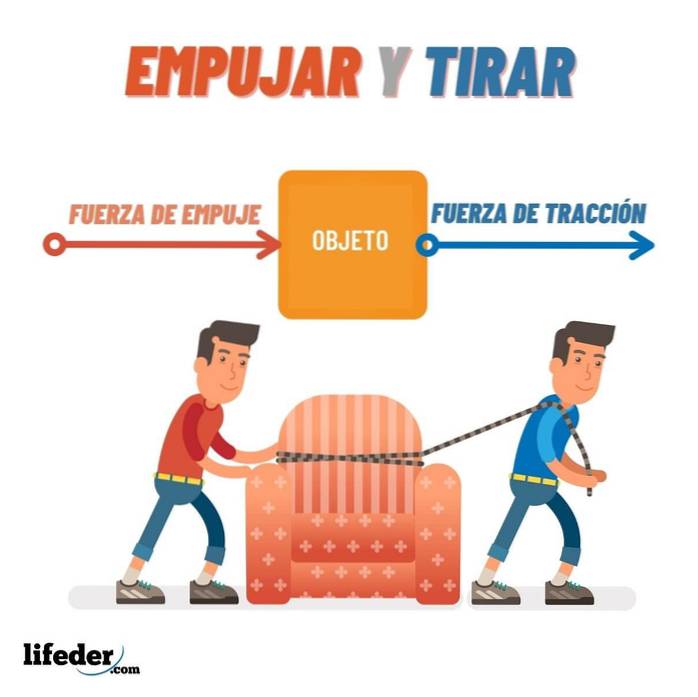
Push and pull

Pushing is a force that pulls an object away from something. Pulling is a force that makes an object bring itself closer.
Force in a pulley
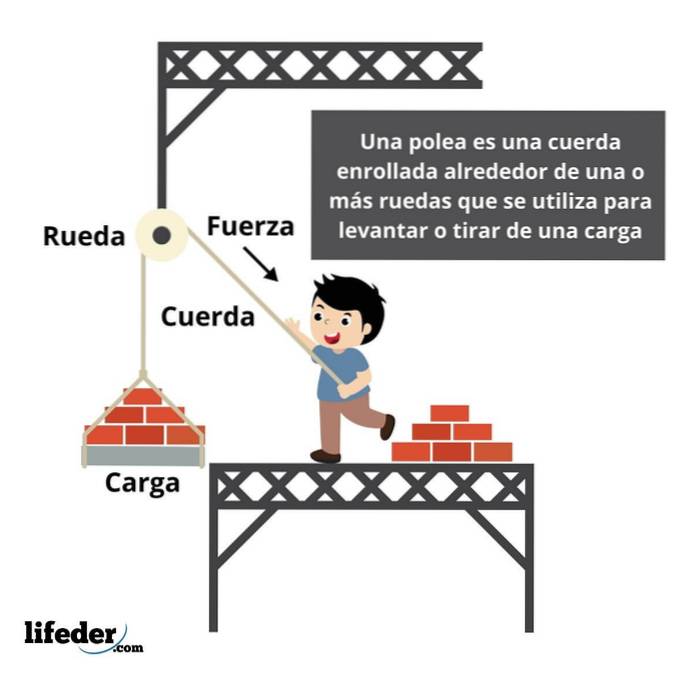
A pulley is a system used to transmit a force from one of its ends. In a simple pulley, in order to lift the load, a force equal to the resistance exerted by the object must be applied.
Normal forces or supports
The normal, as noted above, does zero work when an object resting on a surface moves over it, even if the surface is not flat or if it is inclined..
Magnetic force
Another force that does zero work is the magnetic force that exerts a uniform field on the charged particle that is perpendicular to it. The motion of the particle turns out to be a uniform circular motion, with the force in the radial direction. Since the displacement is perpendicular to the force, it does not do work on the load..
Objects tied to a rope
A rope also does not do work on a suspended pendulum, since the tension in it is always perpendicular to the displacement of the mass..
Satellites in orbit
Gravity also does not work on a satellite in circular orbit, for the same reason as the previous cases: it is perpendicular to the displacement..
Mass-spring system
In a mass-spring system, the force F that the spring exerts on the mass has magnitude F = kx, where k is the spring constant and x its compression or elongation. It is a variable force, therefore the work it does depends on how much the spring stretches or shrinks.
Exercise resolved
The following graph shows the work done by a variable force Fx which depends on the position x. This is the force exerted by a hammer on a nail. The first part is the force used to nail on the softer section of the wall and the second to finish driving the nail.
How much work does the hammer have to do for the nail to sink a total of 5 cm into the wall?
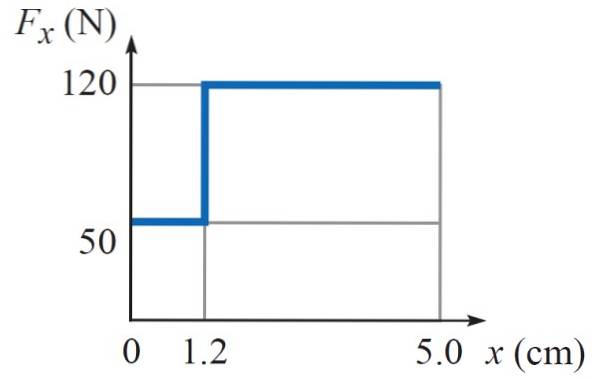
Solution
The force exerted by the hammer is variable, since less intensity (50 N) is required to drive the nail 1.2 cm into the soft part of the wall, while in the harder part, it takes 120 N to make the nail sink down to 5 cm deep, as shown in the chart.
In this case the work is the integral:

Where A = 0 cm and B = 5 cm. Since the integral is the area under the graph Fx vs x, it is enough to find this area, which corresponds to two rectangles, the first with height 50 N and width 1.2 cm, and the second with height 120 N and width (5 cm - 1.2 cm) = 3.8 cm.
Both are calculated and added to give the total work:
W = 50 N x 1.2 cm + 120 N x 3.8 cm = 516 N.cm = 516 N x 0.01 m = 5.16 J.
References
- Figueroa, D. (2005). Series: Physics for Science and Engineering. Volume 2. Dynamics. Edited by Douglas Figueroa (USB).
- Giambattista, A. 2010. Physics. 2nd. Ed. McGraw Hill.
- Sears, Zemansky. 2016. University Physics with Modern Physics. 14th. Ed. Volume 1. Pearson.
- Serway, R., Jewett, J. (2008). Physics for Science and Engineering. Volume 1. 7th. Ed. Cengage Learning.
- Zapata, F. Mechanical work. Recovered from: francesphysics.blogspot.com.



Yet No Comments