Equilateral triangle characteristics, properties, formulas, area

A equilateral triangle it is a polygon with three sides, where all are equal; that is, they have the same measure. For this characteristic it was given the name of equilateral (equal sides).

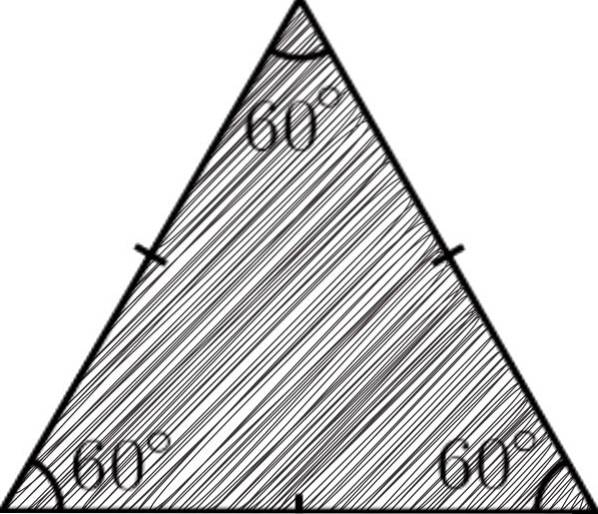
Triangles are polygons considered the simplest in geometry, because they are made up of three sides, three angles, and three vertices. In the case of the equilateral triangle, since it has equal sides, it implies that its three angles will also be equal..

Article index

• 1 Characteristics of equilateral triangles
  • 1.1 - Equal sides
  • 1.2 - Components
• 2 Properties
  • 2.1 Internal angles
  • 2.2 External angles
  • 2.3 Sum of the sides
  • 2.4 Congruent sides
  • 2.5 Congruent angles
• 3 How to calculate the perimeter?
• 4 How to calculate the height?
• 5 How to calculate the sides?
• 6 How to calculate the area?
• 7 Exercises
  • 7.1 - First exercise
  • 7.2 - Second exercise
  • 7.3 - Third exercise
• 8 References

Characteristics of equilateral triangles

- Equal sides

Equilateral triangles are flat and closed figures, made up of three line segments. Triangles are classified by their characteristics, in relation to their sides and angles; the equilateral was classified using the measure of its sides as a parameter, since these are exactly the same, that is, they are congruent.

The equilateral triangle is a particular case of the isosceles triangle because two of its sides are congruent. That is why all equilateral triangles are also isosceles, but not all isosceles triangles will be equilateral.

In this way, equilateral triangles have the same properties as an isosceles triangle..

Equilateral triangles can also be classified by the width of their interior angles as an equilateral acute triangle, which has three sides and three interior angles with the same measure. The angles will be acute, that is, they will be less than 90^or.

- Components (edit)

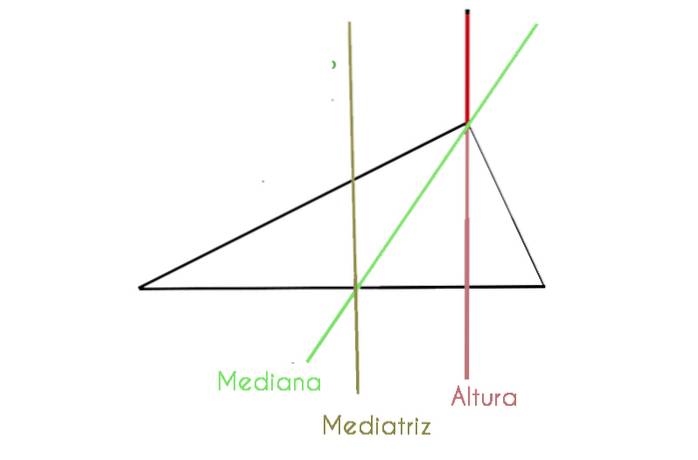
Triangles in general have several lines and points that compose it. They are used to calculate the area, the sides, the angles, the median, bisector, the bisector and the height..

• Median: is a line that starts from the midpoint of one side and reaches the opposite vertex. The three medians meet at a point called the barycenter or centroid..
• Bisector: it is a ray that divides the angle of the vertices into two angles of equal measure, that is why it is known as the axis of symmetry. The equilateral triangle has three axes of symmetry. In the equilateral triangle, the bisector is drawn from the vertex of an angle to its opposite side, cutting it at its midpoint. These meet at a point called incenter.
• The mediatrix: is a segment perpendicular to the side of the triangle that has its origin in the middle of it. There are three mediatices in a triangle and they meet at a point called the circumcenter.
• The height: is the line that goes from the vertex to the side that is opposite and also this line is perpendicular to that side. All triangles have three heights that coincide at a point called the orthocenter..

In the following graph we see a scalene triangle where some of the components mentioned are detailed

The bisector, median and bisector are coincident

The bisector divides the side of a triangle into two parts. In equilateral triangles that side will be divided into two exactly equal parts, that is, the triangle will be divided into two congruent right triangles.

Thus, the bisector drawn from any angle of an equilateral triangle coincides with the median and the bisector of the side opposite that angle.

Example:

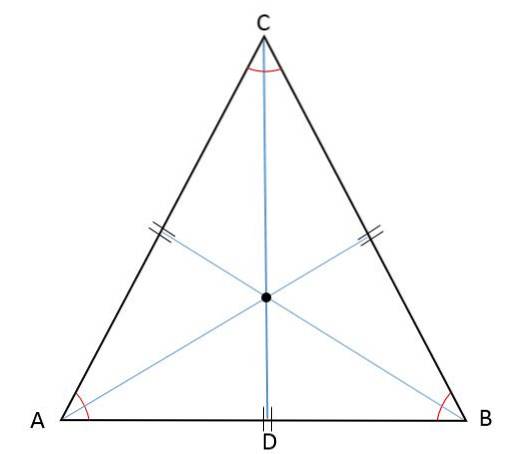
The following figure shows the triangle ABC with a midpoint D that divides one of its sides into two segments AD and BD.

By drawing a line from point D to the opposite vertex, by definition the median CD is obtained, which is relative to vertex C and side AB.

Since the segment CD divides the triangle ABC into two equal triangles CDB and CDA, it means that the congruence case will be had: side, angle, side and therefore CD will also be the bisector of BCD.

When plotting segment CD, divide the vertex angle into two equal angles of 30^or, the angle of vertex A still measures 60^or and the line CD forms an angle of 90^or with respect to the midpoint D.

The segment CD forms angles that have the same measure for the triangles ADC and BDC, that is, they are supplementary in such a way that the measure of each one will be:

Med. (ADB) + Med. (ADC) = 180^or

two _* Med. (ADC) = 180^or

Med. (ADC) = 180^or ÷ 2

Med. (ADC) = 90^or.

And so, we have that the segment CD is also the bisector of the side AB.

The bisector and the height are coincident

By drawing the bisector from the vertex of one angle to the midpoint of the opposite side, it divides the equilateral triangle into two congruent triangles.

In such a way that an angle of 90 is formed^or (right). This indicates that that line segment is totally perpendicular to that side, and by definition that line would be the height.

In this way, the bisector of any angle of an equilateral triangle coincides with the height relative to the opposite side of that angle..

Ortocenter, barycenter, incenter, and coincident circumcenter

As the height, median, bisector and bisector are represented by the same segment at the same time, in an equilateral triangle the meeting points of these segments -the orthocenter, bisector, incenter and circumcenter- will be found at the same point:

Properties

The main property of equilateral triangles is that they will always be isosceles triangles, since isosceles are formed by two congruent sides and equilateral by three..

In this way, the equilateral triangles inherited all the properties of the isosceles triangle:

Internal angles

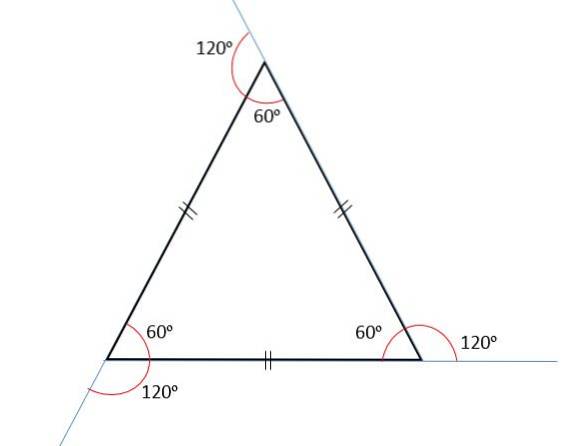
The sum of the interior angles is always equal to 180^or, and since all its angles are congruent, then each of these will measure 60^or.

External angles

The sum of the exterior angles will always equal 360^or, therefore each external angle will measure 120^or. This is because the internal and external angles are supplementary, that is, when adding them they will always be equal to 180^or.

Sum of the sides

The sum of the measures of two sides must always be greater than the measure of the third side, that is, a + b> c, where a, b and c are the measures of each side.

Congruent sides

Equilateral triangles have all three sides with the same measure or length; that is, they are congruent. Therefore, in the previous item we have that a = b = c.

Congruent angles

Equilateral triangles are also known as equiangular triangles, because their three interior angles are congruent with each other. This is because all its sides also have the same measurement.

How to calculate the perimeter?

The perimeter of a polygon is calculated by adding the sides. As in this case the equilateral triangle has all its sides with the same measure, its perimeter is calculated with the following formula:

P = 3 _* side.

How to calculate the height?

Since the height is the line perpendicular to the base, it divides it into two equal parts by extending to the opposite vertex. This is how two equal right triangles are formed.

The height (h) represents the opposite leg (a), half of the side AC to the adjacent leg (b) and the side BC represents the hypotenuse (c).

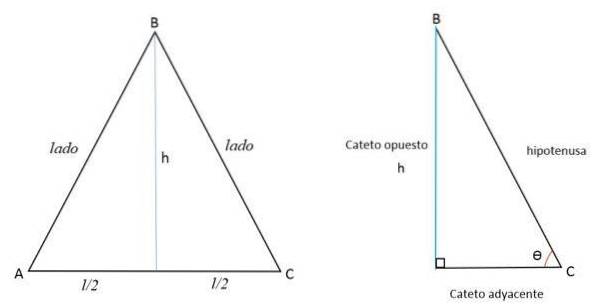
Using the Pythagorean theorem, the value of the height can be determined:

to^two + b^two = c^two

Where:

to^two = height (h).

b^two = side b / 2.

c^two = side a.

Substituting these values ​​in the Pythagorean theorem, and solving for the height, we have:

h^two + ( l / two)^two = l^two

h^two +  l^two/ 4 = l^two

h^two = l^two  -  l^two/ 4

h^two = (4_*l^two -  l^two) / 4

h^two =  3_*l^two /4

√h^two = √ (3_*l^two /4)

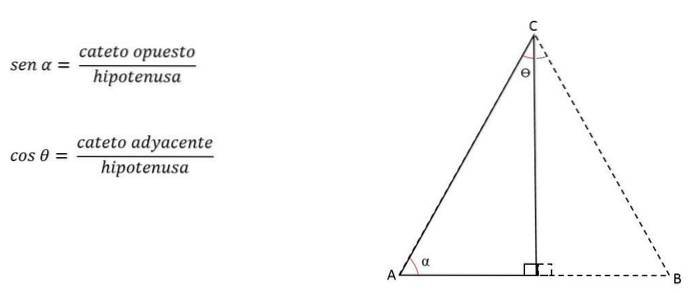
If the angle formed by the congruent sides is known, the height (represented by a leg) can be calculated by applying the trigonometric ratios.

The legs are called opposite or adjacent depending on the angle taken as a reference..

For example, in the previous figure, leg h will be opposite for angle C, but adjacent to angle B:

Thus, the height can be calculated with:

How to calculate the sides?

There are cases where the measures of the sides of the triangle are not known, but their height and the angles that are formed at the vertices.

To determine the area in these cases it is necessary to apply the trigonometric ratios.

Knowing the angle of one of its vertices, the legs are identified and the corresponding trigonometric ratio is used:

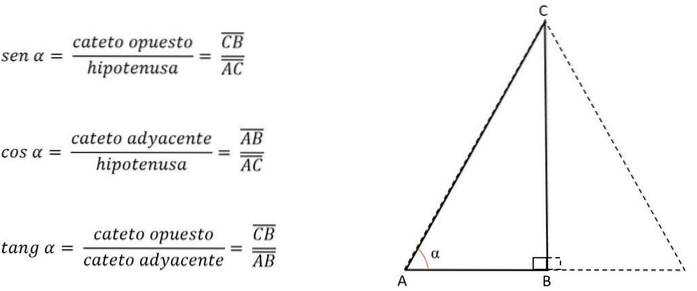
Thus, leg AB will be opposite for angle C, but adjacent to angle A. Depending on the side or leg corresponding to the height, the other side is cleared to obtain its value, knowing that in an equilateral triangle the three sides will always have the same measurement.

How to calculate the area?

The area of ​​the triangles is always calculated with the same formula, multiplying the base times the height and dividing by two:

Area = (b _* h) ÷ 2

Knowing that the height is given by the formula:

Training

- First exercise

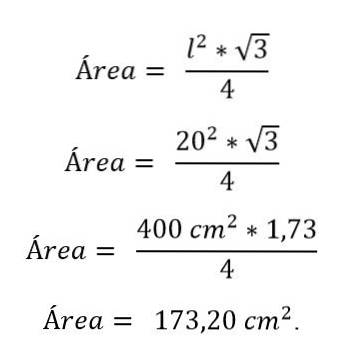
The sides of an equilateral triangle ABC are 20 cm each. Calculate the height and area of ​​that polygon.

Solution

To determine the area of ​​this equilateral triangle, it is necessary to calculate the height, knowing that when drawing it, it divides the triangle into two equal right triangles.

In this way, the Pythagorean theorem can be used to find it:

to^two + b^two = c^two

Where:

a = 20/2 = 10 cm.

b = height.

c = 20 cm.

The data are substituted in the theorem:

10^two + b^two = 20^two

100 cm + b^two = 400 cm

b^two = (400 - 100) cm

b^two = 300cm

b = √300 cm

b = 17.32 cm.

That is, the height of the triangle is equal to 17.32cm. Now it is possible to calculate the area of ​​the given triangle by substituting in the formula:

Area = (b _* h) ÷ 2

Area = (20 cm _* 17.32 cm) ÷ 2

Area = 346.40 cm^two ÷ 2

Area = 173.20 cm^two.

Another simpler way to solve the exercise is by substituting the data in the direct formula for the area, where the value of the height is also found implicitly:

- Second exercise

Flowers will be planted on a piece of land that has the shape of an equilateral triangle. If the perimeter of that land is equal to 450 m, calculate the number of square meters that the flowers will occupy.

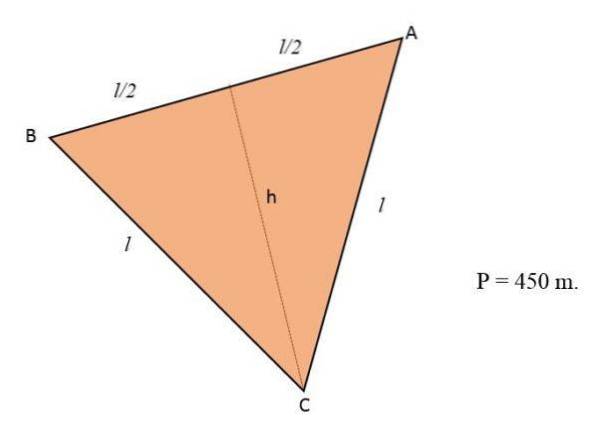
Solution

Knowing that the perimeter of a triangle corresponds to the sum of its three sides and since the terrain has the shape of an equilateral triangle, the three sides of this will have the same measure or length:

P = side + side + side = 3 _* l

3 _* l = 450 m.

l = 450 m ÷ 3

l = 150 m.

Now it is only necessary to calculate the height of that triangle.

The height divides the triangle into two congruent right triangles, where one leg represents the height and the other half the base. By the Pythagorean theorem, the height can be determined:

to^two + b^two = c^two

Where:

to = 150 m ÷ 2 = 75 m.

c = 150 m.

b = height

The data are substituted in the theorem:

(75 m)^two + b^two = (150 m)^two

5,625 m + b^two = 22,500 m

b^two = 22,500 m - 5,625 m

b^two = 16,875 m

b = √16.875 m

b = 129.90 m.

Thus the area that the flowers will occupy will be:

Area = b * h ÷ 2

Area = (150 m _* 129.9 m) ÷ 2

Area = (19,485 m^two) ÷ 2

Area = 9,742.5 m^two

- Third exercise

The equilateral triangle ABC is divided by a line segment that goes from its vertex C to the midpoint D, located on the opposite side (AB). This segment measures 62 meters. Calculate the area and perimeter of that equilateral triangle.

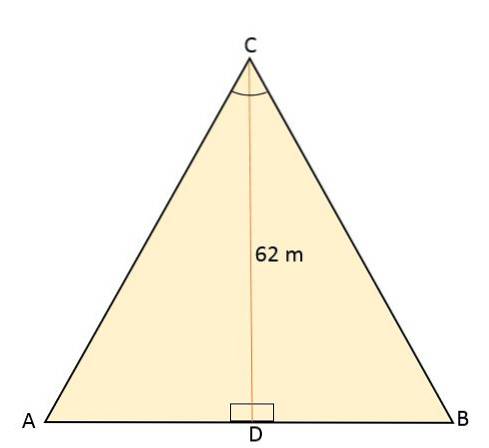
Solution

Knowing that the equilateral triangle is divided by a line segment that corresponds to the height, thus forming two congruent right triangles, this in turn also divides the angle of the vertex C into two angles with the same measure, 30^or each.

The height forms an angle of 90^or with respect to segment AB, and the angle of vertex A will then measure 60^or.

Then using the angle of 30 as a reference^or, the height CD is set as the leg adjacent to the angle and BC as the hypotenuse.

From these data, the value of one of the sides of the triangle can be determined, using the trigonometric ratios:

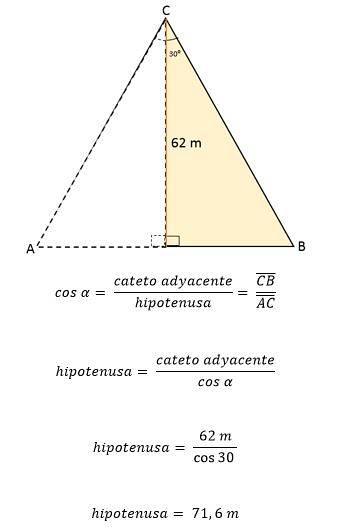
Since in the equilateral triangle all the sides have exactly the same measure or length, it means that each side of the equilateral triangle ABC is equal to 71.6 meters. Knowing that, it is possible to determine its area:

Area = b * h ÷ 2

Area = (71.6 m _* 62 m) ÷ 2

Area = 4,438.6 m^two ÷ 2

Area = 2,219.3 m^two

The perimeter is given by the sum of its three sides:

P = side + side + side = 3 _* l

P = 3_*l

P = 3 _* 71.6 m

P = 214.8 m.

References

1. Álvaro Rendón, A. R. (2004). Technical Drawing: activity book.
2. Arthur Goodman, L. H. (1996). Algebra and trigonometry with analytical geometry. Pearson Education.
3. Baldor, A. (1941). Algebra. Havana: Culture.
4. BARBOSA, J. L. (2006). Plane Euclidean Geometry. SBM. Rio de Janeiro, .
5. Coxford, A. (1971). Geometry A Transformation Approach. USA: Laidlaw Brothers.
6. Euclid, R. P. (1886). Euclid's Elements of Geometry.
7. Héctor Trejo, J. S. (2006). Geometry and trigonometry.
8. León Fernández, G. S. (2007). Integrated Geometry. Metropolitan Technological Institute.
9. Sullivan, J. (2006). Algebra and Trigonometry. Pearson Education.