Congruence congruent figures, criteria, examples, exercises

The congruence, In geometry, it points out that if two plane figures have the same shape and dimensions, they are congruent. For example, two segments are congruent when their lengths are equal. Likewise, congruent angles have the same measure, even though they are not oriented in the same way in the plane..

The term "congruence" comes from Latin congruent, whose meaning is correspondence. Thus, two congruent figures correspond exactly to each other..

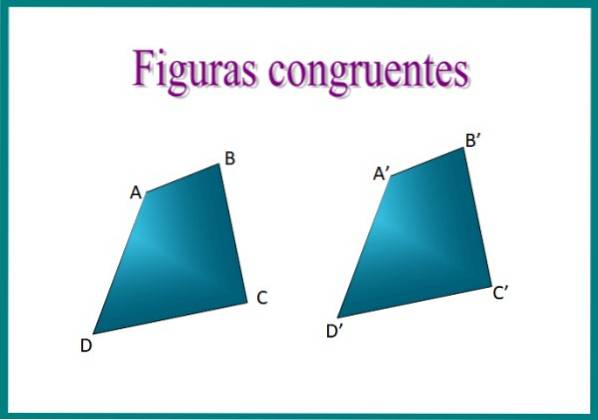
For example, if we superimpose the two quadrilaterals in the image, we will find that they are congruent, since the arrangement of their sides is identical and they measure the same.

By placing quadrilaterals ABCD and A'B'C'D 'on top of each other, the figures will match exactly. The matching sides are called homologous sides or corresponding and to express congruence the symbol ≡ is used. Then we can state that ABCD ≡ A'B'C'D '.

Article index

• 1 Congruence criteria
  • 1.1 Congruence, identity and similarity
• 2 Examples of congruence
  • 2.1 - Angle congruence
  • 2.2 - Congruence of triangles
• 3 Solved exercises
  • 3.1 - Exercise 1
  • 3.2 - Exercise 2
• 4 References

Congruence criteria

The following characteristics are common to congruent polygons:

-Same shape and size.

-Identical measures of their angles.

-The same measure on each of its sides.

In the case that two polygons in question are regular, that is, that all the sides and internal angles measure the same, congruence is assured when it is fulfilled some of the following conditions:

-The sides are congruent

-The apothems they have the same measure

-The radio of each polygon measures equal

The apothem of a regular polygon is the distance between the center and one of the sides, while the radius corresponds to the distance between the center and a vertex or corner of the figure.

Congruence criteria are frequently used because so many parts and pieces of all kinds are mass-produced and must have the same shape and measurements. In this way they can be easily replaced when necessary, for example nuts, bolts, sheets or the paving stones on the ground in the street.

Congruence, identity and similarity

There are geometric concepts related to congruence, for example identical figures and the similar figures, that do not necessarily imply that the figures are congruent.

Note that the congruent figures are identical, however the quadrilaterals in Figure 1 could be oriented in different ways on the plane and still remain congruent, since the different orientation does not change the size of their sides or their angles. In this case they would cease to be identical.

The other concept is that of the similarity of figures: two plane figures are similar if they have the same shape and their internal angles measure the same, although the size of the figures may be different. If this is the case, the figures are not congruent.

Examples of congruence

- Angle congruence

As we indicated at the beginning, congruent angles have the same measure. There are several ways to obtain congruent angles:

Example 1

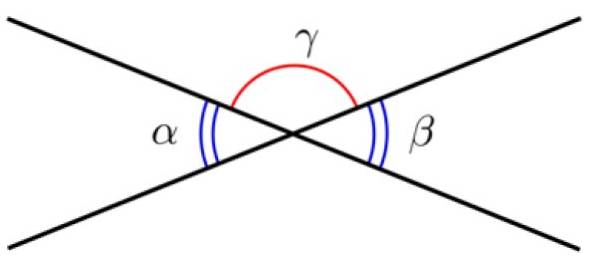
Two lines with a point in common define two angles, called Opposite angles by the vertex. These angles have the same measure, therefore they are congruent.

Example 2

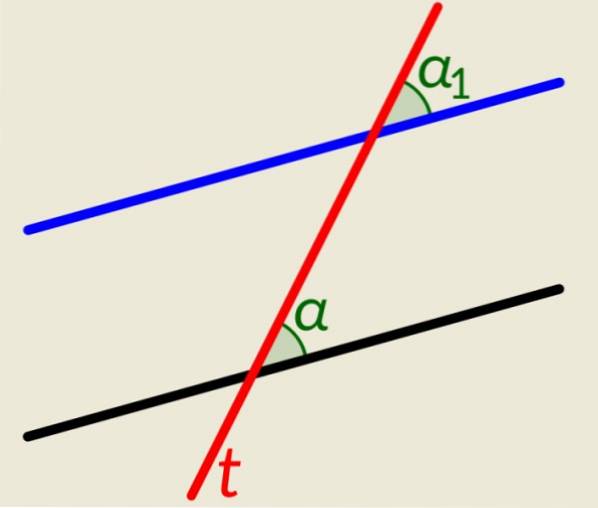
There are two parallel lines plus one line t that intersects them both. As in the previous example, when this line intersects the parallels it generates congruent angles, one on each line on the right side and two on the left side. The figure shows α and α₁, to the right of the line t, that are congruent.

Example 3

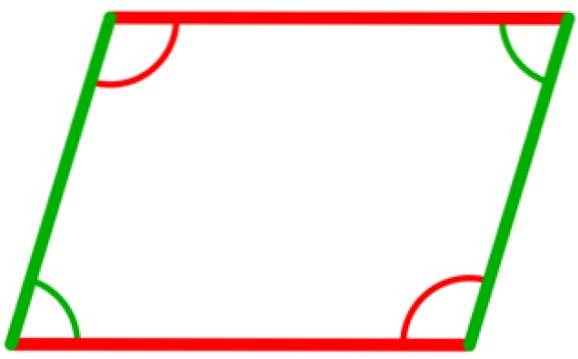
In a parallelogram there are four interior angles, which are congruent two to two. They are the ones between opposite vertices, as shown in the following figure, in which the two angles in green are congruent, as well as the two angles in red..

- Congruence of triangles

Two triangles of the same shape and size are congruent. To verify this there are three criteria that can be examined in search of congruence:

-Criterion LLL: the three sides of the triangles have the same measurements, therefore L₁ = L '₁; L_two = L '_two and L₃ = L '_3.

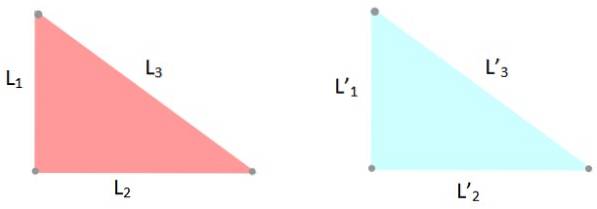
-ALA and AAL criteria: triangles have two equal interior angles and the side between those angles has the same measure.

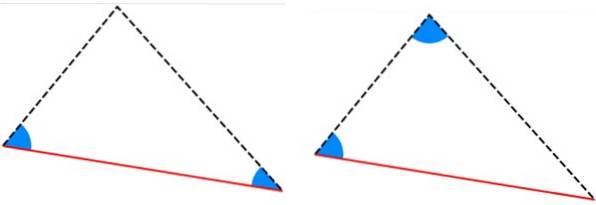
-LAL criterion: two of the sides are identical (corresponding) and between them there is the same angle.

Solved exercises

- Exercise 1

Two triangles are shown in the following figure: ΔABC and ΔECF. It is known that AC = EF, that AB = 6 and that CF = 10. Furthermore, the angles ∡BAC and ∡FEC are congruent and the angles ∡ACB and ∡FCB are also congruent..

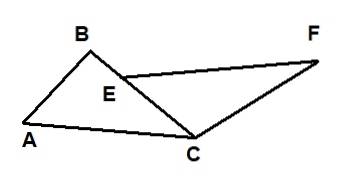
Then the length of segment BE is equal to:

(i) 5 

(ii) 3

(iii) 4 

(iv) 2

(v) 6

Solution

As the two triangles have a side of equal length AC = EF comprised between the equal angles ∡BAC = ∡CEF and ∡BCA = ∡CFE, it can be said that the two triangles are congruent by the ALA criterion.

That is, ΔBAC ≡ ΔCEF, so we have to:

BA = CE = AB = 6

BC = CF = 10

AC = EF

But the segment to be calculated is BE = BC - EC = 10 - 6 = 4.

So the correct answer is (iii).

- Exercise 2

Three triangles are shown in the figure below. It is also known that the two indicated angles measure 80º each and that the segments AB = PD and AP = CD. Find the value of the angle X indicated in the figure.

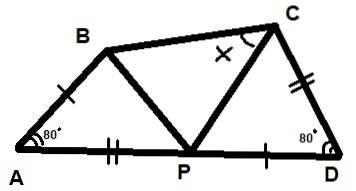
Solution

You have to apply the properties of the triangles, which are detailed step by step.

Step 1

Starting with the LAL triangle congruence criterion, it can be stated that the BAP and PDC triangles are congruent:

ΔBAP ≡ ΔPDC

Step 2

The above leads to affirm that BP = PC, therefore the triangle ΔBPC is isosceles and ∡PCB = ∡PBC = X.

Step 3

If we call the angle BPC γ, it follows that:

2x + γ = 180º

Step 4

And if we call the angles APB and DCP β and α the angles ABP and DPC, we have:

α + β + γ = 180º (since APB is a plane angle).

Step 5

Furthermore, α + β + 80º = 180º by sum of the internal angles of the triangle APB.

Step 6

Combining all these expressions we have:

α + β = 100º

Step 7

And therefore:

γ = 80º.

Step 8

Finally it follows that:

2X + 80º = 180º

With X = 50º.

References

1. Baldor, A. 1973. Plane and Space Geometry. Central American Cultural.
2. CK-12 Foundation. Congruent Polygons. Recovered from: ck 12.org.
3. Enjoy math. Definitions: Radius (polygon). Recovered from: gustolasmatematicas.com.
4. Math Open Reference. Testing Polygons for congruence. Recovered from: mathopenref.com.
5. Wikipedia. Congruence (geometry). Recovered from: es.wikipedia.org.
6. Zapata, F. Triangles, history, elements, classification, properties. Recovered from: lifeder.com.