The unit circle is a circle of radius equal to 1, which is usually centered at the point (0,0) of the Cartesian coordinate system xy. Used to easily define the trigonometric ratios of angles using right triangles.
The equation of the unit circle centered at the origin is:
xtwo + Ytwo = 1
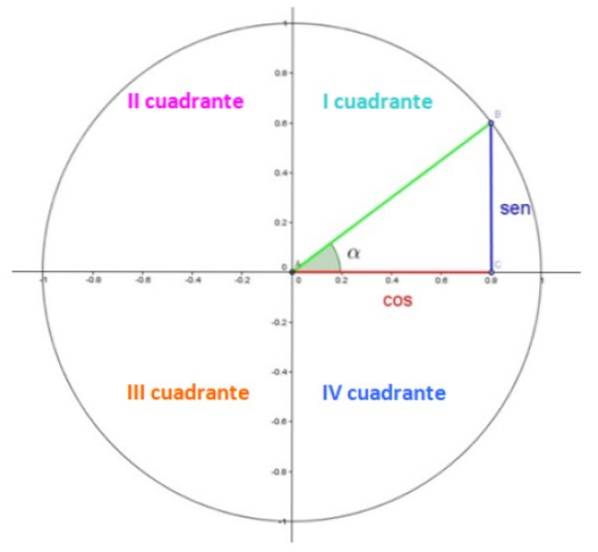
In figure 1 we have the unit circle, in which each quarter is in a quadrant. Quadrants are numbered with Roman numerals and counted counterclockwise.
In the first quadrant there is a triangle. The legs, in red and blue, measure respectively 0.8 and 0.6, while the hypotenuse in green measures 1, since it is a radius.
The acute angle α is a central angle in standard position, which means that its vertex coincides with the point (0,0) and its initial side with the positive x axis. The angle is measured counterclockwise and is assigned a positive sign by convention.
Well, in the unit circle, the cosine and sine coordinates of α are respectively the x and y coordinates of point B, which in the example shown are 0.8 and 0.6.
From these two they are defined:
Article index
If we limit ourselves to right triangles, the trigonometric ratios would apply only to acute angles. However, with the help of the unit circle, the calculation of the trigonometric ratios is extended to any angle α.
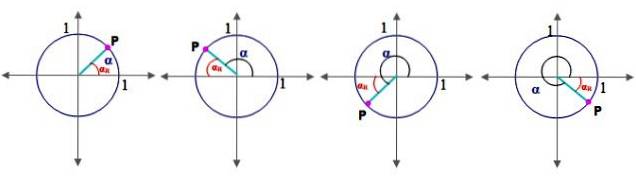
For this, it is necessary to first define the concept of reference angle αR:
Let α be an angle in standard position (the one whose starting side coincides with the positive x-axis), its reference angle αR is between his terminal side and the x-axis. Figure 2 shows the reference angle for angles in I, II, III and IV quadrant.
For each quadrant, the reference angle is calculated like this:
-First quadrant: αR = α
-Second quadrant: αR = 180º - α
-Third quadrant: αR = α - 180º
-Fourth quadrant: αR = 360º - α
Note that the first quadrant the angle α coincides with its reference angle. Well, the trigonometric ratios of the angle α are the same as their reference angle, with the signs according to those of the quadrants in which the terminal side of α falls..
In other words, the trigonometric cosine and sine ratios of the angle α coincide with the coordinates of the point P, according to figure 2.
In the following figure we see the trigonometric ratios of some notable angles, as deduced from the unit circle.
The cosine and sine ratios of any angle in the I quadrant are all positive. For α = 60º we have the coordinates (1/2; √3 / 2), which correspond respectively to cos 60º and sin 60º.
The coordinates of α = 120º are (-1/2; √3 / 2), since being in the second quadrant, the x coordinate is negative.
With the help of the unit circle and the coordinates of the points P on it, it is possible to draw the graphs of the functions cos t and sin t, as we will see below.
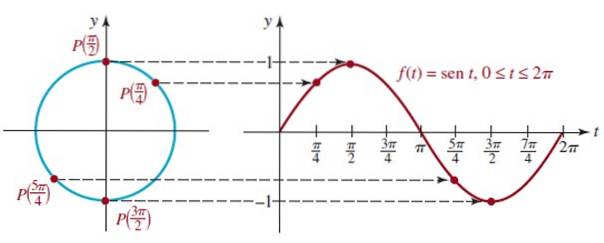
To do this, various positions of the point P (t) are located on the unit circle. We will start with the graph of the function f (t) = sin t.
We can see that when we go from t = 0 to t = π / 2 (90º) the value of sin t increases until it reaches 1, which is the maximum value.
On the other hand, from t = π / 2 to t = 3π / 2 the value of sin t decreases from 1, passing through 0 at t = π until it reaches its minimum of -1 at t = 3π / 2.
The figure shows the graph of the first cycle of f (t) = sin t that corresponds to the first round of the unit circle, this function is periodic with period 2π.
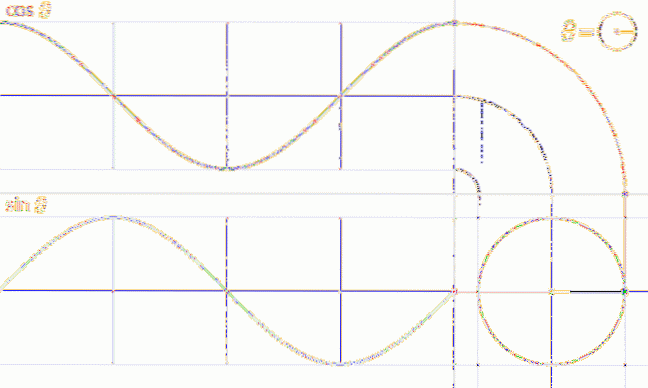
An analogous procedure can be carried out to obtain the graph of the function f (t) = cos t, as shown in the following animation:
-Both functions are continuous in the set of real numbers and also periodic, of period 2π.
-The domain of the functions f (t) = sin t and f (t) = cos t are all real numbers: (-∞, ∞).
-For the range or path of sine and cosine we have the interval [-1,1]. The brackets indicate that -1 and 1 are included.
- The zeros of sin t are the values that correspond to nπ with n integer, while the zeros of cos t are [(2n + 1) / 2] with n also integer.
-The function f (t) = sin t is odd, it has symmetry about the origin while the function cos t is even, its symmetry is about the vertical axis.
Given cos t = - 2/5, which is the horizontal coordinate of the point P (t) on the unit circle in the second quadrant, obtain the corresponding vertical coordinate sin t.
Since P (t) belongs to the unit circle, in which it is true that:
xtwo + Ytwo = 1
Therefore:
y = ± √ 1 - xtwo
Since P (t) is in the second quadrant, the positive value will be taken. The vertical coordinate of point P (t) is y:
y = √ 1 - (-2/5)two = √0.84
A mathematical model for temperature T in degrees Fahrenheit on any given day, t hours after midnight, it is given by:
T (t) = 50 + 10 sin [(π / 12) × (t - 8)]
With t between 0 and 24 hours. Find:
a) The temperature at 8 am.
b) Hours during which T (t) = 60ºF
c) Maximum and minimum temperatures.
We substitute t = 8 in the given function:
T (8) = 50 + 10 sin [(π / 12) × (t-8)] = 50 + 10 sin [(π / 12) × (8-8)] =
= 50 + 10 x sin 0 = 50 ºF
50 + 10 sin [(π / 12) × (t-8)] = 60
It is a trigonometric equation and we must solve for the unknown "t":
10 sin [(π / 12) × (t-8)] = 60 - 50 = 10
sin [(π / 12) × (t-8)] = 1
We know that sin π / 2 = 1, therefore the argument of the sine has to be 1:
(π / 12) × (t-8) = π / 2
t-8 = 6
t = 14 h
It is concluded that at 14 hours after midnight the temperature is 60º, that is, 2 pm. There is no other time throughout the day (24 hours) when this happens.
The maximum temperature corresponds to the value at which sin [(π / 12) × (t-8)] = 1 and is 60ºF. On the other hand, the minimum occurs if sin [(π / 12) × (t-8)] = -1 and is 40ºF.
Yet No Comments