The main difference between trajectory and displacement is that the latter is the distance and direction traveled by an object, while the former is the path or shape that the movement of that object takes.
However, to see more clearly the differences between displacement and trajectory, it is better to specify its conceptualization through examples that allow a better understanding of both terms..
It is understood as the distance and direction traveled by an object taking into account its initial position and its final position, always in a straight line. For its calculation, as it is a vector magnitude, the measurements of length known as centimeters, meters or kilometers are used..
The formula to calculate the displacement is defined as follows:
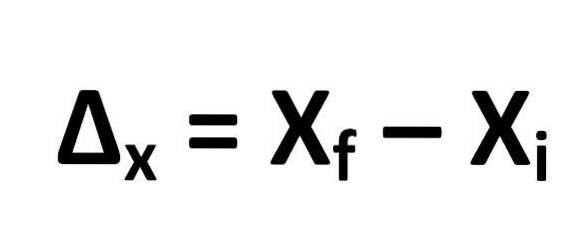
From which it follows that:
1- If a group of children are at the beginning of a route, whose initial position is 50m, moving in a straight line, determine the displacement at each of the points XF .
2- The data of the problem are extracted by substituting the values of Xtwo and X1 in the offset formula:
3- In this first approach we say that Δx is equal to 120m, which corresponds to the first value we find of XF, minus 50m which is the value of Xi, The result is 70m, that is, when reaching 120m traveled, the displacement was 70m to the right.
4- We proceed to solve in the same way for the values of b, c and d
In this case, the displacement gave us negative, that means that the final position is in the opposite direction to the initial position.
It is the route or line determined by an object during its movement and its evaluation in the International System, generally adopts geometric shapes such as the line, parabola, circle or ellipse). It is identified through an imaginary line and because it is a scalar quantity it is measured in meters.
It should be noted that to calculate the trajectory we must know if the body is at rest or movement, that is, it is subjected to the reference system that we select.
The equation to calculate the trajectory of an object in the International System is given by:
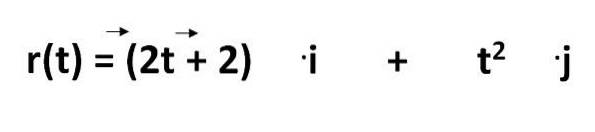
Of which we have to:
To understand the calculation of the path traveled by an object, we are going to develop the following example:
First step: As a path equation is a function of X, to do this define the values of X and Y respectively in each of the proposed vectors:
1- Solve the first position vector:
2- Ty = f (x), where X is given by the content of the unit vector .i and Y is given by the content of the unit vector .j:
3- y = f (x), that is, time is not part of the expression therefore we must solve it, we have:
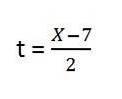
4- We substitute the clearance in Y. It remains:
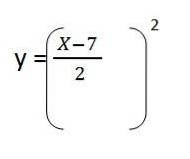
5- We solve the content of the parentheses and we have the equation of the resulting path for the first unit vector:
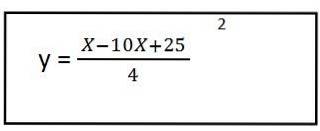
As we can see, it gave us a second degree equation, this means that the trajectory has the shape of a parabola.
Second step: We proceed in the same way to calculate the trajectory of the second unit vector
r (t) = (t - 2) .i + 2t .j
2- Following the steps that we saw previously y = f (x), we must clear the time because it is not part of the expression, we are left with:
3- We substitute the clearance in Y, remaining:
4- Solving the parentheses we have the equation of the resulting trajectory for the second unit vector:
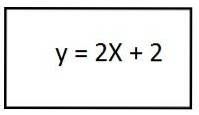
In this procedure the result was a straight line, which tells us that the trajectory has a rectilinear shape.
Once the concepts of displacement and trajectory are understood, we can deduce the rest of the differences that exist between both terms..
It is the path or line determined by an object during its movement. Adopts geometric shapes (straight, parabolic, circular or elliptical).
Yet No Comments