Redox balancing method steps, examples, exercises

The redox balancing method It is the one that allows balancing the chemical equations of redox reactions, which would otherwise be a headache. Here one or more species exchange electrons; the one that donates or loses them is called the oxidizing species, while the one that accepts or gains them, the reducing species.

In this method it is essential to know the oxidation numbers of these species, since they reveal how many electrons they have gained or lost per mole. Thanks to this, it is possible to balance the electric charges by writing the electrons in the equations as if they were reactants or products..

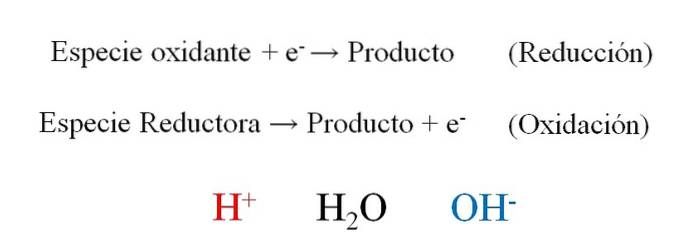
The image above shows how effectively electrons, and^- they are placed as reactants when the oxidizing species gains them; and as products when the reducing species loses them. Note that to balance these types of equations it is necessary to master the concepts of oxidation and oxidation-reduction numbers..

The species H⁺, H_twoO and OH^-, Depending on the pH of the reaction medium, it allows redox balancing, which is why it is very common to find them in exercises. If the medium is acidic, we turn to the H⁺; but if on the contrary the medium is basic, then we use the OH^- for rocking.

The nature of the reaction itself dictates what the pH of the medium should be. That is why, although it can be balanced assuming an acidic or basic medium, the final balanced equation will indicate whether the H ions are really dispensable or not.⁺ and OH^-.

Article index

• 1 Steps
  • 1.1 - General
  • 1.2 - Balancing in an acid medium
  • 1.3 - Balancing in basic medium
• 2 Examples
• 3 Exercises
  • 3.1 Exercise 1
  • 3.2 Exercise 2
• 4 References

Steps

- General

Check the oxidation numbers of the reactants and products

Assume the following chemical equation:

Cu (s) + AgNO₃(ac) → Cu (NO₃)_two + Ag (s)

This corresponds to a redox reaction, in which a change occurs in the oxidation numbers of the reactants:

Cu⁰(s) + Ag⁺NOT₃(ac) → Cu^two+(NOT₃)_two + Ag (s)⁰

Identify the oxidizing and reducing species

The oxidizing species gains electrons by oxidizing the reducing species. Therefore, its oxidation number decreases: it becomes less positive. Meanwhile, the oxidation number of the reducing species increases, since it loses electrons: it becomes more positive..

Thus, in the previous reaction, copper is oxidized, since it passes from Cu⁰ to Cu^two+; and silver is reduced, as it passes from Ag⁺ to Ag⁰. Copper is the reducing species, and silver the oxidizing species.

Write the half-reactions and balance atoms and charges

Identifying which species gain or lose electrons, the redox half-reactions are written for both the reduction and oxidation reactions:

Cu⁰ → Cu^two+

Ag⁺ → Ag⁰

Copper loses two electrons, while silver gains one. We place the electrons in both half-reactions:

Cu⁰ → Cu^two+ + 2e^-

Ag⁺ + and^- → Ag⁰

Note that the loads remain balanced in both half-reactions; but if they were added together, the law of conservation of matter would be violated: the number of electrons must be equal in the two half-reactions. Therefore, the second equation is multiplied by 2 and the two equations are added:

(Cu⁰ → Cu^two+ + 2e^-) x 1

(Ag⁺ + and^-  → Ag⁰) x 2 

Cu⁰ + 2Ag⁺ + 2e^- → Cu^two+ + 2Ag⁰ + 2e^-

The electrons cancel out because they are on the sides of the reactants and products:

Cu⁰ + 2Ag⁺ → Cu^two+ + 2Ag⁰

This is the global ionic equation.

Substitute coefficients from the ionic equation into the general equation

Finally, the stoichiometric coefficients from the previous equation are transferred to the first equation:

Cu (s) + 2AgNO₃(ac) → Cu (NO₃)_two + 2Ag (s)

Note that 2 was positioned with AgNO₃ because in this salt the silver is like Ag⁺, and the same happens with Cu (NO₃)_two. If this equation is not balanced at the end, we proceed to carry out the trial.

The equation proposed in the previous steps could have been balanced directly by trial and error. However, there are redox reactions that require an acid medium (H⁺) or basic (OH^-) to take place. When this happens, it cannot be balanced assuming the medium is neutral; as just shown (no H⁺ and neither OH^-).

On the other hand, it is convenient to know that the atoms, ions or compounds (mostly oxides) in which the changes in oxidation numbers occur are written in the half-reactions. This will be highlighted in the exercises section.

- Balance in acid medium

When the medium is acid, it is necessary to stop at the two half-reactions. This time when balancing we ignore the oxygen and hydrogen atoms, and also the electrons. Electrons will balance in the end.

Then, on the side of the reaction with fewer oxygen atoms, we add water molecules to make up for it. On the other side, we balance the hydrogens with H ions⁺. And finally, we add the electrons and proceed by following the general steps already exposed.

- Balance in basic medium

When the medium is basic, one proceeds in the same way as in the acidic medium with a small difference: this time on the side where there are more oxygen, a number of water molecules equal to this excess oxygen will be located; and on the other side, OH ions^- to compensate for hydrogens.

Finally, the electrons are balanced, the two half-reactions are added, and the coefficients of the global ionic equation are substituted in the general equation.

Examples

The following balanced and unbalanced redox equations serve as examples to see how much they change after applying this balancing method:

P₄ + ClO^- → PO₄^3- + Cl^- (unbalanced)

P₄ + 10 ClO^- + 6 h_twoO → 4 PO₄^3- + 10 Cl^- + 12 h⁺ (balanced acid medium)

P₄ + 10 ClO^- + 12 OH^- → 4 PO₄^3- + 10 Cl^- + 6 h_twoO (balanced medium basic)

I_two + KNO₃ → I^- + KIO₃ + NOT₃^- (unbalanced)

3I_two + KNO₃ + 3H_twoO → 5I^- + KIO₃ + NOT₃^- + 6H⁺ (balanced acid medium)

Cr_twoOR_two^7- + HNO_two → Cr³⁺ + NOT₃^- (unbalanced)

3HNO_two + 5H⁺ + Cr_twoOR_two^7- → 3NO₃^- +2Cr³⁺ + 4H_twoO (balanced acid medium)

Training

Exercise 1

Balance the following equation in basic medium:

I_two + KNO₃ → I^- + KIO₃ + NOT₃^-

General steps

We begin by writing the oxidation numbers of the species that we suspect have been oxidized or reduced; in this case, the iodine atoms:

I_two⁰ + KNO₃ → I^- + KI⁵⁺OR₃ + NOT₃^-

Note that iodine is oxidized and at the same time reduced, so we proceed to write their two respective half-reactions:

I_two → I^- (reduction, for every I^- 1 electron is consumed)

I_two  → IO₃^- (oxidation, for every IO₃^- 5 electrons are released)

In the oxidation half-reaction we place the anion IO₃^-, and not the iodine atom like I⁵⁺. We balance the iodine atoms:

I_two → 2I^-

I_two  → 2IO₃^-

Balance in basic medium

Now we focus on balancing the oxidation semi-reaction in a basic medium, since it has an oxygenated species. We add on the product side the same number of water molecules as there are oxygen atoms:

I_two  → 2IO₃^- + 6H_twoOR

And on the left side we balance the hydrogens with OH^-:

I_two  + 12OH^- → 2IO₃^- + 6H_twoOR

We write the two half-reactions and add the missing electrons to balance the negative charges:

I_two + 2e^- → 2I^-

I_two  + 12OH^- → 2IO₃^- + 6H_twoO + 10e^-

We equalize the numbers of the electrons in both half-reactions and add them:

(I_two + 2e^- → 2I^-) x 10

(I_two  + 12OH^- → 2IO₃^- + 6H_twoO + 10e^-) x 2

12I_two + 24 OH^- + 20e^- → 20I^- + 4IO₃^- + 12H_twoO + 20e^-

The electrons cancel out and we divide all coefficients by four to simplify the global ionic equation:

(12I_two + 24 OH^-  → 20I^- + 4IO₃^- + 12H_twoO) x ¼

3I_two + 6OH^- → 5I^- + IO₃^- + 3H_twoOR

And finally, we substitute the coefficients of the ionic equation in the first equation:

3I_two + 6OH^- + KNO₃ → 5I^- + KIO₃ + NOT₃^- + 3H_twoOR

The equation is already balanced. Compare this result with the balancing in acid medium of example 2.

Exercise 2

Balance the following equation in an acid medium:

Faith_twoOR₃ + CO → Fe + CO_two

General steps

We look at the oxidation numbers of iron and carbon to find out which of the two has been oxidized or reduced:

Faith_two³⁺OR₃ + C^two+O → Faith⁰ + C⁴⁺OR_two

Iron has been reduced, making it the oxidizing species. Meanwhile, the carbon has been oxidized, behaving as the reducing species. The half-reactions for oxidation and reduction concerned are:

Faith_two³⁺OR₃ → Faith⁰  (reduction, for each Fe 3 electrons are consumed)

CO → CO_two (oxidation, for every CO_two 2 electrons are released)

Note that we write the oxide, Fe_twoOR₃, because it contains faith³⁺, instead of just placing the Fe³⁺. We balance the atoms that are needed except those of oxygen:

Faith_twoOR₃ → 2Fe

CO → CO_two

And we proceed to carry out the balancing in an acid medium in both half-reactions, since there are oxygenated species in between..

Balance in acid medium

We add water to balance the oxygens, and then H⁺ to balance hydrogens:

Faith_twoOR₃ → 2Fe + 3H_twoOR

6H⁺ +  Faith_twoOR₃ → 2Fe + 3H_twoOR

CO + H_twoO → CO_two

CO + H_twoO → CO_two + 2H⁺

Now we balance the charges by placing the electrons involved in the half-reactions:

6H⁺ +  6e^- + Faith_twoOR₃ → 2Fe + 3H_twoOR

CO + H_twoO → CO_two + 2H⁺ + 2e^-

We equal the number of electrons in both half-reactions and add them:

(6H⁺ +  6e^- + Faith_twoOR₃ → 2Fe + 3H_twoO) x 2

(CO + H_twoO → CO_two + 2H⁺ + 2e^-) x 6

12 h⁺ + 12e^- + 2Fe_twoOR₃ + 6CO + 6H_twoO → 4Fe + 6H_twoO + 6CO_two + 12H⁺ + 12e^-

We cancel the electrons, the H ions⁺ and the water molecules:

2Fe_twoOR₃ + 6CO → 4Fe + 6CO_two

But these coefficients can be divided by two to simplify the equation even more, having:

Faith_twoOR₃ + 3CO → 2Fe + 3CO_two

This question arises: was redox balancing necessary for this equation? By trial and error it would have been much faster. This shows that this reaction proceeds regardless of the pH of the medium..

References

1. Whitten, Davis, Peck & Stanley. (2008). Chemistry. (8th ed.). CENGAGE Learning.
2. Helmenstine, Anne Marie, Ph.D. (September 22, 2019). How to Balance Redox Reactions. Recovered from: thoughtco.com
3. Ann Nguyen & Luvleen Brar. (June 05, 2019). Balancing Redox Reactions. Chemistry LibreTexts. Recovered from: chem.libretexts.org
4. Quimitube. (2012). Exercise 19: Adjustment of a redox reaction in basic medium with two oxidation half-reactions. Recovered from: quimitube.com
5. Washington University in St. Louis. (s.f.). Practice Problems: Redox Reactions. Recovered from: chemistry.wustl.edu
6. John Wiley & Sons. (2020). How to Balance Redox Equations. Recovered from: dummies.com
7. Rubén Darío O. G. (2015). Balancing chemical equations. Recovered from: aprendeenlinea.udea.edu.co