
Quota sampling method, advantages, disadvantages, examples
The quota sampling It is a non-probabilistic way of taking data from a sample by assigning quotas by strata. The quotas must be proportional to the fraction that this stratum represents with respect to the total population and the sum of the quotas must be equal to the size of the sample..
The researcher is the one who decides which groups or strata will be, for example he can divide a population into men and women. Another example of strata are age ranges, for example from 18 to 25, from 26 to 40 and from 40 onwards, which can be labeled as follows: youth, adults and seniors.

It is very convenient to know in advance what percentage of the total population represents each stratum. Then a statistically significant sample size is chosen, and proportional quotas are assigned to the percentage of each stratum with respect to the total population. The sum of the quotas per stratum must be equal to the total size of the sample.
Finally we proceed to take the data of the quotas assigned to each stratum, choosing the first elements that complete the quota.
It is precisely because of this non-random way of choosing the elements that this sampling method is considered non-probabilistic..
Article index
- 1 Steps to perform a quota sampling
- 1.1 Step 1
- 1.2 Step 2
- 1.3 Step 3
- 1.4 Step 4
- 1.5 Step 5
- 2 Case study
- 3 Applicability, advantages and disadvantages
- 3.1 Advantages
- 3.2 Disadvantages
- 4 Simple application example
- 4.1 Determination of quotas by age
- 4.2 Determination of quotas by age and sex
- 4.3 Difference with stratified random sampling
- 5 Proposed exercise
- 6 References
Steps to Perform Quota Sampling
Step 1
Segment the total population into strata or groups with some common characteristic. This characteristic will be previously decided by the statistical researcher conducting the study..
Step 2
Determine what percentage of the total population represents each of the strata or groups chosen in the previous step.
Step 3
Estimate a statistically significant sample size, according to the criteria and methodologies of statistical science..
Step 4
Calculate the number of elements or quotas for each stratum, so that they are proportional to the percentage that each one of them represents with respect to the total population and the total sample size..
Step 5
Take the data of the elements in each stratum until completing the quota corresponding to each stratum.
Practical case
Suppose you want to know the level of satisfaction with the metro service in a city. Previous studies on a population of 2000 people determined that 50% of users are youths between 16 and 21 years old, 40% are Adults between 21 and 55 years old and only 10% of users are greater over 55 years old.
Taking advantage of the results of this study, it is segmented or stratified according to the age of the users:
-Youths: fifty%
-Adults: 40%
-Greater: 10%
As there is a limited budget, the study has to be applied to a small sample, but one that is statistically significant. A sample size of 200 is chosen, that is, the survey on the level of satisfaction will be applied to 200 people in total.
It now remains to determine the quota or number of surveys for each segment or stratum, which must be proportional to the size of the sample and the percentage per stratum..
Quota per stratum
The quota for the number of surveys per stratum is as follows:
Youths: 200 * 50% = 200 * (50/100) = 100 surveys
Adults: 200 * 40% = 200 * (40/100) = 80 surveys
Greater: 200 * 10% = 200 * (10/100) = 20 surveys
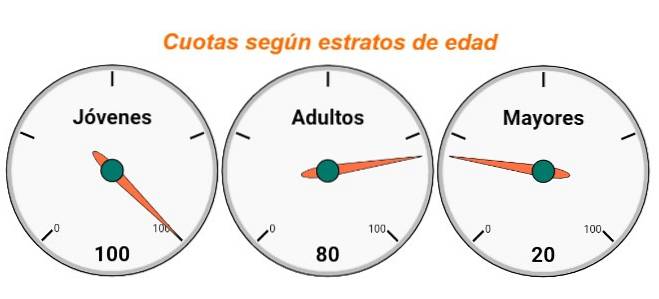
Note that the sum of the fees has to be equal to the sample size, that is, equal to the total number of surveys that will be applied. Then the surveys are passed until the quotas for each stratum are met.
It is important to note that this method is much better than taking all the surveys and passing them on to the first 200 people who appear, because according to previous data, it is very likely that the minority stratum will be left out of the study.
Applicability, advantages and disadvantages
For the method to be applicable, a criterion is required for the formation of the strata, which depends on the objective of the study..
Quota sampling is suitable when you want to know the preferences, differences or characteristics by sectors to direct specific campaigns according to the stratum or segment.
Its use is also useful when for some reason it is interesting to know the characteristics or interests of minority sectors, or when they do not want to leave them out of the study.
To be applicable, the weight or significance of each stratum with respect to the total population must be known. It is very important that this knowledge is reliable, otherwise erroneous results will be obtained.
Advantage
-Reduces study times, because quotas per stratum are usually small
-Simplify data analysis.
-Minimizes costs because the study is applied to small but well representative samples of the total population.
Disadvantages
-As the strata are defined a priori, it is possible that certain sectors of the population are left out of the study.
-By establishing a limited number of strata, it is possible that detail is being lost in the study.
-By obviating or incorporating as part of another some stratum, wrong conclusions can be drawn in the study.
-It makes it impossible to estimate the maximum sampling error.
Simple application example
You want to do a statistical study on the anxiety level in a population of 2000 people.
The researcher who directs the research intuits that differences in the results must be found depending on age and sex. Therefore, he decides to form three age strata denoted as follows: First_Age, Second_Age Y Third_Age. Regarding the segment sex the two usual types are defined: Male Y Feminine.
It defines First_Age, the one between 18 and 25 years old, Second_Age the one between 26 and 50 years old and finally Third_Age the one between 50 and 80 years.
Analyzing the data of the total population it is necessary to:
45% of the population belongs to the First_Age.
40% are in the Second_Age.
Finally, only 15% of the study population belongs to the Third_Age.
Using an appropriate methodology, which is not detailed here, a sample of 300 people is determined to be statistically significant.
Determination of quotas by age
The next step will then be to find the corresponding quotas for the segment Age, which is done as follows:
First_Age: 300 * 45% = 300 * 45/100 = 135
Second_Age: 300 * 40% = 300 * 40/100 = 120
Third_Age: 300 * 15% = 300 * 15/100 = 45
It is verified that the sum of the quotas gives the total size of the sample.
Determination of quotas by age and sex
So far the segment has not been taken into account sex of the population, of this segment two strata have already been defined: Feminine Y Male. Again we must analyze the data of the total population, which yield the following information:
-60% of the total population are of sex Feminine.
-Meanwhile, 40% of the population to be studied belongs to the sex Male.
It is important to note that the previous percentages regarding the distribution of the population according to sex do not take age into account..
Given that no more information is available, the assumption will be made that these proportions in terms of sex are equally distributed in the 3 strata of Age that have been defined for this study. With these considerations, we now proceed to establish the quotas by Age and Sex, which means that there will now be 6 sub-strata:
S1 = First_Age and Female: 135 * 60% = 135 * 60/100 = 81
S2 = First_Age and Male: 135 * 40% = 135 * 40/100 = 54
S3 = Second_Age and Female: 120 * 60% = 120 * 60/100 = 72
S4 = Second_Age and Male: 120 * 40% = 120 * 40/100 = 48
S5 = Third_Age and Female: 45 * 60% = 45 * 60/100 = 27
S6 = Third_Age and Male: 45 * 40% = 45 * 40/100 = 18
Application of surveys and study of the results
Once the six (6) segments and their corresponding quotas have been established, 300 surveys are prepared that will be applied according to the quotas already calculated..
The surveys will be applied as follows, 81 surveys are taken and the first 81 people who are in the segment are interviewed S1. Then it is done in the same way with the remaining five segments.
The study sequence is as follows:
-Analyze the survey results, which are then discussed, analyzing the results by segment.
-Make comparisons between the results by segment.
-Finally, develop hypotheses that explain the causes of these results..
Difference with stratified random sampling
In our example in which we apply sampling by quotas, the first thing to do is establish the quotas and then carry out the study. Of course, these quotas are not whimsical at all, because they have been based on previous statistical information on the total population..
If you do not have prior information on the study population, it is preferable to reverse the procedure, that is, first define the sample size and once the sample size has been established, proceed with the application of the survey in random way.
One way to ensure randomness would be to use a random number generator and poll employees whose employee number matches that of the random generator..
Once the data is available, and as the objective of the study is to see the anxiety levels according to the age and sex strata, the data is separated according to the six categories that we had previously defined. But without setting any prior fee.
It is for this reason that the method of stratified random sampling it is considered a probabilistic method. Meanwhile he quota sampling previously established no.
However, if the quotas are established with information based on population statistics, then it can be said that the method of quota sampling is roughly probabilistic.
Proposed exercise
The following exercise is proposed:
In a secondary school you want to do a survey on the preference between studying science or studying humanities.
Suppose the school has a total of 1000 students grouped into five levels according to the year of study. It is known that there are 350 students in the first year, 300 in the second, 200 in the third, 100 in the fourth and finally 50 in the fifth year. It is also known that 55% of the school's students are boys and 45% are girls..
Determine the strata and the quotas by stratum, in order to know the number of surveys to be applied according to the year of study and sex segments. Suppose further that the sample will be 10% of the total student population..
References
- Berenson, M. 1985. Statistics for Management and Economics, Concepts and Applications. Editorial Interamericana.
- Statistics. Quota sampling. Recovered from: encyclopediaeconomica.com.
- Statistics. Sampling. Recovered from: Estadistica.mat.uson.mx.
- Explorable. Quota sampling. Recovered from: explorable.com.
- Moore, D. 2005. Applied Basic Statistics. 2nd. Edition.
- Netquest. Probability sampling: stratified sampling. Recovered from: netquest.com.
- Wikipedia. Statistical sampling. Recovered from: en.wikipedia.org



Yet No Comments