
Complementary angles which and how are they calculated, examples, exercises

Two or more angles are complementary angles if the sum of its measurements corresponds to that of a right angle. As is known, the measure of a right angle in degrees is 90º, and in radians it is π / 2.
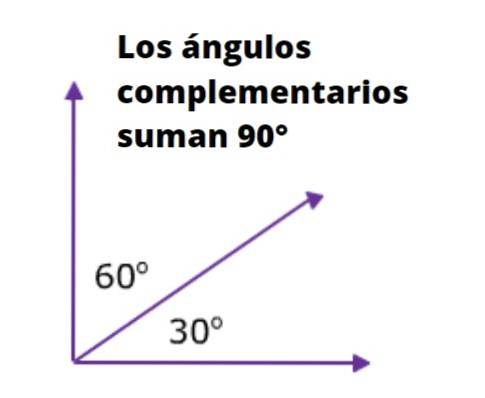
For example, the two angles adjacent to the hypotenuse of a right triangle are complementary to each other, since the sum of their measures is 90º. The following figure is very illustrative in this regard:
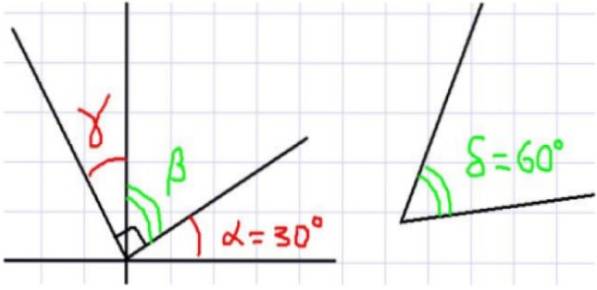
A total of four angles are shown in figure 1. α and β are complementary since they are adjacent and their sum completes a right angle. Similarly β is complementary to γ, from which it follows that γ and α are of equal measure.
Now, since the sum of α and δ is equal to 90 degrees, it can be stated that α and δ are complementary. Furthermore, since β and δ have the same complementary α, it can be stated that β and δ have the same measure.
Article index
- 1 Examples of complementary angles
- 1.1 - Examples A, B and C
- 1.2 - Examples D, E and F
- 2 Exercises
- 2.1 - Exercise 1
- 2.2 - Exercise 2
- 2.3 - Exercise 3
- 3 Angles of perpendicular sides
- 3.1 General rule for perpendicular side angles
- 4 References
Examples of complementary angles
In the following examples it is asked to find the unknown angles, marked with question marks in figure 2.

- Examples A, B and C
The following examples are in order of complexity.
Example A
In the figure above we have that the adjacent angles α and 40º add up to a right angle. That is, α + 40º = 90º, therefore α = 90º- 40º = 50º.
Example B
Since β is complementary to the angle of 35º, then β = 90º - 35º = 55º.
Example C
From figure 2C we have that the sum of γ + 15º + 15º = 90º. In other words, γ is complementary to the angle 30º = 15º + 15º. So that:
γ = 90º- 30º = 60º
- Examples D, E and F
In these examples there are more angles involved. To find the unknowns, the reader must apply the concept of complementary angle as many times as necessary.
Example D
Since X is complementary to 72º, it follows that X = 90º - 72º = 18º. Furthermore Y is complementary with X, so Y = 90º - 18º = 72º.
Finally Z is complementary with Y. From all the above it follows that:
Z = 90º - 72º = 18º
Example E
The angles δ and 2δ are complementary, therefore δ + 2δ = 90º.
That is, 3δ = 90º, which implies that δ = 90º / 3 = 30º.
Example F
If we call the angle between ω and 10º U, then U is supplementary to both of them, because it is observed that their sum completes a right angle. From which it follows that U = 80º. Since U is complementary with ω, then ω = 10º.
Training
Three exercises are proposed below. In all of them the value of the angles A and B in degrees must be found, so that the relationships shown in figure 3 are fulfilled.
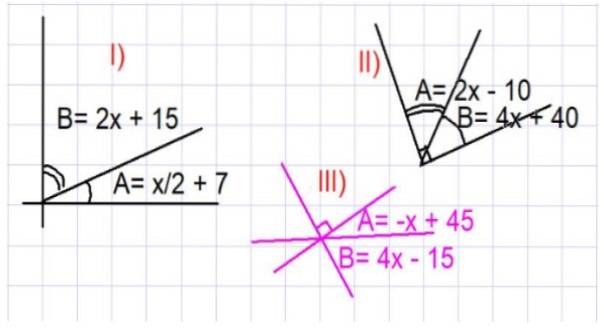
- Exercise 1
Determine the values of angles A and B from part I) of Figure 3.
Solution
From the figure shown it can be seen that A and B are complementary, therefore A + B = 90º. We substitute the expression for A and B as a function of x given in part I):
(x / 2 + 7) + (2x + 15) = 90
The terms are then grouped appropriately and a simple linear equation is obtained:
(5x / 2) + 22 = 90
Subtracting 22 in both members we have:
5x / 2 = 90 -22 = 68
And finally the value of x is cleared:
x = 2 * 68/5 = 136/5
Now the angle A is found by substituting the value of X:
A = (136/5) / 2 +7 = 103/5 = 20.6 º.
While angle B is:
B = 2 * 136/5 + 15 = 347 / 5º = 69.4º .
- Exercise 2
Find the values of the angles A and B of image II, figure 3.
Solution
Again, since A and B are complementary angles, we have: A + B = 90º. Substituting the expression for A and B as a function of x given in part II) of figure 3, we have:
(2x - 10) + (4x +40) = 90
Like terms are grouped together to obtain the equation:
6 x + 30 = 90
Dividing both members by 6 you get:
x + 5 = 15
From which it follows that x = 10º.
Therefore:
A = 2 * 10 - 10 = 10º
B = 4 * 10 + 40 = 80º.
- Exercise 3
Determine the values of angles A and B from part III) of Figure 3.
Solution
Again the figure is carefully analyzed to find the complementary angles. In this case we have that A + B = 90 degrees. Substituting the expression for A and B as a function of x given in the figure, we have:
(-x +45) + (4x -15) = 90
3 x + 30 = 90
Dividing both members by 3 results in the following:
x + 10 = 30
From which it follows that x = 20º.
In other words, the angle A = -20 +45 = 25º. And for its part: B = 4 * 20 -15 = 65º.
Perpendicular Side Angles
Two angles are said to be perpendicular sides if each side has its corresponding perpendicular on the other. The following figure clarifies the concept:
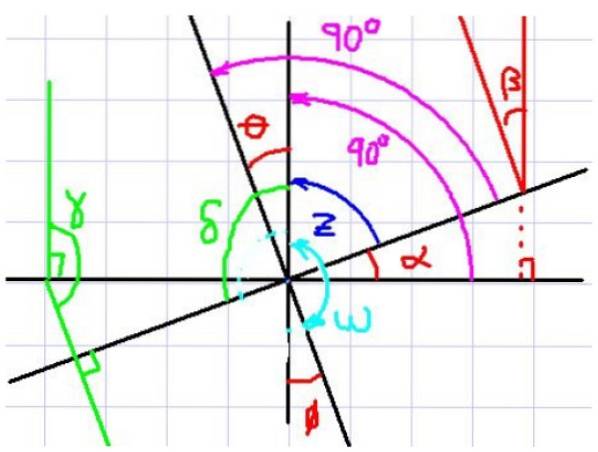
In figure 4 the angles α and θ are observed, for example. Now notice that each angle has its corresponding perpendicular to the other angle.
It is also seen that α and θ have the same complementary angle z, therefore the observer immediately concludes that α and θ have the same measure. It would seem then that if two angles have sides perpendicular to each other, they are equal, but let's look at another case.
Now consider the angles α and ω. These two angles also have corresponding perpendicular sides, however they cannot be said to be of equal measure, since one is acute and the other is obtuse..
Note that ω + θ = 180º. Furthermore θ = α. If you substitute this expression for z in the first equation you get:
δ + α = 180º, where δ and α are mutually perpendicular angles of sides.
Rule of thumb for angles of perpendicular sides
From the aforementioned, a rule can be established that is fulfilled as long as the angles have perpendicular sides:
If two angles have mutually perpendicular sides, then they are equal if both are acute or both are obtuse. Otherwise, if one is acute and the other is obtuse, then they are supplementary, that is, they add up to 180º.
Applying this rule and referring to the angles in figure 4 we can affirm the following:
α = β = θ = φ
γ = δ
With the supplementary angle ω of α, β, θ and φ.
References
- Baldor, J. A. 1973. Plane and space geometry. Central American Cultural.
- Mathematical laws and formulas. Angle measurement systems. Recovered from: ingemecanica.com.
- Wentworth, G. Plane Geometry. Recovered from: gutenberg.org.
- Wikipedia. Complementary angles. Recovered from: es.wikipedia.com
- Wikipedia. Conveyor. Recovered from: es.wikipedia.com
- Zapata F. Goniómetro: history, parts, operation. Recovered from: lifeder.com


Yet No Comments