
Perimeter of the circle how to get it out and formulas, solved exercises

The circle perimeter is the set of points that form the outline of a circle and is also known as length of the circumference. It depends on the radius, since a larger circumference will obviously have a larger contour.
Be P the perimeter of a circle and R the radius of it, then we can calculate P with the following equation:
P = 2π.R

Where π is a real number (read “pi”) that is approximately 3.1416… The ellipsis is due to the fact that π has infinite decimal places. Therefore, when making calculations, it is necessary to round its value.
However, for most applications, it is enough to take the amount indicated here, or to use all the decimals that the calculator with which you are working returns..
If instead of having the radius, it is preferred to use the diameter D, which we know is twice the radius, the perimeter is expressed as follows:
P = π.2R = π.D
As the perimeter is a length, it must always be expressed in units such as meters, centimeters, feet, inches and more, depending on the system that is preferred..
Article index
- 1 Circumferences and circles
- 2 Demonstration exercises for calculating the perimeter of the circle
- 2.1 - Exercise 1
- 2.2 - Exercise 2
- 2.3 - Exercise 3
- 3 Applications
- 4 References
Circumferences and circles
They are often terms that are used interchangeably, that is, synonymously. But it happens that there are differences between them.
The word "perimeter" comes from the Greek "peri" which means contour and "meter" or measure. The circumference is the outline or perimeter of the circle. Formally it is defined as follows:
A circle is the set of points with equal distance to a point called the center, this distance being the radius of the circumference.
For its part, the circle is defined as follows:
A circle is the set of points whose distance to a point called the center is less than or equal at a fixed distance called radio.
The reader can see the subtle difference between the two concepts. The circumference only refers to the set of points of the edge, while the circle is the set of points from the edge to the interior, of which the circumference is the border.
Exercises dedemonstration of calculating the perimeter of the circle
Through the following exercises, the concepts described above will be put into practice, as well as some others that will be explained as they appear. We will start from the simplest and the degree of difficulty will progressively increase.
- Exercise 1
Find the perimeter and area of the circle with radius 5 cm.
Solution
The equation given at the beginning is applied directly:
P = 2π.R= 2π.5 cm = 10 π cm = 31.416 cm
To calculate the area TO the following formula is used:
TO = π.Rtwo = π. (5cm)two= 25π cmtwo= 78.534 cmtwo
- Exercise 2
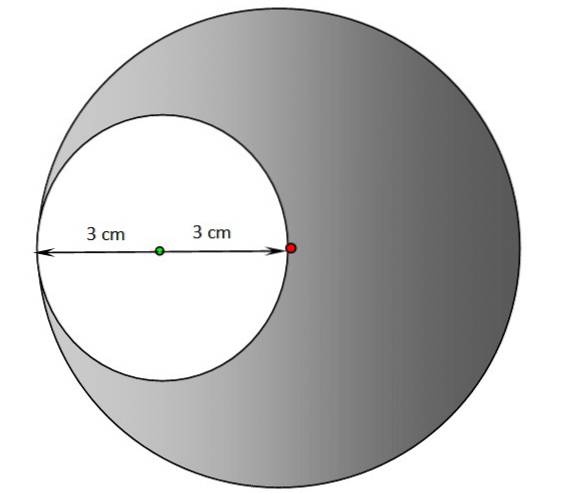
a) Find the perimeter and area of the blank region in the following figure. The center of the shaded circle is at the red point, while the center of the white circle is the green point.
b) Repeat the previous section for the shaded region.
Solution
a) The radius of the white circle is 3 cm, therefore we apply the same equations as in exercise 1:
P = 2π.R= 2π.3 cm = 6 π cm = 18.85 cm
TO = π.Rtwo = π. (3cm)two= 9π cmtwo= 28.27 cmtwo
b) For the shaded circle, the radius is 6 cm, its perimeter is double that calculated in section a):
P = 2π.R= 2π.6 cm = 12 π cm = 37.70 cm
And finally the area of the shaded region is calculated as follows:
- First we find the area of the shaded circle as if it were complete, which we will call A ', like this:
TO' = π.Rtwo= π. (6 cm)two = 36π cmtwo= 113.10 cmtwo
- Then to the area TO' The area of the white circle is subtracted, previously calculated in section a), in this way the requested area is obtained, which will be simply denoted as A:
A = A '- 28.27 cmtwo = 113.10-28.27 cmtwo = 84.83 cmtwo
- Exercise 3
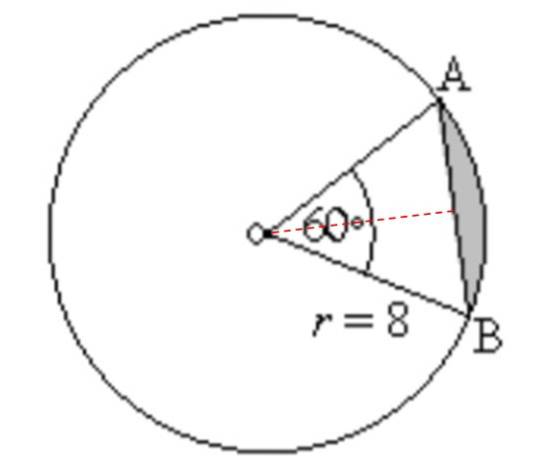
Find the area and perimeter of the shaded region in the following figure:
Solution
Calculation of the area of the shaded region
We first calculate the area of circular sector or wedge, between the straight segments OA and OB and the circular segment AB, as shown in the following figure:
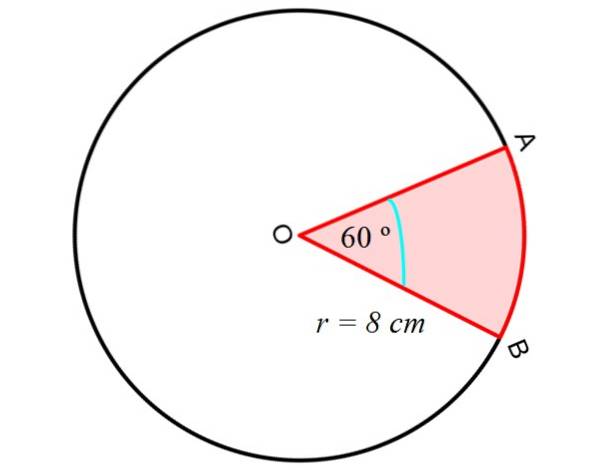
For this, the following equation is used, which gives us the area of a circular sector, knowing the radius R and the central angle between the segments OA and OB, that is, two of the radii of the circumference:
TO circular sector = Π.Rtwo. (αº / 360º)
Where αº is the central angle - it is central because its vertex is the center of the circumference - between two radii.
Step 1: calculate the area of the circular sector
Thus, the area of the sector shown in the figure is:
TO circular sector = Π.Rtwo. (αº / 360º) = π. (8 cm)two. (60º / 360º) = (64/6) π cmtwo= 33.51 cmtwo
Step 2: calculating the area of the triangle
Next we will calculate the area of the white triangle in figure 3. This triangle is equilateral and its area is:
TO triangle = (1/2) base x height
The height is the red dotted line seen in figure 4. To find it you can use the Pythagorean theorem, for example. But it's not the only way.
The observant reader will have noticed that the equilateral triangle is divided into two identical right triangles, whose base is 4 cm:
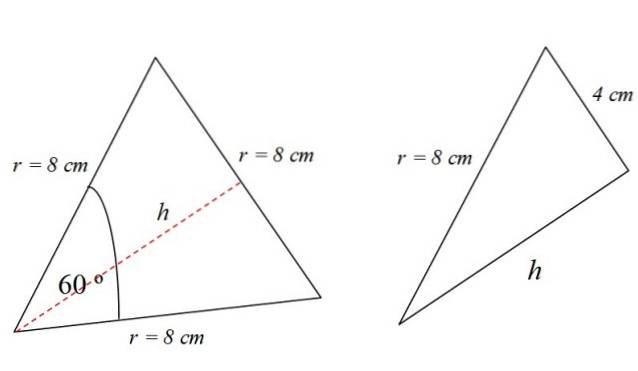
In a right triangle, the Pythagorean theorem is fulfilled, therefore:

TO triangle = (1/2) base x height = (1/2) 8 cm x 6.93 cm = 27.71 cmtwo.
Step 3: calculating the shaded area
Simply subtract the larger area (that of the circular sector) from the smaller area (that of the equilateral triangle): A shaded region = 33.51 cmtwo - 27.71 cmtwo = 5.80 cmtwo.
Calculation of the perimeter of the shaded region
The perimeter sought is the sum of the rectilinear side of 8 cm and the arc of circumference AB. Now, the complete circumference subtends 360º, therefore an arc that subtends 60º is one sixth of the complete length, which we know to be 2.π.R:
AB = 2.π.R / 6 = 2.π.8 cm / 6 = 8.38 cm
Substituting, the perimeter of the shaded region is:
P = 8 cm + 8.38 cm = 16.38 cm.
Applications
The perimeter, like the area, is a very important concept in geometry and with many applications in daily life..
Artists, designers, architects, engineers and many other people make use of the perimeter while developing their work, especially that of a circle, since the round shape is everywhere: from advertising, through food to machinery.

To directly know the length of a circumference, it is enough to wrap it with a thread or string, then extend this thread and measure it with a tape measure. The other alternative is to measure the radius or diameter of the circle and use one of the formulas described above..
In daily work, the concept of perimeter is used when:
-The right mold is chosen for a certain size of pizza or cake.
-An urban road is going to be designed, by calculating the size of a vial where cars can turn to change direction.
-We know that the Earth revolves around the Sun in a roughly circular orbit - planetary orbits are actually elliptical, according to Kepler's laws - but the circumference is a very good approximation for most planets..
-The appropriate size of a ring is chosen to be bought in an online store.
-We choose a wrench of the right size to loosen a nut.
And many more.
References
- Free Mathematics Tutorials. Area and Perimeter of a Circle - Geometry Calculator. Recovered from: analyzemath.com.
- Math Open Reference. Circumference, Perimeter of a circle. Recovered from: mathopenref.com.
- Monterey Institute. Perimeter and Area. Recovered from: montereyinstitute.org.
- Sciencing. How to find the Perimeter of a Circle. Recovered from: sciencing.com.
- Wikipedia. Circumference. Recovered from: en.wikipedia.org.


Yet No Comments