
What is a polygonal graph? (with examples)

A polygonal graph It is a line graph typically used by statistics to compare data and represent the magnitude or frequency of certain variables. In other words, a polygonal graph is one that can be found in a Cartesian plane, where two variables are related and the points marked between them are joined to form a continuous and irregular line..
A polygon plot serves the same purpose as a histogram, but is particularly useful for comparing groups of data. Also, it is a good alternative to show cumulative frequency distributions.
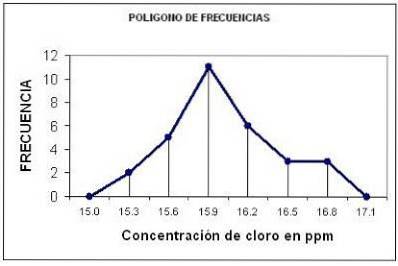
In this sense, the term frequency is understood as the number of times an event takes place within a sample.
All polygon graphs are initially structured as histograms. In this way, an axis in X (horizontal) and a axis in Y (vertical) are marked.
Also, variables with their respective intervals and frequencies are chosen to measure said intervals. Usually, the variables are plotted in the X plane and the frequencies in the Y plane..
Once the variables and frequencies have been established on the X and Y axes, we proceed to mark the points that relate them within the plane.
These points are subsequently joined, forming a continuous and irregular line known as a polygonal graph (Education, 2017).
Polygon graph function
The main function of a polygonal graph is to indicate the changes undergone by a phenomenon within a defined period of time or in relation to another phenomenon known as frequency.
In this way, it is a useful tool to compare the state of variables over time or in contrast to other factors (Lane, 2017).
Some common examples that can be evidenced in daily life include the analysis of the variation in prices of certain products over the years, the change in body weight, the increase in the minimum wage of a country, and in general.
In general terms, a polygonal graph is used when you want to visually represent the variation of a phenomenon over time, in order to be able to establish quantitative comparisons of it.
This graph is derived in many cases from a histogram in that the points that are marked on the Cartesian plane correspond to those that span the bars of the histogram.
Graphic representation
Unlike the histogram, the polygon graph does not use bars of different heights to mark the change of the variables within a defined time.
The graph uses line segments that rise or fall within the Cartesian plane, depending on the value given to the points that mark the change in the behavior of the variables on both the X and Y axes..
Thanks to this particularity, the polygonal graph receives its name, since the figure resulting from the union of the points with line segments within the Cartesian plane is a polygon with consecutive straight segments.
An important feature that must be taken into account when you want to represent a polygonal graph is that both the variables on the X axis and the frequencies on the Y axis must be marked with the title of what they are measuring..
In this way, the reading of the continuous quantitative variables included in the graph, it is possible.
On the other hand, to be able to make a polygonal graph, two intervals must be added at the ends, each of them of equal size and with a frequency equivalent to zero..
In this way, the highest and lowest limits of the analyzed variable are taken and each one is divided by two, to determine the place where the line of the polygonal graph should start and end (Xiwhanoki, 2012).
Finally, the location of the points on the graph will depend on the data previously available for both the variable and the frequency..
These data must be organized in pairs whose location within the Cartesian plane will be represented by a point. To form the polygon graph, the points must be joined in the direction from left to right
Examples of polygonal graphs
Example 1
In a group of 400 students, their height is expressed in the following table:
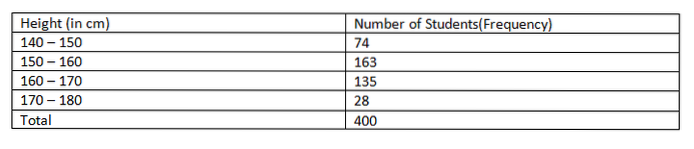
The polygon graph of this table would be the following:
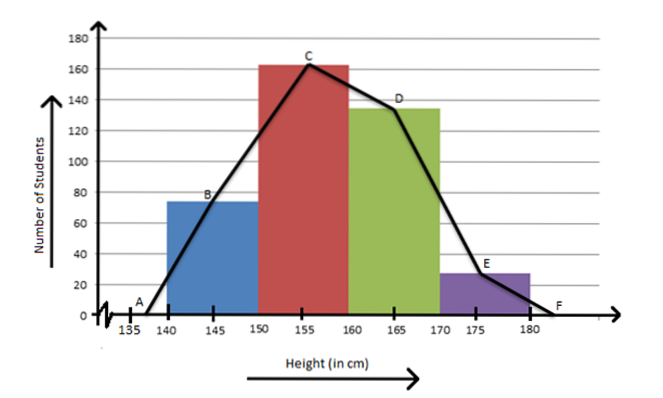
The stature of the students is represented on the X axis or horizontal axis on a scale defined in cm as its title indicates, whose value increases every five units.
On the other hand, the number of students is represented on the Y axis or vertical axis on a scale that increases its value every 20 units.
The rectangular bars within this graph correspond to those of a histogram. However, within the polygonal graph these bars are used to represent the width of the class interval covered by each variable, and their height marks the frequency corresponding to each of these intervals (ByJu's, 2016).
Example 2
In a group of 36 students, an analysis of their weight will be made according to the information collected in the following table:
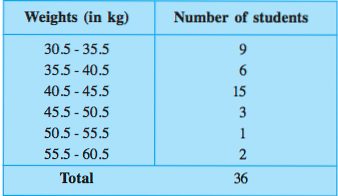
The polygon graph of this table would be the following:
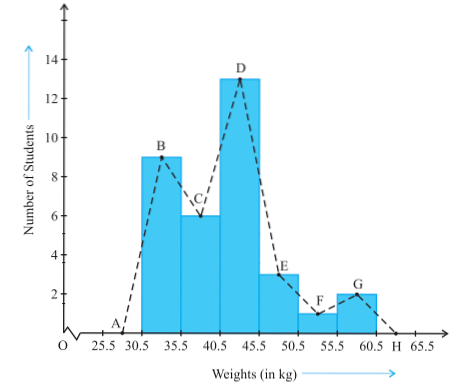
Within the X axis or horizontal axis the weights of the students are represented in kilograms. The class interval increases every 5 kilograms.
However, between zero and the first point of the interval, an irregularity in the plane has been marked to denote that this first space represents a value greater than 5 kilograms..
The vertical y-axis expresses the frequency, that is, the number of students, advancing on a scale whose number increases every two units.
This scale is established taking into account the values given in the table where the initial information was collected..
In this example, as in the previous one, the rectangles are used to mark the class intervals manifested in the table.
However, within the polygonal graph the relevant information is obtained from the line that results from joining the points resulting from the pair of related data in the table (Net, 2017).
References
- ByJu's. (August 11, 2016). ByJu's. Obtained from Frequency Polygons: byjus.com
- Education, M. H. (2017). Middle / High School Algebra, Geometry, and Statistics (AGS). In M. H. Education, Middle / High School Algebra, Geometry, and Statistics (AGS) (p. 48). Mcgraw hill.
- Lane, D. M. (2017). Rice University. Obtained from Frequency Polygons: onlinestatbook.com.
- Net, K. (2017). Kwiz Net. Retrieved from Middle / High School Algebra, Geometry, and Statistics (AGS): kwiznet.com.
- (September 1, 2012). Essay Club. Obtained from What is a polygonal graph ?: clubensayos.com.



Yet No Comments