
Hund's rule or principle of maximum multiplicity
The Hund's rule or principle of maximum multiplicity establishes, empirically, how the energy-degenerate orbital electrons must occupy. This rule, as its name alone suggests, came from the German physicist Friedrich Hund, in 1927, and since then it has been very useful in quantum and spectroscopic chemistry..
There are really three Hund's rules applied in quantum chemistry; However, the first one is the simplest for the basic understanding of how to electronically structure an atom.
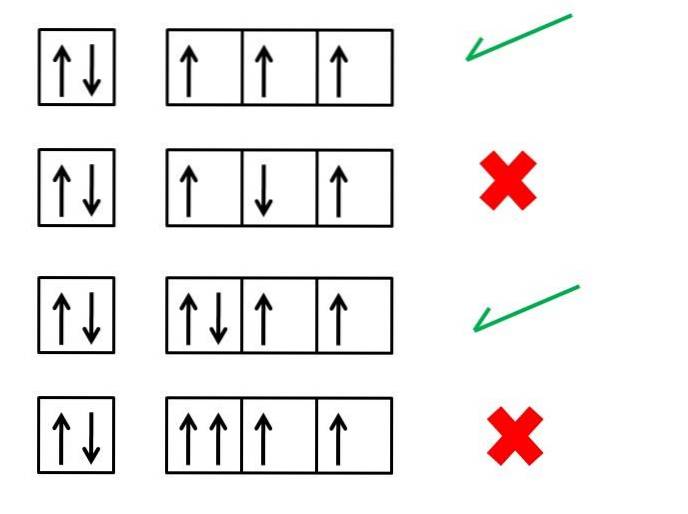
Hund's first rule, that of maximum multiplicity, is essential to understand the electronic configurations of the elements; establishes what should be the ordering of the electrons in the orbitals to generate an atom (ion or molecule) with greater stability.
For example, the image above shows four series of electron configurations; the boxes represent the orbitals, and the black arrows represent the electrons.
The first and third series correspond to correct ways to order the electrons, while the second and fourth series indicate how the electrons should not be placed in the orbitals.
Article index
- 1 Order of filling the orbitals according to Hund's rule
- 1.1 Pairing of spins
- 1.2 Parallel and antiparallel spins
- 2 Multiplicity
- 3 Exercises
- 3.1 Fluorine
- 3.2 Titanium
- 3.3 Iron
- 4 References
Orbital filling order according to Hund's rule
Although no mention is made of the other two Hund rules, correctly executing the fill order is implicitly applying these three rules at the same time.
What do the first and third series of orbitals in the image have in common? Why are they correct? For starters, each orbital can only “house” two electrons, which is why the first box is complete. The filling must therefore continue with the three boxes or orbitals on the right.
Spin mating
Each box in the first series has an arrow pointing upwards, which symbolizes three electrons with spins in the same direction. When pointing up, it means that their spins have a value of +1/2, and if they point down, their spins will have values of -1/2.
Note that the three electrons occupy different orbitals, but with unpaired spins.
In the third series, the sixth electron is located with a spin in the opposite direction, -1/2. This is not the case for the fourth series, where this electron enters the orbital with a spin of +1/2.
And so, the two electrons, like those of the first orbital, will have their paired spins (one with spin +1/2 and one with spin -1/2).
The fourth series of boxes or orbitals violates the Pauli exclusion principle, which states that no electron can have the same four quantum numbers. Hund's rule and Pauli's exclusion principle always go hand in hand.
Therefore, the arrows should be placed in such a way that they are unpaired until they occupy all the boxes; and immediately afterwards, they are completed with the arrows pointing in the opposite direction.
Parallel and antiparallel spins
It is not enough that electrons have their spins paired: they must also be parallel. This in the representation of boxes and arrows is guaranteed by placing the latter with their ends parallel to each other..
The second series presents the error that the electron in the third box meets its spin in an antiparallel sense with respect to the others..
Thus, it can be summarized that the ground state of an atom is one that obeys Hund's rules, and therefore has the most stable electronic structure.
The theoretical and experimental basis affirms that when an atom has electrons with a greater number of unpaired and parallel spins, it stabilizes as a result of an increase in electrostatic interactions between the nucleus and the electrons; increase that is due to the decrease of the shielding effect.
Multiplicity
The word 'multiplicity' was mentioned at the beginning, but what does it mean in this context? Hund's first rule establishes that the most stable ground state for an atom is the one with the highest number of spin multiplicity; in other words, the one that presents its orbitals with the highest number of unpaired electrons.
The formula to calculate the multiplicity of the spin is
2S + 1
Where S equals the number of unpaired electrons multiplied by 1/2. Thus, having several electronic structures with the same number of electrons, 2S + 1 can be estimated for each one and the one with the highest multiplicity value will be the most stable.
The multiplicity of the spin can be calculated for the first series of orbitals with three electrons with their spins unparalleled and parallel:
S = 3 (1/2) = 3/2
And the multiplicity then is
2 (3/2) + 1 = 4
This is Hund's first rule. The most stable configuration must also comply with other parameters, but which for chemical understanding purposes are not entirely necessary..
Training
Fluorine
Only the valence shell is considered, since it is assumed that the inner shell is already filled with electrons. The electron configuration of fluorine is therefore [He] 2stwo2 P5.
A 2s orbital must be filled first and then three p orbitals. To fill the 2s orbital with the two electrons, it is enough to place them in such a way that their spins are paired.
The other five electrons for the three 2p orbitals are arranged as illustrated below.

The red arrow represents the last electron to fill the orbitals. Note that the first three electrons that enter the 2p orbitals are placed unpaired and with their spins parallel.
Then, from the fourth electron, it begins to pair its spin -1/2 with the other electron. The fifth and last electron proceeds in the same way.
Titanium
The electron configuration of titanium is [Ar] 3dtwo4stwo. Since there are five d orbitals, it is suggested to start on the left side:
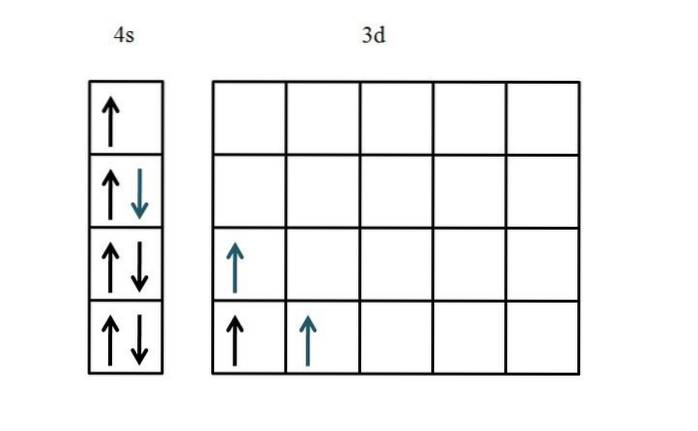
This time the filling of the 4s orbital was shown. As there are only two electrons in the 3d orbitals, there is almost no problem or confusion when placing them with their unpaired and parallel spins (blue arrows).
Iron
Another example, and finally, is iron, a metal that has more electrons in its d orbitals than titanium. Its electron configuration is [Ar] 3d64stwo.
If it were not for Hund's rule and the Pauli exclusion principle, we would not know how to arrange such six electrons in their five d orbitals..
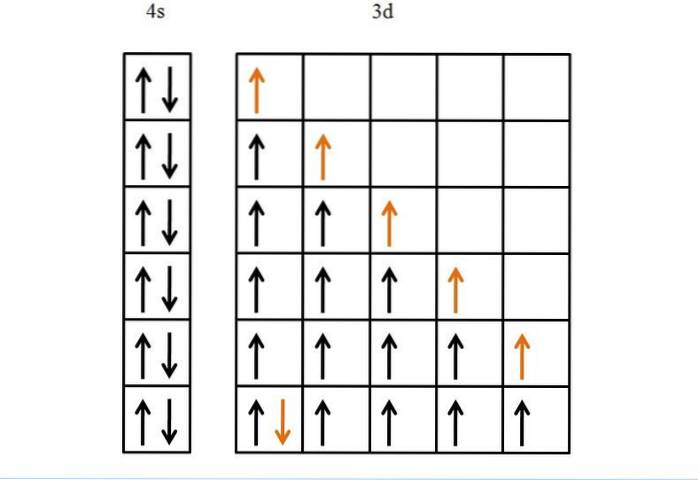
Although it may seem easy, without these rules many wrong possibilities could arise regarding the order of filling of the orbitals.
Thanks to these, the advance of the golden arrow is logical and monotonous, which is nothing more than the last electron that is being placed in the orbitals..
References
- Serway & Jewett. (2009). Physics: for science and engineering with Modern Physics. Volume 2. (Seventh edition). Cengage Learning.
- Glasstone. (1970). Textbook of physical chemistry. In Chemical kinetics. Second edition. D. Van Nostrand, Company, Inc.
- Méndez A. (March 21, 2012). Hund's rule. Recovered from: quimica.laguia2000.com
- Wikipedia. (2018). Hund's rule of maximum multiplicity. Recovered from: en.wikipedia.org
- Chemistry LibreTexts. (August 23, 2017). Hund's Rules. Recovered from: chem.libretexts.org
- Nave R. (2016). Hund's Rules. Recovered from: hyperphysics.phy-astr.gsu.edu



Yet No Comments