
Sturges Rule Explanation, Applications and Examples
The sturges rule it is a criterion used to determine the number of classes or intervals that are necessary to graphically represent a set of statistical data. This rule was enunciated in 1926 by the German mathematician Herbert Sturges.
Sturges proposed a simple method, based on the number of samples x that would allow us to find the number of classes and their range width. Sturges' rule is widely used, especially in the area of statistics, specifically to construct frequency histograms..
Article index
- 1 Explanation
- 2 Applications
- 3 Example
- 4 References
Explanation
Sturges' rule is an empirical method widely used in descriptive statistics to determine the number of classes that must exist in a frequency histogram, in order to classify a set of data representing a sample or population..
Basically, this rule determines the width of the graphic containers, of the frequency histograms.
To establish his rule Herbert Sturges considered an ideal frequency diagram, consisting of K intervals, where the i-th interval contains a certain number of samples (i = 0,… k - 1), represented as:
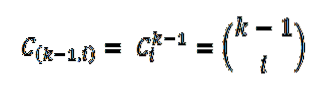
That number of samples is given by the number of ways in which a subset of a set can be extracted; that is, by the binomial coefficient, expressed as follows:
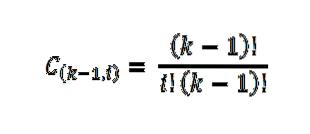
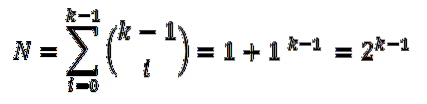
To simplify the expression, he applied the properties of logarithms to both parts of the equation:
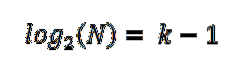
Thus, Sturges established that the optimal number of intervals k is given by the expression:

It can also be expressed as:

In this expression:
- k is the number of classes.
- N is the total number of observations in the sample.
- Log is the common logarithm of base 10.
For example, to construct a frequency histogram that expresses a random sample of the height of 142 children, the number of intervals or classes that the distribution will have is:
k = 1 + 3,322 * log10 (N)
k = 1 + 3,322* log (142)
k = 1 + 3,322* 2.1523
k = 8.14 ≈ 8
Thus, the distribution will be in 8 intervals.
The number of intervals must always be represented by whole numbers. In cases where the value is decimal, an approximation must be made to the nearest whole number.
Applications
Sturges's rule is applied mainly in statistics, since it allows a frequency distribution to be made through the calculation of the number of classes (k), as well as the length of each of these, also known as amplitude..
The amplitude is the difference of the upper and lower limit of the class, divided by the number of classes, and is expressed:

There are many rules of thumb that allow you to make a frequency distribution. However, Sturges' rule is commonly used because it approximates the number of classes, which generally ranges from 5 to 15..
Thus, it considers a value that adequately represents a sample or population; that is, the approximation does not represent extreme groupings, nor does it work with an excessive number of classes that do not allow the sample to be summarized..
Example
A frequency histogram needs to be made according to the data given, which correspond to ages obtained in a survey of men who exercise in a local gym..

To determine the intervals, one must know the size of the sample or the number of observations; in this case, we have 30.
Then the Sturges rule applies:
k = 1 + 3,322 * log10 (N)
k = 1 + 3,322* log (30)
k = 1 + 3,322* 1.4771
k = 5.90 ≈ 6 intervals.
From the number of intervals, the amplitude that these are going to have can be calculated; that is, the width of each bar represented in the frequency histogram:
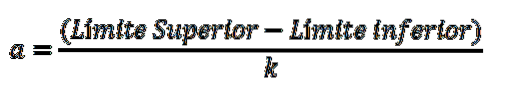
The lower limit is considered as the smallest value of the data, and the upper limit is the largest value. The difference between the upper and lower limits is called the range or range of the variable (R).
From the table we have that the upper limit is 46 and the lower limit is 13; that way, the amplitude of each class will be:
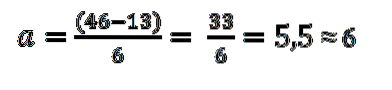
The intervals will be made up of an upper and a lower limit. To determine these intervals, we start by counting from the lower limit, adding to this the amplitude determined by rule (6), as follows:
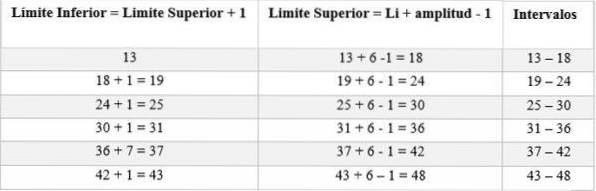
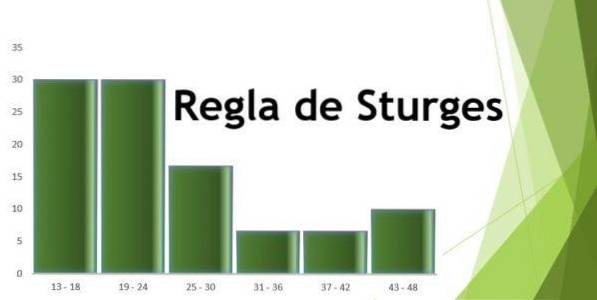
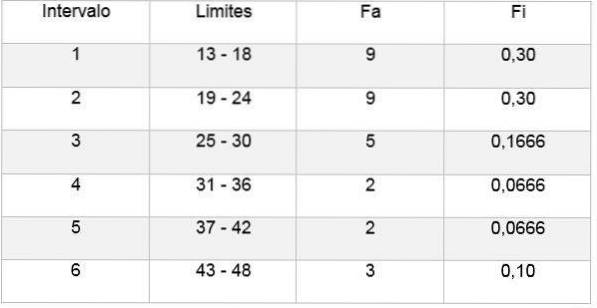
Then the absolute frequency is calculated to determine the number of men corresponding to each interval; in this case it is:
- Interval 1: 13 - 18 = 9
- Interval 2: 19 - 24 = 9
- Interval 3: 25 - 30 = 5
- Interval 4: 31 - 36 = 2
- Interval 5: 37 - 42 = 2
- Range 6: 43 - 48 = 3
When adding the absolute frequency of each class, this must be equal to the total number of the sample; in this case, 30.
Subsequently, the relative frequency of each interval is calculated, dividing its absolute frequency by the total number of observations:

- Interval 1: fi = 9 ÷ 30 = 0.30
- Interval 2: fi = 9 ÷ 30 = 0.30
- Interval 3: fi = 5 ÷ 30 = 0.1666
- Interval 4: fi = 2 ÷ 30 = 0.0666
- Interval 5: fi = 2 ÷ 30 = 0.0666
- Interval 4: fi = 3 ÷ 30 = 0.10
Then you can make a table that reflects the data, and also the diagram from the relative frequency in relation to the intervals obtained, as can be seen in the following images:
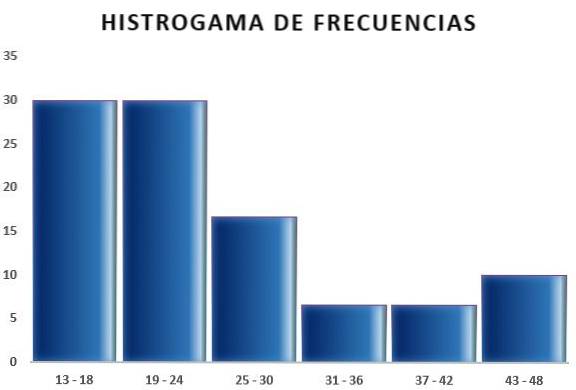
In this way, the Sturges rule allows determining the number of classes or intervals in which a sample can be divided, in order to summarize a data sample through the elaboration of tables and graphs..
References
- Alfonso Urquía, M. V. (2013). Modeling And Simulation Of Discrete Events. UNED,.
- Altman Naomi, M. K. (2015). "Simple Linear Regression." Nature Methods .
- Antúnez, R. J. (2014). Statistics in education. Digital UNIT.
- Fox, J. (1997.). Applied Regression Analysis, Linear Models, and Related Methods. SAGE Publications.
- Humberto Llinás Solano, C. R. (2005). Descriptive statistics and probability distributions. northern University.
- Panteleeva, O. V. (2005). Fundamentals of Probability and Statistics.
- O. Kuehl, M. O. (2001). Design of Experiments: Statistical Principles of Research Design and Analysis. Thomson Editors.



Yet No Comments