
Newton's second law applications, experiments and exercises
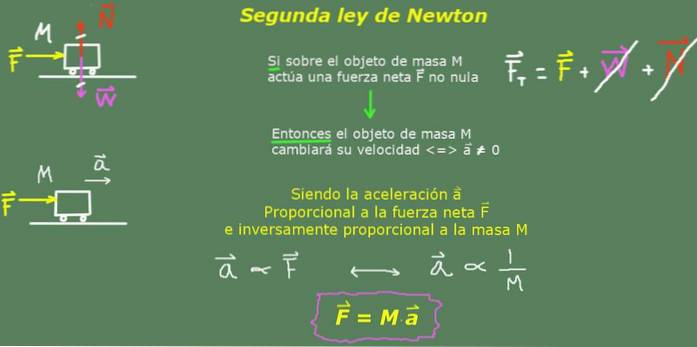
The second law of Newton or the fundamental law of dynamics establishes that if an object is subjected to a force or a set of forces that do not cancel, then the object will accelerate in the direction of the resultant force, this acceleration being proportional to the intensity of that net force and inversely proportional to the mass of the object.
Yes F is the net force, M the mass of the object and to the acceleration it acquires, then Newton's second law would be expressed mathematically like this: to = F / M or in the most usual way F = M∙to
Article index
- 1 Explanation and formulas
- 2 Solved exercises
- 2.1 Exercise 1
- 2.2 Exercise 2
- 3 Applications of Newton's Second Law
- 3.1 The acceleration of an elevator
- 3.2 The mayonnaise jar
- 4 Experiments for children
- 4.1 Experiment 1
- 4.2 Experiment 2
- 5 Articles of interest
- 6 References
Explanation and formulas
As explained above, the usual way to express the second law is with the formula:
F = M∙to
Both acceleration and force have to be measured from an inertial reference frame. Note that the mass is a positive quantity, so the acceleration points in the same direction as the resultant force.
Note also that when the resultant force is zero (F = 0) then the acceleration will also be zero ( to = 0 ) as long as M> 0. This result fully agrees with Newton's first law or law of inertia.
Newton's first law establishes inertial reference systems as those that move with constant velocity with respect to a free particle. In practice and for the purpose of the most common applications, a reference system fixed to the ground or any other that moves at a constant speed with respect to it, will be considered inertial..
Force is the mathematical expression of the object's interaction with the environment. The force can be a constant quantity or change with time, the position and the speed of the object..
The unit in the International System (SI) for force is the Newton (N). The mass in the (SI) is measured in (kg) and the acceleration in (m / stwo). One Newton of force is the force necessary to accelerate an object of mass 1 kg at 1 m / stwo .
Solved exercises
Exercise 1
An object of mass m is released from a certain height and a fall acceleration of 9.8 m / s² is measured.
The same happens with another object of mass m 'and another of mass m "and another and another. The result is always the acceleration of gravity which is denoted by g and is equal to 9.8 m / s². In these experiments the shape of the object and the value of its mass is such that the force due to air resistance is negligible.
It is asked to find a model for the earth's attractive force (known as weight) that is consistent with the experimental results..
Solution
We choose an inertial reference system (fixed with respect to the floor) with a positive direction of the vertical X axis and downwards.
The only force acting on the mass object m is the terrestrial attraction, that force is called the weight P, how it points down is positive.
The acceleration that the mass object acquires m once it is released it is a = g , pointed down and positive.
We propose Newton's second law
P = m a
What will be the model of P such that the acceleration predicted by the second law is g regardless of the value of m? : The only alternative is that P = m g whenever m> 0.
m g = m a from where we clear: a = g
We conclude that the weight, the force with which the Earth attracts an object will be the mass of the object multiplied by the acceleration of gravity and its direction is vertical and pointed down.
P = m∙g
Exercise 2
A block of 2 kg of mass rests on a completely flat and horizontal floor. If a force of 1 N is applied, what is the acceleration of the block and what speed will it have after 1 s?.
Solution
The first thing is to define an inertial coordinate system. One has been chosen with the X axis on the floor and the Y axis perpendicular to it. Then a force diagram is made, placing the forces due to the interactions of the block with its environment.

The force N represents the normal, it is the vertical upward force that the floor surface exerts on the block M. It is known that N exactly balances P because the block does not move in the vertical direction.
F is the horizontal force applied to block M, pointing in the positive direction of the X axis.
The net force is the sum of all the forces on the block of mass M. We make the vector sum of F, P and N. Since P and N are equal and opposite, they cancel each other, and the net force is F.
So the resulting acceleration will be the quotient of the net force and the mass:
a = F / M = 1 N / 2 kg = 0.5 m / s²
As the block starts from rest after 1s its velocity will have changed from 0 m / s to 0.5 m / s .
Applications of Newton's Second Law
Accelerating an elevator
A boy uses a bathroom scale to measure his weight. The value you get is 50 kg. Then the boy takes the weight to the elevator of his building, because he wants to measure the acceleration of the elevator. The results obtained when starting up are:
- The scale registers a weight of 58 kg for 1.5 s
- Then measure 50 kg again.
With these data, calculate the acceleration of the elevator and the speed it acquires.
Solution
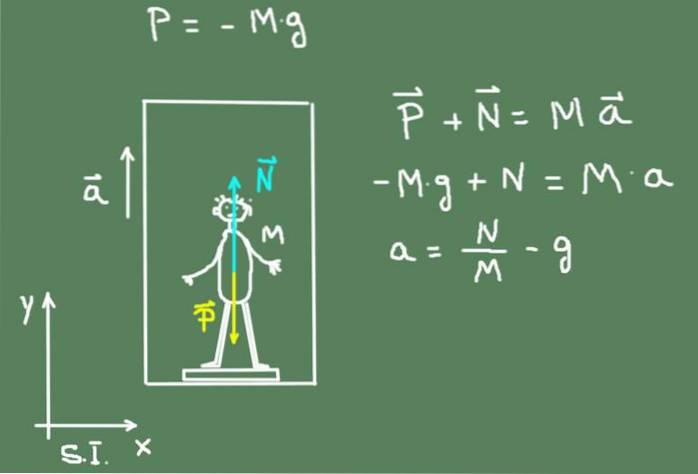
The scale measures weight in a unit called kilogram_force. By definition, the kilogram_force is the force with which the planet Earth attracts an object of mass 1 kg.
When the only force acting on the object is its weight, then it acquires an acceleration of 9.8 m / s². So 1 kg_f equals 9.8 N.
The weight P of the boy is then 50 kg * 9.8m / s² = 490 N
During acceleration the scale exerts a force N on the boy of 58 kg_f equivalent to 58 kg * 9.8 m / s² = 568.4 N.
The acceleration of the elevator will be given by:
a = N / M - g = 568.4 N / 50 kg - 9.8 m / s² = 1.57 m / s²
The velocity acquired by the elevator after 1.5 s with acceleration of 1.57 m / s² is:
v = a * t = 1.57 m / s² * 1.5 s = 2.36 m / s = 8.5 Km / h
The following figure shows a diagram of the forces acting on the boy:
The mayonnaise jar
A boy hands his brother the jar of mayonnaise to his brother, who is at the other end of the table. For that, it drives it in such a way that it acquires a speed of 3 m / s. From the moment the bottle was released until it stopped at the opposite end of the table, the travel was 1.5 m.
Determine the value of the friction force that the table exerts on the bottle, knowing that it has a mass of 0.45 kg.
Solution
First we will determine the braking acceleration. For this we will use the following relationship, already known from the uniformly accelerated rectilinear motion:
Vf² = Vi² + 2 * a * d
where Vf is the final speed, Saw initial velocity, to acceleration and d the displacement.
The acceleration obtained from the previous relationship is, where the displacement of the bottle has been taken as positive.
a = (0 - 9 (m / s) ²) / (2 * 1.5 m) = -3 m / s²
The net force on the mayonnaise jar is the friction force, since the normal and the weight of the jar balance: Fnet = Fr.
Fr = m * a = 0.45 kg * (-3 m / s²) = -1.35 N = -0.14 kg-f
Experiments for children
Children and adults can carry out simple experiments that allow them to verify that Newton's second law really works in real life. Here are two very interesting:
Experiment 1
A simple experiment requires a bathroom scale and an elevator. Take a bathroom weight into an elevator and record the values it marks during the up start, the down start, and during the time you are moving at constant speed. Calculate the elevator accelerations for each case.
Experiment 2
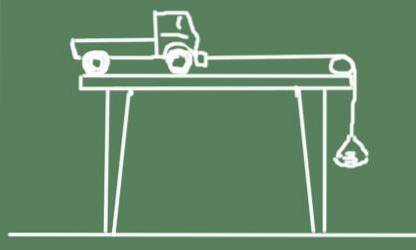
- Take a toy car that has its wheels well lubricated
- Attach a rope to the end.
- At the edge of the table, tape a pencil, or other smooth, cylindrical object over which the string will pass..
- At the other end of the rope, hang a small basket, to which you will place some coins or something that serves as a weight..
The scheme of the experiment is shown below:
- Drop the cart and watch it speed up.
- Then increase the mass of the cart by placing coins on it, or something that increases its mass..
- Say whether the acceleration increases or decreases. Put more dough on the cart, watch it accelerate, and finish.
The cart is then left without extra weight and allowed to accelerate. Then more weight is placed on the basket in order to increase the force applied to the cart..
- Compare the acceleration with the previous case, indicate if it increases or decreases. You can repeat adding more weight to the basket and observe the acceleration of the cart.
- Indicate if it increases or decreases.
- Analyze your results and say whether or not they agree with Newton's second law.
Articles of interest
Examples of Newton's Second Law.
Newton's first law.
Examples of Newton's Second Law.
References
- Alonso M., Finn E. 1970. Physics volume I: Mechanics. Inter-American Educational Fund S.A. 156-163.
- Hewitt, P. 2012. Conceptual Physical Science. Fifth edition. 41-46.
- Young, Hugh. 2015. University Physics with Modern Physics. 14th Ed. Pearson. 108-115.


Yet No Comments