
Bayes theorem explanation, applications, exercises
The Bayes theorem is a procedure that allows us to express the conditional probability of a random event A given B, in terms of the probability distribution of event B given A and the probability distribution of only A.
This theorem is very useful, since thanks to it we can relate the probability that an event A occurs knowing that B occurred, with the probability that the opposite occurs, that is, that B occurs given A.
Bayes' theorem was a silver proposition by the Reverend Thomas Bayes, an 18th century English theologian who was also a mathematician. He was the author of several works in theology, but at present he is known for a couple of mathematical treatises, among which the already mentioned Bayes Theorem stands out as the main result.
Bayes dealt with this theorem in a work entitled "An Essay towards solving a Problem in the Doctrine of Chances", published in 1763, and on which great numbers have been developed. studies with applications in various areas of knowledge.
Article index
- 1 Explanation
- 2 Applications of Bayes' Theorem
- 2.1 Solved Exercises
- 3 References
Explanation
First, for a better understanding of this theorem, some basic notions of probability theory are necessary, especially the multiplication theorem for conditional probability, which states that

For E and A arbitrary events of a sample space S.
And the definition of partitions, which tells us that if we have A1 ,TOtwo,… , TOn events of a sample space S, these will form a partition of S, if the Ai are mutually exclusive and their union is S.
Given this, let B be another event. So we can see B as
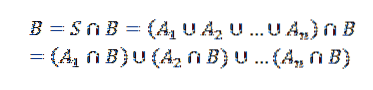
Where the Ai intersected with B are mutually exclusive events.
And in consequence,
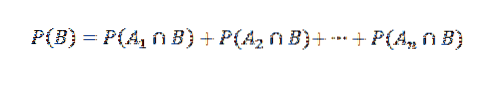
Then, applying the multiplication theorem
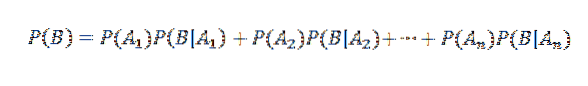
On the other hand, the conditional probability of Ai given B is defined by
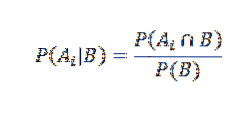
Substituting appropriately we have that for any i
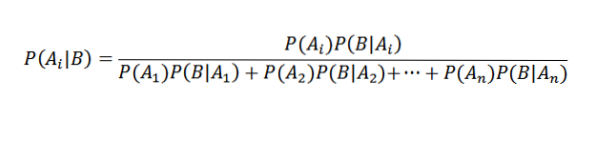
Applications of Bayes' Theorem
Thanks to this result, research groups and various corporations have managed to improve systems that are based on knowledge..
For example, in the study of diseases, Bayes' theorem can help to discern the probability that a disease is found in a group of people with a given characteristic, taking as data the global rates of the disease and the predominance of said characteristics in both healthy and sick people.
On the other hand, in the world of high technologies, it has influenced large companies that have developed, thanks to this result, "Knowledge-Based" software.
As a daily example we have the Microsoft Office assistant. The Bayes theorem helps the software to evaluate the problems that the user presents and determine what advice to provide and thus be able to offer a better service according to the user's habits.
It should be noted that this formula was ignored until recent times, this is mainly because when this result was developed 200 years ago, there was little practical use for them. However, in our time, thanks to great technological advances, scientists have found ways to put this result into practice.
Solved Exercises
Exercise 1
A cell phone company has two machines A and B. 54% of the cell phones produced are made by machine A and the rest by machine B. Not all cell phones produced are in good condition.
The proportion of defective cell phones made by A is 0.2 and by B is 0.5. What is the probability that a cell phone from that factory is defective? What is the probability that, knowing that a cell phone is defective, it comes from machine A?
Solution
Here, you have an experiment that is performed in two parts; in the first part the events occur:
A: cell made by machine A.
B: cell made by machine B.
Since machine A produces 54% of cell phones and the rest are produced by machine B, it follows that machine B produces 46% of cell phones. The probabilities of these events are given, namely:
P (A) = 0.54.
P (B) = 0.46.
The events of the second part of the experiment are:
D: defective cell phone.
E: non-defective cell phone.
As stated in the statement, the probabilities of these events depend on the result obtained in the first part:
P (D | A) = 0.2.
P (D | B) = 0.5.
Using these values, the probabilities of the complements of these events can also be determined, that is:
P (E | A) = 1 - P (D | A)
= 1 - 0.2
= 0.8
Y
p (E | B) = 1 - P (D | B)
= 1 - 0.5
= 0.5.
Now event D can be written as follows:
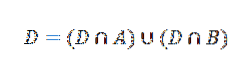
Using the Multiplication Theorem for conditional probability results:
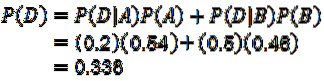
With which the first question is answered.
Now we only need to calculate P (A | D), for which Bayes's Theorem is applied:
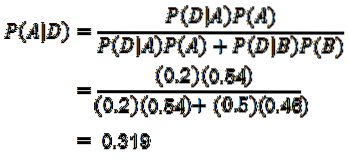
Thanks to Bayes' theorem, it can be stated that the probability that a cell phone has been made by machine A, knowing that the cell phone is defective, is 0.319.
Exercise 2
Three boxes contain black and white balls. The composition of each of them is as follows: U1 = 3B, 1N, U2 = 2B, 2N, U3 = 1B, 3N.
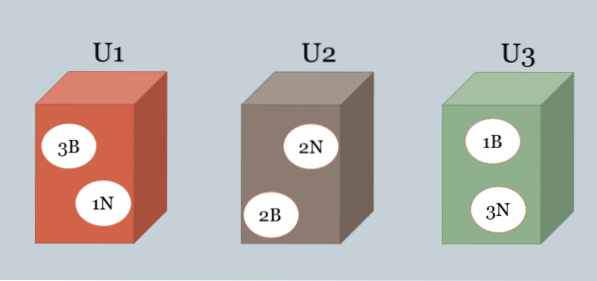
One of the boxes is chosen at random and a ball is drawn at random which turns out to be white. What is the box most likely to have been chosen?
Solution
Using U1, U2 and U3, we will also represent the chosen box.
These events constitute a partition of S and it is verified that P (U1) = P (U2) = P (U3) = 1/3 since the choice of the box is random.
If B = the drawn ball is white, we will have P (B | U1) = 3/4, P (B | U2) = 2/4, P (B | U3) = 1/4 .
What we want to obtain is the probability that the ball has been taken out of the box Ui knowing that said ball was white, that is, P (Ui | B), and see which of the three values was the highest to know of which box has been most likely the extraction of the white ball.
Applying Bayes' theorem to the first of the boxes:
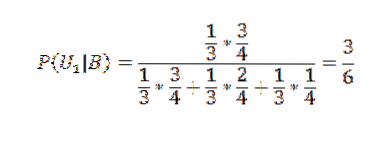
And for the other two:
P (U2 | B) = 2/6 and P (U3 | B) = 1/6.
Then, the first of the boxes is the one with the highest probability of having been chosen for the extraction of the white ball..
References
- Kai Lai Chung. Elementary Proability Theory with Stochastic Processes. Springer-Verlag New York Inc
- Kenneth.H. Rosen. Discrete Mathematics and its Applications. S.A. MCGRAW-HILL / INTERAMERICANA DE ESPAÑA.
- Paul L. Meyer. Probability and Statistical Applications. S.A. MEXICAN ALHAMBRA.
- Seymour Lipschutz Ph.D. 2000 Solved Problems of Discrete Mathematics. McGRAW-HILL.
- Seymour Lipschutz Ph.D. Theory and Probability Problems. McGRAW-HILL.


Yet No Comments