Isosceles trapezoid properties, relationships and formulas, examples

A trapeze isosceles is a quadrilateral in which two of the sides are parallel to each other and also, the two angles adjacent to one of those parallel sides have the same measure.

In figure 1 we have the quadrilateral ABCD, in which the sides AD and BC are parallel. Additionally, the angles ∠DAB and ∠ADC adjacent to the parallel side AD have the same measure α. 

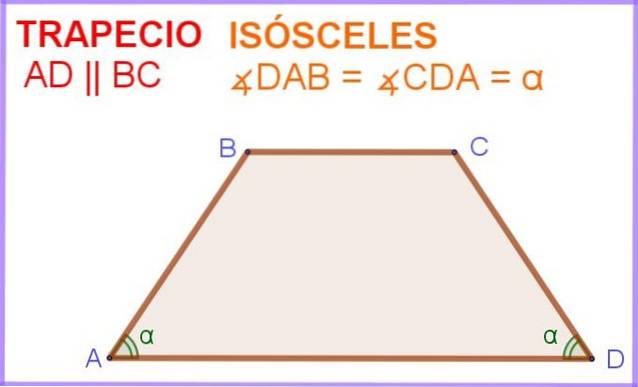
So this quadrilateral, or four-sided polygon, is in effect an isosceles trapezoid.

In a trapezoid, the parallel sides are called bases and the non-parallels are called lateral. Another important feature is the height, which is the distance that separates the parallel sides.

In addition to the isosceles trapezoid there are other types of trapezoid:

-Tscalene monkfish, which has all its different angles and sides.

-Tanglerfish rectangle, in which a lateral has right adjacent angles.

The trapezoidal shape is common in various fields of design, architecture, electronics, calculation and many more, as will be seen later. Hence the importance of becoming familiar with its properties.

Article index

• 1 Properties
  • 1.1 Exclusive to the isosceles trapezoid
  • 1.2 For all trapezoids
• 2 Relations and formulas
  • 2.1 Unique relationships of the isosceles trapezium
  • 2.2 Relationships for any trapezoid
  • 2.3 Relations for isosceles trapezoid with inscribed circumference
  • 2.4 Formulas for determining one side, knowing the others and an angle
  • 2.5 Determination of one side, knowing the others and a diagonal
  • 2.6 Base from height, area and other base
  • 2.7 Known lateral bases, area and an angle
  • 2.8 Known lateral median, area and angle
  • 2.9 Known height of the sides
  • 2.10 Known height an angle and two sides
  • 2.11 Known diagonals all sides, or two sides and an angle
  • 2.12 Perimeter of the isosceles triangle 
  • 2.13 Area of ​​the isosceles trapezoid
  • 2.14 Radius of the circumscribed circle
• 3 Examples of use of the isosceles trapezoid
  • 3.1 In architecture and construction
  • 3.2 In design
• 4 Solved exercises
  • 4.1 - Exercise 1
  • 4.2 - Exercise 2
• 5 References

Properties

Exclusive to the isosceles trapezoid

If a trapezoid is isosceles then it has the following characteristic properties:

1.- The sides have the same measurement.

2.- The angles adjacent to the bases are equal.

3.- Opposite angles are supplementary.

4.- The diagonals have the same length, the two segments that join the opposite vertices being the same.

5.- The angle formed between the bases and the diagonals are all of the same measure.

6.- It has a circumscribed circumference.

Conversely, if a trapezoid fulfills any of the above properties, then it is an isosceles trapezoid.

If in an isosceles trapezoid one of the angles is right (90º), then all the other angles will be right too, forming a rectangle. That is, a rectangle is a particular case of isosceles trapezoid.

For all trapeze

The following set of properties are valid for any trapezoid:

7.- The median of the trapezoid, that is, the segment that joins the midpoints of its non-parallel sides, is parallel to any of the bases.

8.- The length of the median is equal to the semisum (sum divided by 2) of that of its bases.

9.- The median of a trapezoid cuts its diagonals at the midpoint.

10.- The diagonals of a trapezoid intersect at a point that divide them into two sections proportional to the quotients of the bases.

11.- The sum of the squares of the diagonals of a trapezoid is equal to the sum of the squares of its sides plus the double product of its bases.

12.- The segment that joins the midpoints of the diagonals has a length equal to the semidifference of the bases.

13.- The angles adjacent to the lateral ones are supplementary.

14.- A trapezoid has an inscribed circumference if and only if the sum of its bases is equal to the sum of its sides.

15.- If a trapezoid has an inscribed circumference, then the angles with a vertex in the center of said circumference and sides that pass through the ends of the same side are right angles.

Relationships and formulas

The following set of relations and formulas refer to figure 3, where in addition to the isosceles trapezoid other important segments already mentioned are shown, such as diagonals, height and median.

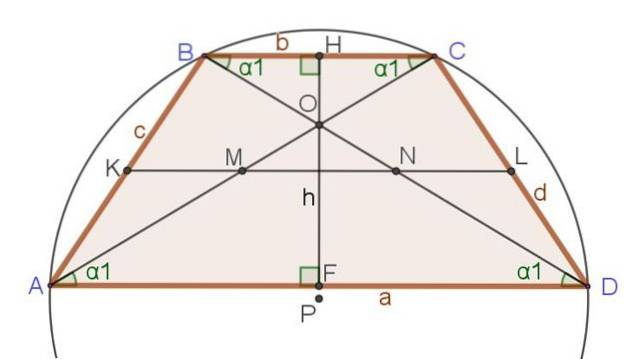
Unique relationships of the isosceles trapezium

1.- AB = DC = c = d

2.- ∡DAB = ∡CDA and ∡ABC = ∡BCD

3.- ∡DAB + ∡BCD = 180º and ∡CDA + ∡ABC = 180º

4.- BD = AC

5.- ∡CAD = ∡BDA = ∡CBD = ∡BCA = α₁

6.- A, B, C and D belong to the circumscribed circle.

Relationships for any trapezoid

7. If AK = KB and DL = LC ⇒ KL || AD and KL || BC

8.- KL = (AD + BC) / 2

9.- AM = MC = AC / 2 and DN = NB = DB / 2

10.- AO / OC = AD / BC and DO / OB = AD / BC

11.- AC^two + DB^two = AB^two + DC^two + 2⋅AD⋅BC

12.- MN = (AD - BC) / 2

13.- ∡DAB + ∡ABC = 180º and ∡CDA + ∡BCD = 180º

14.- If AD + BC = AB + DC ⇒ ∃ R than equidistant from AD, BC, AB and DC

15.- If ∃ R equidistant from AD, BC, AB and DC, then:

∡BRA = ∡DRC = 90º

Relations for isosceles trapezoid with inscribed circumference

If in an isosceles trapezoid the sum of the bases is equal to twice a lateral one, then the inscribed circumference exists.

The following properties apply when the isosceles trapezoid has an inscribed circumference (see figure 4 above):

16.- KL = AB = DC = (AD + BC) / 2

17.- The diagonals intersect at right angles: AC ⊥ BD

18.- The height measures the same as the median: HF = KL, that is, h = m.

19.- The square of the height is equal to the product of the bases: h^two = BC⋅AD

20.- Under these specific conditions, the area of ​​the trapezoid is equal to the square of the height or the product of the bases: Area = h^two = BC⋅AD.

Formulas for determining one side, knowing the others and an angle

Knowing a base, the lateral and an angle, the other base can be determined by:

a = b + 2c Cos α

b = a - 2c Cos α

If the length of the bases and an angle are given as known data, then the lengths of both sides are:

c = (a - b) / (2 Cos α)

Determination of one side, knowing the others and a diagonal

a = (d₁^two - c^two) / b;

b = (d₁^two - c^two)/ to 

c = √ (d₁^two - a⋅b)

Where d₁ is the length of the diagonals.

Base from height, area, and other base

a = (2 A) / h - b

b = (2 A) / h - a

Known lateral bases, area and an angle

c = (2A) / [(a + b) sin α]

Known lateral median, area and angle

c = A / (m sin α)

Known height the sides

h = √ [4 c^two - (a - b)^two]

Known height an angle and two sides

h = tg α⋅ (a - b) / 2 = c. sin α

Known diagonals all sides, or two sides and an angle

d₁ = √ (c^two+ a b)

d₁ = √ (a^two+ c^two - 2 a c Cos α)

d₁ = √ (b^two + c^two- 2 b c Cos β)

Perimeter of the isosceles triangle 

P = a + b + 2c

Isosceles trapezium area

There are several formulas for calculating the area, depending on the data that is known. The following is the best known, depending on the bases and height:

A = h⋅ (a + b) / 2

And you can also use these others:

-If the sides are known

A = [(a + b) / 4] √ [4c^two - (a - b)^two]

-When you have two sides and an angle

A = (b + c Cos α) c Sen α = (a - c Cos α) c Sen α

-If the radius of the inscribed circle and an angle are known

A = 4 r^two / Sen α = 4 r^two / Sen β

-When the bases and an angle are known

A = a⋅b / Sen α = a⋅b / Sen β 

-If the trapezoid can be inscribed a circumference

A = c⋅√ (a⋅b) = m⋅√ (a⋅b) = r⋅ (a + b) / 2

-Known the diagonals and the angle they form with each other

A = (d₁^two/ 2) Sen γ = (d₁^two / 2) Sen δ 

-When you have the lateral, the median and an angle

A = mc.sen α = mc.sen β

Radius of the circumscribed circle

Only isosceles trapezoids have a circumscribed circumference. If the greater base a, the lateral c and the diagonal d are known₁, then the radius R of the circle that passes through the four vertices of the trapezoid is:

R = a⋅c⋅d₁ / 4√ [p (p -a) (p -c) (p - d₁)]

Where p = (a + c + d₁) / two

Examples of using the isosceles trapezoid

The isosceles trapezoid appears in the field of design, as seen in Figure 2. And here are some additional examples:

In architecture and construction

The ancient Incas knew the isosceles trapezoid and used it as a building element in this window in Cuzco, Peru:

And here the trapeze appears again in the call trapezoidal sheet, a material frequently used in construction:

In design

We have already seen that the isosceles trapezoid appears in everyday objects, including foods like this chocolate bar:

Solved exercises

- Exercise 1

An isosceles trapezoid has a base greater than 9 cm, a base less than 3 cm, and its diagonals 8 cm each. Calculate:

a) Side

b) Height

c) Perimeter

d) Area

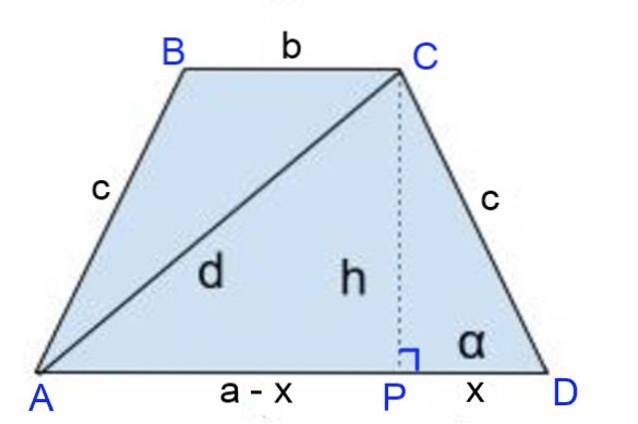
Solution to

The height CP = h is plotted, where the foot of the height defines the segments:

PD = x = (a-b) / 2 y 

AP = a - x = a - a / 2 + b / 2 = (a + b) / 2.

Using the Pythagorean theorem to the right triangle DPC:

c^two = h^two + (a - b)^two /4

And also to the right triangle APC:

d^two = h^two + AP^two = h^two + (a + b)^two /4

Finally, member by member, the second equation is subtracted from the first and simplified:

d^two - c^two = ¼ [(a + b)^two - (a-b)^two] = ¼ [(a + b + a-b) (a + b-a + b)]

d^two - c^two = ¼ [2a 2b] = a b

c^two= d^two - a b ⇒ c = √ (d^two - a b) = √ (8^two - 9⋅3) = √37 = 6.08 cm

Solution b

h^two = d^two - (a + b)^two / 4 = 8^two - (12^two / two^two ) = 8^two - 6^two = 28

h = 2 √7 = 5.29 cm

Solution c

Perimeter = a + b + 2 c = 9 + 3 + 2⋅6.083 = 24.166 cm

Solution d

Area = h (a + b) / 2 = 5.29 (12) / 2 = 31.74 cm

- Exercise 2

There is an isosceles trapezoid whose largest base is twice the smallest and its smallest base is equal to the height, which is 6 cm. Decide:

a) The length of the lateral

b) Perimeter

c) Area

d) Angles

Solution to

Data: a = 12, b = a / 2 = 6 and h = b = 6

We proceed as follows: the height h is drawn and the Pythagorean theorem is applied to the hypotenuse triangle “c” and legs h and x:

c^two = h^two+xc^two

Then you have to calculate the value of the height from the data (h = b) and that of the leg x: 

a = b + 2 x ⇒ x = (a-b) / 2

Substituting the previous expressions we have:

c^two = b^two+(a-b)^two/two^two

Now the numerical values ​​are introduced and it is simplified:

c^two = 62+ (12-6) 2/4

c^two = 62 (1 + ¼) = 62 (5/4)

Obtaining:

c = 3√5 = 6.71 cm

Solution b

The perimeter P = a + b + 2 c

P = 12 + 6 + 6√5 = 6 (8 + √5) = 61.42 cm

Solution c

The area as a function of the height and length of the bases is:

A = h⋅ (a + b) / 2 = 6⋅ (12 + 6) / 2 = 54 cm^two

Solution d

The angle α formed by the lateral with the larger base is obtained by trigonometry:

Tan (α) = h / x = 6/3 = 2

α = ArcTan (2) = 63.44º

The other angle, the one that forms the lateral with the smaller base, is β, which is supplementary to α:

β = 180º - α = 180º - 63.44º = 116.56º

References

1. E. A. 2003. Elements of geometry: with exercises and compass geometry. University of Medellin.
2. Campos, F. 2014. Mathematics 2. Grupo Editorial Patria.
3. Freed, K. 2007. Discover Polygons. Benchmark Education Company.
4. Hendrik, V. 2013. Generalized Polygons. Birkhäuser.
5. IGER. Mathematics First Semester Tacaná. IGER.
6. Jr. geometry. 2014. Polygons. Lulu Press, Inc.
7. Miller, Heeren, & Hornsby. 2006. Mathematics: Reasoning And Applications. 10th. Edition. Pearson Education.
8. Patiño, M. 2006. Mathematics 5. Editorial Progreso.
9. Wikipedia. Trapeze. Recovered from: es.wikipedia.com