Balancing vector calculation, examples, exercises

The balancing vector It is the one that is opposed to the resulting vector and therefore is capable of balancing a system, since it has the same magnitude and the same direction, but the opposite direction to this.

On many occasions the balancing vector refers to a force vector. To calculate the balancing force, first find the resultant force, as shown in the following figure:

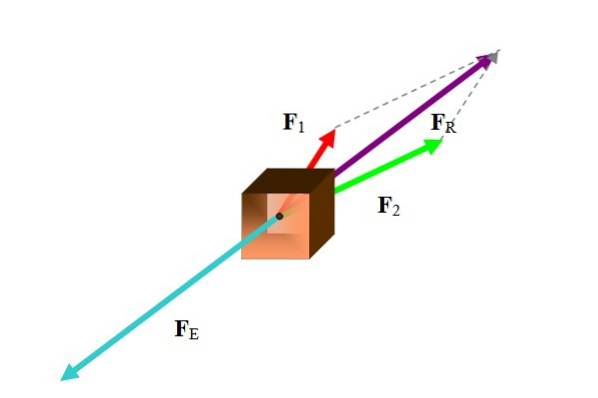
There are various methods of undertaking this task, depending on the data at hand. Since the forces are vectors, the resultant is the vector sum of the participating forces:

F_R = F₁ + F_two + F₃ +  ... .

Among the methods to be used are graphic methods such as polygonal, parallelogram and analytical methods such as decomposition of forces into their Cartesian components. In the example the figure used the parallelogram method.

Once the resultant force has been found, the balancing force is just the opposite vector.

Yes F_AND is the balancing force, then it is satisfied that F_AND applied at a certain point, it guarantees the translational equilibrium of the system. If it is a single particle, it will not move (or maybe it will move with constant speed), but if it is an extended object, it will still have the possibility to rotate:

F_R + F_AND = 0

Article index

• 1 Examples
  • 1.1 Force table
• 2 Solved exercises
  • 2.1 -Exercise 1
  • 2.2 -Exercise 2
• 3 References

Examples

Balancing forces are present everywhere. We ourselves are balanced by the force that the chair exerts to compensate for the weight. Objects that are at rest: books, furniture, ceiling lamps and a large number of mechanisms, are continually being balanced by forces.

For example, a book at rest on a table is balanced by the normal force that it exerts on the book, preventing it from falling. The same thing happens with the chain or cable that holds the lamp hanging from the ceiling in a room. The cables that hold a load distribute their weight through the tension in them.

In a fluid some objects are able to float and remain at rest, since their weight is balanced by an upward force exerted by the liquid, called push.

Various mechanisms need to be balanced knowing the balancing force vector such as bars, beams and columns.

When using a scale, it is necessary to somehow balance the weight of the object with a force that is equivalent, either by adding weights or by means of springs..

Force table

The force table is used in the laboratory to determine the balancing force. It consists of a circular platform, of which you have the top view in the figure, and which has a protractor to measure angles.

On the edges of the table there are pulleys through which ropes that hold weights pass and that converge in a ring that is in the center.

For example, two weights are hung. The tensions generated in the strings by these weights are drawn in red and blue in figure 2. A third weight in green can balance the resulting force of the other two and keep the system in balance..

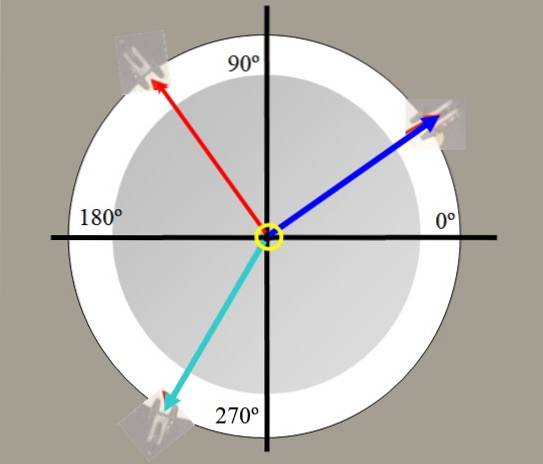
With the force table it is possible to verify the vector character of the forces, decompose forces, find the balancing force and verify Lamy's theorem:

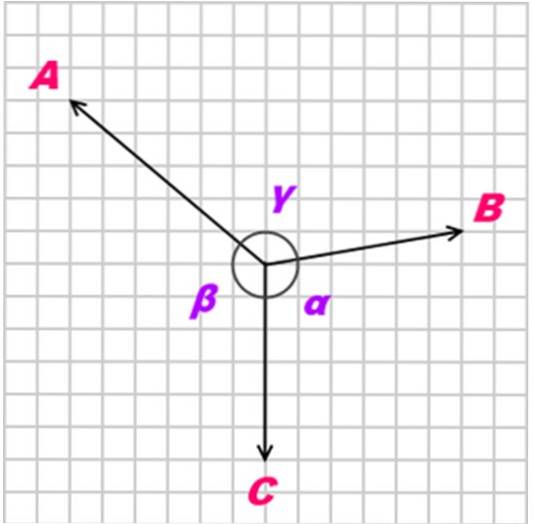
If a body is in equilibrium thanks to three coplanar forces, concurrent and non-collinear (not parallel), called TO, B Y C, the relationship between these forces is as follows:

A / sin α = B / sin β = C / sin γ

Solved exercises

-Exercise 1

225 g (blue tension) and 150 g (red tension) weights are hung on the force table of Figure 2, with the angles shown. Find the value of the balancing force and the angle it makes with the vertical axis.

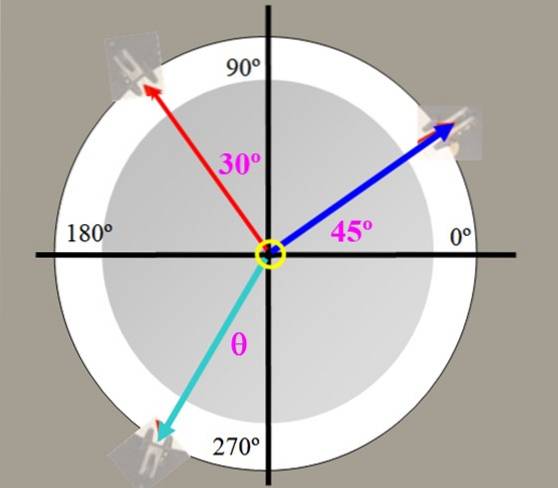
Solution

The problem can be worked with the weights expressed in grams (forces). Let P₁ = 150 grams and P_two = 225 grams, the respective components of each are:

P_1x = 225. cos 45 ° g = 159.10 g; P_1y = 225. cos 45º g = 159.10 g

P_2x = -150. sin 30 g = -75.00 g; P_{2 and} = 150. cos 30º g = 129.90 g

The resulting weight P_R is found by algebraically adding the components:

P_Rx = 159.10 - 75.00 g = 84.10 g

P_Ry = 159.10 + 129.90 g = 289.00 g

The balancing weight P_AND is the vector opposite P_R:

P_Former = -84.10 g

P_Hey = -289.00 g

The magnitude of the balancing weight is calculated by:

P_AND = (P_Former^two + P_Hey^two)^1/2 = ((-84.10)^two + (-289.00)^two)^1/2 g = 301 g

The angle θ in the figure is:

θ = arctg (-84.10 / -289.00) = 16.2º with respect to the axis Y negative.

-Exercise 2

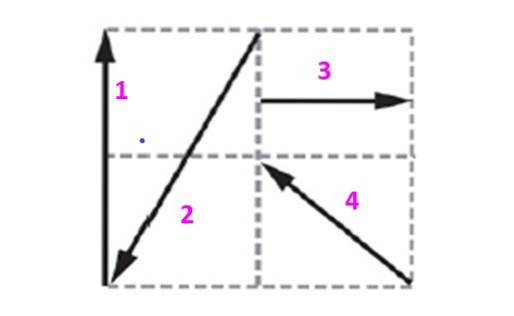
Find the balancing vector of the system shown in the figure, knowing that each square measures 10 m on a side.

Solution

The vectors contained in this grid will be expressed in terms of the unit and orthogonal vectors i Y j that determine the plane. Vector 1, which will be denoted as v₁ it has magnitude 20 m and is directed vertically upwards. It can be expressed as:

v₁ = 0 i +twenty j m

From the drawing it can be seen that vector 2 is:

v_two = -10 i - twenty j m

Vector 3 is horizontal and points in the positive direction:

v₃ = 10 i + 0 j  m

Finally vector 4 is inclined 45º, since it is the diagonal of the square, therefore its components measure the same:

v₄ = -10 i + 10 j m

Note that the signs indicate towards which side of the axis the components are: above and to the right have a + sign, while below and to the left they have a sign -.

The resulting vector is obtained by adding component to component:

v_R = -10 i + 10 j m

Then the balancing vector of the system is:

v_AND = 10 i - 10 j m

References

1. Beardon, T. 2011. An introduction to vectors. Retrieved from: nrich.maths.org.
2. Bedford, 2000. A. Engineering Mechanics: Statics. Addison Wesley. 38-52.
3. Figueroa, D. Series: Physics for Sciences and Engineering. Volume 1. Kinematics 31-68.
4. Physical. Module 8: Vectors. Recovered from: frtl.utn.edu.ar
5. Hibbeler, R. 2006. Mechanics for Engineers. Static. 6th Edition. Continental Publishing Company. 15-53.
6. Vector Addition Calculator. Recovered from: 1728.org
7. Vectors. Recovered from: wikibooks.org