Significant figures rules, examples, solved exercises

Is named Significant numbers to the number of digits contained in the mantissa of a number. The more numbers you have, the more precisely the quantity is known. As a reminder, the mantissa is the figure that accompanies the power of 10 when the number is written in scientific notation..

For example, let's take the number 0.00376, which is written as 3.76 x 10 ^-3. The mantissa is 3.76 and the number has a total of 3 significant figures. The number 0.129 also has 3 significant figures, while 4.5 has only 2.

And what happens when the number is an integer? It means that it is known with as much precision as possible, in other words, it has infinite precision. For example, when counting people, animals, or objects such as books and telephones, the result is a precise whole number.

If we say that in a movie theater there are 110 people watching a movie, this is the exact number, neither more nor less, and it has 3 significant figures.

Significant figures are handled by some simple rules that are memorized with a little practice, as we will see below..

Article index

• 1 Rules for determining the significant figures of a number
  • 1.1 Rule 1
  • 1.2 Rule 3
  • 1.3 Rule 4
  • 1.4 Rule 5
• 2 Examples
  • 2.1 Example 1
  • 2.2 Example 2
  • 2.3 Example 3
• 3 Rules for rounding numbers
• 4 References

Rules for determining the significant figures of a number

Rule 1

The leading zeros do not count as a significant figure, so 0.045 and 4.5 both have 2 significant figures, since these start to be counted from the left and starting from the first non-zero digit.

Rule 2

The zeros after (to the right) the first significant digit do count as a significant figure (as long as it is justified by the precision of the measuring instrument).

Finally, the zeros in the middle are also counted as a significant digit..

Rule 3

For numbers written in scientific notation, all figures in the mantissa are significant, and the exponent does not influence the precision.

Rule 4

When doing operations with decimals, for example when calculating areas or other similar operations, the result must have the same number of significant figures as the quantity with the fewest number of significant figures that participated in the operation. This rule is valid for any arithmetic operation.

Rule 5

The sign of the number does not influence its number of significant figures.

We will see immediately some examples of this and all the other rules.

Examples

Example 1

Find how many significant figures are in each of these numbers.

a) 876

b) 1000.68

c) 0.00005026

d) 4.8

e) -6.99

Answers

a) 876 has 3 significant figures.

b) 1000.68 has 6 significant figures, since the zeros in between count as such.

c) Instead 0.00005026 has 4 significant figures. Note that the 5 zeros to the left of the 5 are not counted as a significant figure, instead the 0 that is between 5 and 2 is..

d) 4.8 has 2 significant figures.

e) -6.99 has 3 significant figures.

Example 2

It is common to take measurements with measuring instruments, such as tape measures, watches, thermometers, scales and so on. With how many significant figures should we report the quantities that we measure in this way?

Answer

It depends on the appreciation of the instrument with which it is measured. Let's take an example: measure the external diameter of a tube, with a graduated ruler and with a vernier or caliper.

The vernier is an instrument that measures lengths very precisely because it has an extra small scale, called vernier, which allows for greater fineness, so to speak, when measuring.

It is more precise than a graduated ruler because with it we can know more significant figures of a certain length.

That is why it makes no sense to report a perimeter of, say, 35.88 cm if we measure it with a tape measure, since this instrument is not accurate enough to report so many significant digits..

The appreciation A of the tape measure is given by:

Example 3

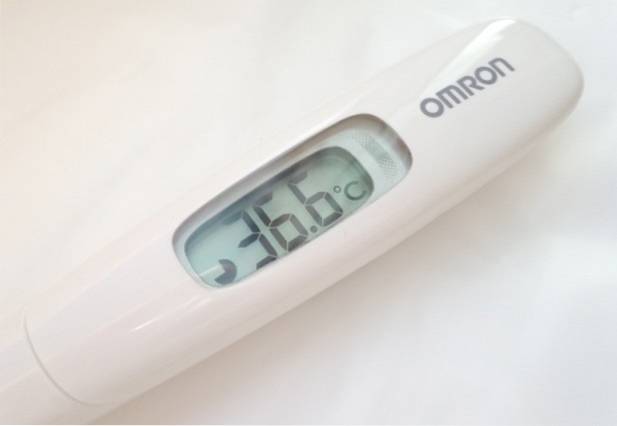
How many significant figures does the reading made with the digital thermometer have?

Answer

The thermometer in the figure provides three-digit temperature readings. However, in the measurement shown, of 36.6 ºC, only the first two digits from left to right are accurate, since the decimal is affected by the instrument's appreciation error, which is usually indicated on the back of the instrument or on the your operations manual.

The usual thing for the type of digital instrument shown is an error of appreciation of 0.1 ºC. This is enough to be sure that you do not have a fever..

Rules for rounding numbers

When using a calculator to perform calculations with measurements obtained, it is not correct to give the result using all the digits that appear on the screen.

Only those that are precisely known are retained, as only those have true meaning. Then it becomes necessary to round the results to fit the number of precisely known figures. These rules are:

-If the number following the digit to be withheld is equal to or greater than 5, 1 is added to this digit.

For example, when rounding 3.786 to two decimals, we want to retain the numbers up to 8. Since the number that follows (6) is greater than 5, the 8 becomes 8 + 1 = 9 and the number remains as 3.79.

-When the number following the digit to be retained is less than 5, the digit remains the same.

If we want to round 1.27924 so that it has only 3 decimal places, this is achieved by reaching 9, which is followed by 2. Since 2 is less than 5, these decimals disappear and the rounded number remains 1.279.

Exercise resolved

A dining table has the shape and dimensions indicated in the accompanying figure. You are asked to calculate its area using the rules of operations with significant figures.

Solution

The table area can be divided into a central rectangular area and two semi-circles, one on each side, which together make 1 full circle.

We will call A₁ to the area of ​​the rectangle, given by:

TO₁ = base × height = 2.5 m x 1.0 m = 2.5 m^two

For its part, the area of ​​the circle, which is equal to that of 1 semicircle multiplied by 2 is:

TO_two = π × radius^two

The diameter of any of the semicircles is 1.0 m, therefore the radius is 0.50 m. The diameter could also be used directly to calculate the area, in this case:

TO_two = (π × diameter^two) / 4

In any case:

TO_two = [π x (1.0 m)^two] / 4 = 0.785398163 m^two

All the digits provided by the calculator were used. Now we add A₁ already_two for the total area A of the table:

A = (2.5 + 0.785398163) m^two = 3.285398163 m^two

As the dimensions of the table are known to 2 significant figures, it does not make sense to express the result with all the decimal places given by the calculator, which never gives the number of significant figures of a result.

What you have to do is round the area so that it has the same number of significant figures as the dimensions of the table, that is, 2. Therefore, the final result is reported as follows:

A = 3.3 m^two

References

1. Bauer, W. 2011. Physics for Engineering and Sciences. Volume 1. Mc Graw Hill.
2. Figueroa, D. (2005). Series: Physics for Science and Engineering. Volume 1. Kinematics. Edited by Douglas Figueroa (USB).
3. Fisicalab. Significant figures and rounding. Recovered from: fisicalab.com.
4. Giancoli, D. 2006. Physics: Principles with Applications. 6th. Ed prentice hall.
5. Sears, Zemansky. 2016. University Physics with Modern Physics. 14th. Ed. Volume1.