
Constant function characteristics, examples, exercises
The constant function is the one in which the value of y is kept constant. In other words: a constant function always has the form f (x) = k, where k is a real number.
When graphing the constant function in the coordinate system xy, always results in a straight line parallel to the horizontal axis or axis of the x.
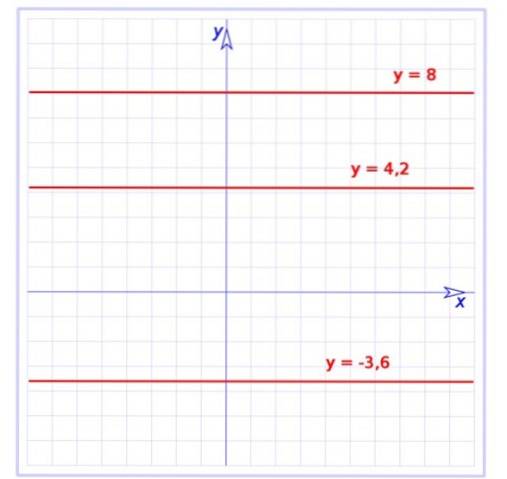
This function is a particular case of the affine function, whose graph is also a straight line, but with a slope. The constant function has zero slope, that is, it is a horizontal line, as shown in figure 1.
There the graph of three constant functions is shown:
f (x) = -3.6
g (x) = 4.2
h (x) = 8
All are lines parallel to the horizontal axis, the first of them is below said axis, while the rest are above.
Article index
- 1 Characteristics of the constant function
- 2 Examples
- 2.1 Another way to represent a constant function
- 3 Solved exercises
- 3.1 - Exercise 1
- 3.2 - Exercise 2
- 3.3 - Exercise 3
- 3.4 - Exercise 4
- 3.5 - Exercise 6
- 4 References
Characteristics of the constant function
We can summarize the main characteristics of the constant function as follows:
-Its graph is a straight horizontal line.
-It has a single intersection with the axis Y, worth k.
-Is continuous.
-The domain of the constant function (the set of values that the x) is the set of real numbers R.
-The path, range or counter-domain (the set of values that the variable takes Y) is simply the constant k.
Examples
Functions are necessary to establish links between quantities that depend on each other in some way. The relationship between them can be mathematically modeled, to find out how one of them behaves when the other varies..
This helps to build models for many situations and make predictions about their behavior and evolution..
Despite its apparent simplicity, the constant function has many applications. For example, when it comes to studying magnitudes that remain constant over time, or at least for an appreciable time.
In this way, magnitudes behave in situations such as the following:
-The velocity cruising a car moving down a long straight highway. As long as you do not brake or accelerate, the car has a uniform rectilinear movement.

-A fully charged capacitor disconnected from a circuit has a load constant in time.
-Finally, a flat rate parking lot maintains a price constant no matter how long a car is parked there.
Another way to represent a constant function
The constant function can alternatively be represented as follows:
f (x) = kx0
Since any value of x raised to 0 gives 1 as a result, the previous expression reduces to the already familiar one:
f (x) = k
Of course that happens as long as the value of k is different from 0.
That is why the constant function is also classified as a polynomial function of degree 0, since the exponent of the variable x is 0.
Solved exercises
- Exercise 1
Answer the following questions:
a) Can it be stated that the line given by x = 4 is a constant function? Reason for your answer.
b) Can a constant function have an x-intercept?
c) Is the function f (x) = w constanttwo?
Answer to
Here is the graph of the line x = 4:
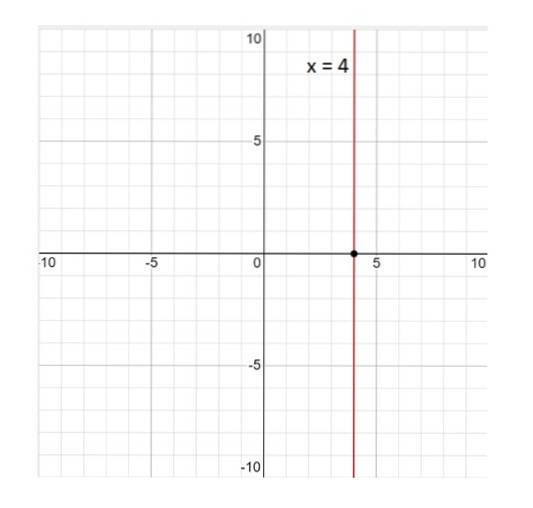
The line x = 4 is not a function; by definition a function is a relation such that at each value of the variable x corresponds to a single value of Y. And in this case this is not true, since the value x = 4 is associated with infinite values of Y. Therefore the answer is no.
Answer b
In general a constant function has no intersection with the axis x, unless it's about y = 0, in which case it is the axis x Properly said.
Answer c
Yes, since w is constant, its square is also constant. What matters is that w don't depend on input variable x.
- Exercise 2
Find the intersection between the functions f (x) = 5 Y g (x) = 5x - 2
Solution
To find the intersection between these two functions, they can be respectively rewritten as:
y = 5; y = 5x - 2
They are equalized, obtaining:
5x - 2 = 5
What is a linear equation of the first degree, whose solution is:
5x = 5 + 2 = 7
x = 7/5
The point of intersection is (7/5; 5).
- Exercise 3
Show that the derivative of a constant function is 0.
Solution
From the definition of derivative we have:
f (x + h) = k
Substituting in the definition:

Also, if we think of the derivative as the rate of change dy / dx, the constant function does not undergo any change, therefore its derivative is zero.
- Exercise 4
Find the indefinite integral of f (x) = k.
Solution

A cell phone company offers unlimited flat rate Internet service for $ 15 per month. What is the price function according to time?
Solution
Let P be the price to pay in $ and t the time, which can be expressed in days. The function is set like this:
P (t) = 15
- Exercise 6
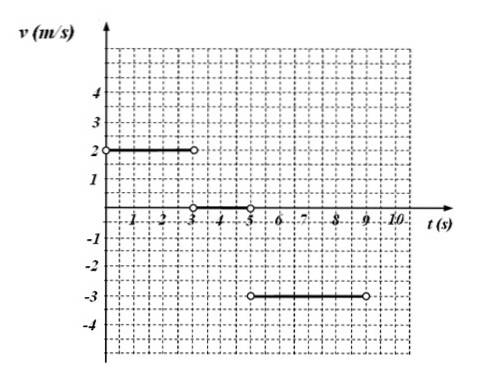
The following graph of velocity versus time corresponds to the motion of a particle.

It asks:
a) Write an expression for the velocity function as a function of time v (t).
b) Find the distance traveled by the mobile in the time interval between 0 and 9 seconds.
Solution to
From the graph shown, it can be seen that:
-v = 2 m / s in the time interval between 0 and 3 seconds
-The mobile is stopped between 3 and 5 seconds, since in this interval the speed is 0.
-v = - 3 m / s between 5 and 9 seconds.
It is an example of a piecewise function, or piecewise function, which in turn is composed of constant functions, valid only for the indicated time intervals. It is concluded that the sought function is:

Solution b
From the v (t) graph, the distance traveled by the mobile can be calculated, which is numerically equivalent to the area under / on the curve. In this way:
-Distance traveled between 0 and 3 seconds = 2 m / s. 3 s = 6 m
-Between 3 and 5 seconds he was stopped, therefore he did not travel any distance.
-Distance traveled between 5 and 9 seconds = 3 m / s. 4 s = 12 m
In total, the mobile traveled 18 m. Note that although the speed is negative in the interval between 5 and 9 seconds, the distance traveled is positive. What happens is that during that time interval, the mobile had changed the sense of its speed.
References
- Geogebra. Constant functions. Recovered from: geogebra.org.
- Maplesoft. The Constant Function. Recovered from: maplesoft.com.
- Wikibooks. Calculation in a variable / Functions / Constant function. Recovered from: es.wikibooks.org.
- Wikipedia. Constant function. Recovered from: en.wikipedia.org
- Wikipedia. Constant function. Recovered from: es.wikipedia.org.


Yet No Comments