Moment of inertia formulas, equations and calculation examples

The moment of inertia of a rigid body with respect to a certain axis of rotation, represents its resistance to changing its angular velocity around said axis. It is proportional to the mass and also to the location of the axis of rotation, since the body, depending on its geometry, can rotate more easily around certain axes than in others.

Suppose a large object (consisting of many particles) which can rotate around an axis. Suppose a force acts F, applied tangentially on the mass element Δm_i, that produces a torque or moment, given by τ_net = ∑r_i x F_i. The vector r_i is the position of Δm_i (see figure 2).

This moment is perpendicular to the plane of rotation (direction +k = coming out of the paper). Since the force and the radial position vector are always perpendicular, the cross product remains:

τ_net = ∑ F_i r_i k = ∑ (Δm_i to_i) r_i  k = ∑ Δm_i (to_i r_i ) k

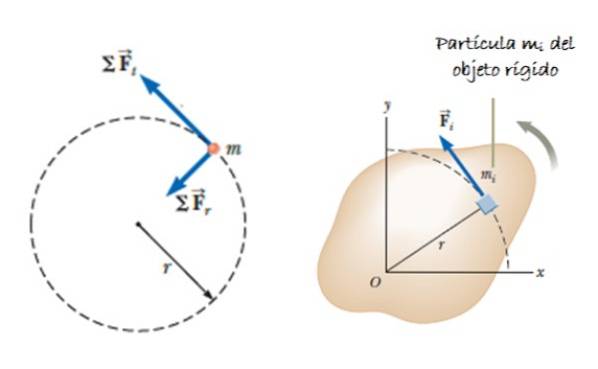
Acceleration to_i represents the tangential component of acceleration, since radial acceleration does not contribute to torque. As a function of the angular acceleration α, we can indicate that:

to_i = α r_i

Therefore the net torque looks like this:

τ_net = ∑ Δm_i (α r_i^two) k = (∑ r_i^two Δm_i) α k

The angular acceleration α is the same for the whole object, therefore it is not affected by the subscript "i" and can leave the summation, which is precisely the moment of inertia of the object symbolized by the letter I:

I = ∑ r_i^two Δm_i

This is the moment of inertia of a discrete mass distribution. When the distribution is continuous, the summation is replaced with an integral and Δm becomes a mass differential dm. The integral is carried out over the entire object:

I = ∫_M(r^two) dm

The units of the moment of inertia in the SI International System are kg x m^two. It is a scalar and positive quantity, since it is the product of a mass and the square of a distance.

Article index

• 1 Calculation examples
  • 1.1 Moment of inertia of a thin bar with respect to an axis passing through its center
  • 1.2 Moment of inertia of a disk with respect to an axis passing through its center
  • 1.3 Moment of inertia of a solid sphere with respect to a diameter
  • 1.4 Moment of inertia of a solid cylinder with respect to the axial axis
  • 1.5 Moment of inertia of a rectangular sheet with respect to an axis passing through its center
  • 1.6 Moment of inertia of a square sheet with respect to an axis passing through its center
• 2 Moment of inertia theorems
  • 2.1 Steiner's theorem
  • 2.2 Perpendicular axes theorem
• 3 Exercise resolved
• 4 References

Calculation examples

An extended object, such as a bar, disk, sphere, or other, whose density ρ is constant and knowing that the density is the mass - volume ratio, the mass differential dm is written as:

ρ = dm / dV → dm = ρdV

Substituting in the integral for the moment of inertia, we have:

I = ∫r^two ρdV = ρ ∫r^twodV

This is a general expression, valid for a three-dimensional object, whose volume V and position r are functions of the spatial coordinates x, Y Y z. Note that being constant, the density is outside the integral.

The density ρ It is also known as volumetric density, but if the object is very flat, like a sheet or very thin and narrow like a rod, other forms of density can be used, let's see:

- For a very thin sheet, the density to use is σ, the surface density (mass per unit area) and gives is the area differential.

- And if it is a thin bar, where only the length is relevant, the linear mass density is used λ and a length differential, according to the axis used as a reference.

In the examples that follow, all objects are considered to be rigid (not deformable) and have uniform density.

Moment of inertia of a thin bar with respect to an axis passing through its center

Here we are going to calculate the moment of inertia of a thin, rigid, homogeneous bar of length L and mass M, with respect to an axis that passes through the middle.

First, it is necessary to establish a coordinate system and build a figure with the appropriate geometry, like this:

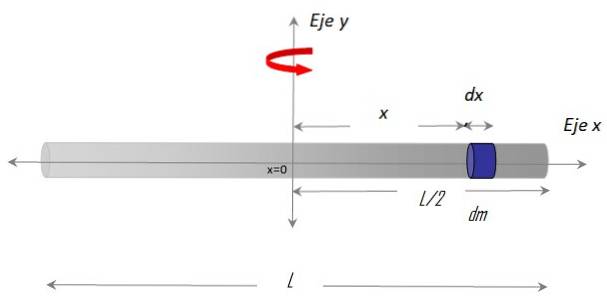
The X axis along the bar and the Axis y as the axis of rotation. The procedure for establishing the integral also requires choosing a differential of mass over the bar, called dm, which has a differential length dx and is located at the position x arbitrary, relative to the center x = 0.

According to the definition of linear mass density λ:

λ = M / L

Since the density is uniform, which is valid for M and L, it is also valid for dm and dx:

λ = dm / dx → dm = λdx.

On the other hand, the mass element is in the position x, then by substituting this geometry in the definition, we have a definite integral, whose limits are the ends of the bar according to the coordinate system:

Substituting the linear density λ = M / L:

To find the moment of inertia of the bar with respect to another axis of rotation, for example one that passes through one of its ends, you can use Steiner's theorem (see exercise solved at the end) or perform a direct calculation similar to the one shown here, but modifying the geometry appropriately.

Moment of inertia of a disk with respect to an axis passing through its center

A very thin disk of negligible thickness is a flat figure. If the mass is uniformly distributed over the entire surface of area A, the mass density σ is:

σ = M / Y

So much dm What gives correspond to the mass and the area of ​​the differential ring shown in the figure. We will assume that the whole assembly rotates around the y-axis.

You can imagine that the disk is composed of many concentric rings of radius r, each with its respective moment of inertia. Adding the contributions of all the rings until reaching the radius R, we will have the total moment of inertia of the disk.

σ = dm / dA → dm = σgives

Where M represents the entire mass of the disk. The area of ​​a disk depends on its radius r as:

A = π.r^two

Deriving with respect to r:

dA / dr = 2 = 2π.r → dA = 2π.rdr

Substituting the above in the definition of I:

Substituting σ = M / (π.R^two ) remains:

Moment of inertia of a solid sphere with respect to a diameter

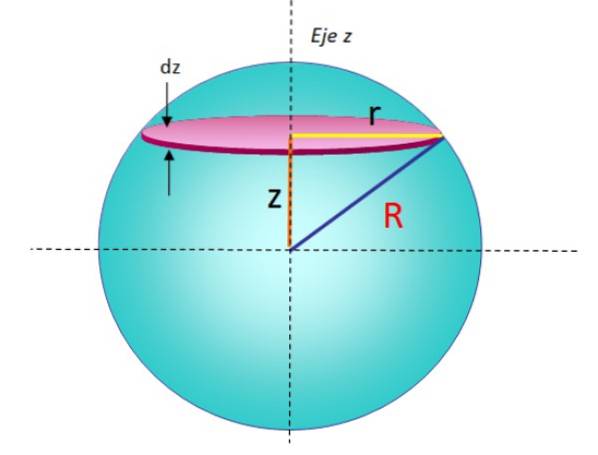
A sphere of radius R can be thought of as a series of disks stacked one on top of the other, where each disk of infinitesimal mass dm, radio r and thickness dz, has a moment of inertia given by:

gave_disk = (½) r^twodm

To find this differential, we simply took the formula from the previous section and substituted M Y R for dm Y r, respectively. A disk like this can be seen in the geometry of figure 5.

By adding all the infinitesimal moments of inertia of stacked disks, the total moment of inertia of the sphere is obtained:

I_sphere = ∫dI_disk

Which is equivalent to:

I = ∫_sphere (½) r^twodm

To solve the integral you need to express dm properly. As always, it is achieved from the density:

ρ = M / V = ​​dm / dV → dm = ρ.dV

The volume of a differential disk is:

dV = Area of ​​base x height

The height of the disc is the thickness dz, while the area of ​​the base is πr^two, Thus:

dV = πr^twodz

And substituting in the proposed integral it would look like this:

I = ∫_sphere(½) r^twodm = ∫ (½) r^two(ρπr^twodz)

But before integrating, it must be observed that r -the radius of the disk- depends on z and R -the radius of the sphere-, as can be seen from figure 5. Using the Pythagorean theorem:

R^two = r^two + z^two → r^two = R^two - z^two

 Which leads us to:

I = ∫_sphere(½) ρ r^two(πr^twodz) = ∫_sphere(½) ρ π r⁴dz= ∫_sphere(½) ρ π (R^two - z^two)^two dz

To integrate over the entire sphere, we note that z varies between -R and R, therefore:

Knowing that ρ = M / V = ​​M / [(4/3) πR³] finally it is obtained, after simplifying:

Moment of inertia of a solid cylinder with respect to the axial axis

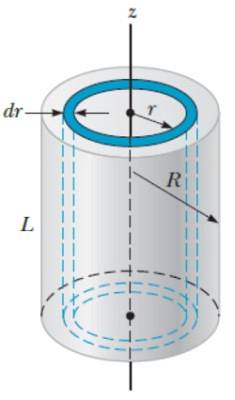
For this object a method similar to the one used for the sphere is used, only this time it is easier if the cylinder is imagined to be formed by cylindrical shells of radius r, thickness dr and height H, as if they were the layers of an onion.

The volume dV of a cylindrical layer is:

dV = 2π.rL.dr

Therefore the shell mass is:

dm = ρ.dV = ρ. 2π.r.L.dr

This expression is substituted in the definition of moment of inertia:

The above equation indicates that the moment of inertia of the cylinder does not depend on its length, but on its mass and radius only. Yes L changed, the moment of inertia with respect to the axial axis would remain the same. For this reason, I of the cylinder coincides with that of the previously calculated thin disk.

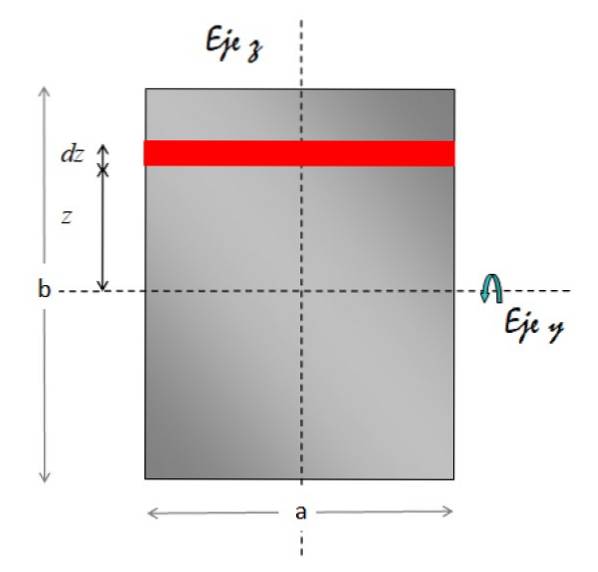
Moment of inertia of a rectangular sheet with respect to an axis passing through its center

The Axis y horizontal axis of rotation. The figure below shows the geometry required to carry out the integration:

The area element marked in red is rectangular. Its area is base x height, therefore:

dA = a.dz

Therefore the mass differential is:

dm = σ.dA = σ. (a.dz)

Regarding the distance from the area element to the axis of rotation, it is always z. We substitute all this in the integral of the moment of inertia:

Now the surface mass density σ is replaced by:

σ = M / ab

And it definitely looks like this:

Note that it is like the one with the thin bar.

Moment of inertia of a square sheet with respect to an axis passing through its center

For a side square L, in the previous expression valid for a rectangle, simply substitute the value of b for that of L:

Moment of Inertia Theorems

There are two especially useful theorems to simplify the calculation of moments of inertia about other axes, which could otherwise be difficult to find due to lack of symmetry. These theorems are:

Steiner's theorem

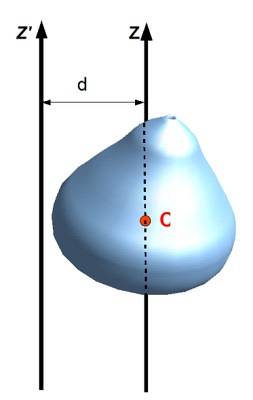
Also called parallel axes theorem, relates the moment of inertia with respect to an axis with another that passes through the center of mass of the object, as long as the axes are parallel. To apply it, it is necessary to know the distance D between both axes and of course the mass M of the object.

Be I_z the moment of inertia of an extended object with respect to the z axis, I_CM the moment of inertia with respect to an axis that passes through the center of mass (CM) of said object, then it is true that:

I_z = I_CM + MD^two

Or in the notation of the following figure: I_{z '} = I_z + Md^two

Perpendicular axes theorem

This theorem is applied to plane surfaces and goes like this: the moment of inertia of a plane object around an axis perpendicular to it is the sum of the moments of inertia around two axes perpendicular to the first axis:

I_z = I_x + I_Y

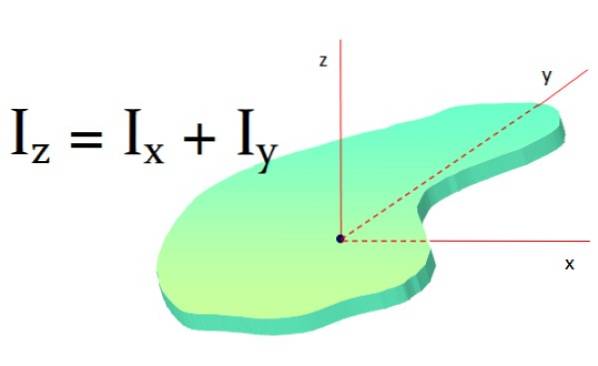
If the object has symmetry such that I_x and I_Y are equal, then it is true that:

I_z = 2I_x

Exercise resolved

Find the moment of inertia of the bar with respect to an axis that passes through one of its ends, as shown in figure 1 (below and to the right) and figure 10.

Solution:

We already have the moment of inertia of the bar around an axis that passes through its geometric center. Since the bar is homogeneous, its center of mass is at that point, so this will be our I_CM to apply Steiner's theorem.

If the length of the bar is L, the z axis is at a distance D = L / 2, therefore:

I_z = I_CM + MD^two= (1/12) ML^two+M (L / 2)^two= (1/3) ML^two

References

1. Bauer, W. 2011. Physics for Engineering and Sciences. Volume 1. Mc Graw Hill. 313-340
2. Rex, A. 2011. Fundamentals of Physics. Pearson. 190-200.
3. Parallel Axis Theorem. Recovered from: hyperphysics.phy-astr.gsu.edu.
4. Serway, R. 2018. Physics for Science and Engineering. Volume 1. Cengage.
5. Sevilla University. Spherical solids moment of inertia. Recovered from: laplace.us.es.
6. Sevilla University. Moment of inertia of a particle system. Recovered from: laplace.us.es.
7. Wikipedia. Parallel axis theorem. Recovered from: en.wikipedia.org