The irrational numbers are those whose decimal expression has infinite figures without a repeating pattern, therefore, they cannot be obtained by making the quotient between any two integers.
Among the best known irrational numbers are:
Among them, without a doubt π (pi) is the most familiar, but there are many more. All of them belong to the set of real numbers, which is the numerical set that groups rational and irrational numbers..
The ellipsis in figure 1 indicate that the decimals continue indefinitely, what happens is that the space of ordinary calculators only allows showing a few.
If we look carefully, whenever we make the quotient between two whole numbers, we obtain a decimal with limited figures or if not, with infinite figures in which one or more are repeated. Well, this does not happen with irrational numbers..
Article index
The great ancient mathematician Pythagoras, born 582 BC in Samos, Greece, founded the Pythagorean school of thought and discovered the famous theorem that bears his name. We have it down here on the left (maybe the Babylonians already knew it long before).
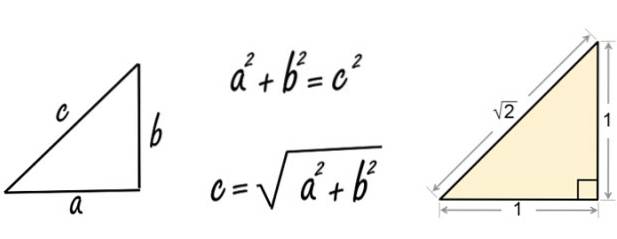
Well, when Pythagoras (or probably a disciple of his) applied the theorem to a right triangle with sides equal to 1, he found the irrational number √2.
He did it this way:
c = √1two + 1two = √1 + 1 = √2
And immediately he realized that this new number did not come from the quotient between two other natural numbers, which were the ones that were known at that time.
Therefore he called it irrational, and the discovery caused great anxiety and confusion among the Pythagoreans.
-The set of all irrational numbers is denoted by the letter I and sometimes as Q * or QC. The union between the irrational numbers I or Q * and the rational numbers Q, gives rise to the set of real numbers R.
-With irrational numbers, the known arithmetic operations can be performed: addition, subtraction, multiplication, division, empowerment and more.
-Division by 0 is also not defined between irrational numbers.
-The sum and the product between irrational numbers is not necessarily another irrational number. For example:
√2 x √8 = √16 = 4
And 4 is not an irrational number.
-However, the sum of a rational number plus an irrational number does result in an irrational. In this way:
1 + √2 = 2.41421356237…
-The product of a rational number other than 0 by an irrational number is also irrational. Let's look at this example:
2 x √2 = 2.828427125…
-The inverse of an irrational results in another irrational number. Let's try some:
1 / √2 = 0.707106781…
1 / √3 = 0.577350269…
These numbers are interesting because they are also the values of some trigonometric ratios of known angles. Most of the trigonometric ratios are irrational numbers, but there are exceptions, such as sin 30º = 0.5 = ½, which is rational.
-In addition, the commutative and associative properties are fulfilled. If a and b are two irrational numbers, this means that:
a + b = b + a.
And if c is another irrational number, then:
(a + b) + c = a + (b + c).
-The distributive property of multiplication with respect to addition is another well-known property that also holds for irrational numbers. In this case:
a. (b + c) = a.b + a.c.
-An irrational a has its opposite: -a. When they are added the result is 0:
a + (- a) = 0
-Between two different rationals, there is at least one irrational number.
The real line is a horizontal line where the real numbers are located, of which the irrationals are an important part.
To find an irrational number on the real line, in geometric form, we can use the Pythagorean theorem, a ruler and a compass.
As an example we are going to locate √5 on the real line, for which we draw a right triangle with sides x = 2 Y y = 1, as the picture shows:
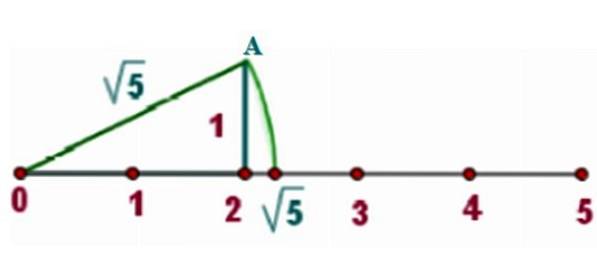
By the Pythagorean theorem, the hypotenuse of such a triangle is:
c = √2two + 1two = √4 + 1 = √5
Now the compass is placed with the point at 0, where one of the vertices of the right triangle is also. The point of the compass pencil should be at vertex A.
An arc of circumference is drawn that cuts to the real line. Since the distance between the center of the circumference and any point on it is the radius, which is equal to √5, the intersection point is also far √5 from the center.
From the graph it can be seen that √5 is between 2 and 2.5. A calculator gives us the approximate value of:
√5 = 2.236068
And so, by building a triangle with the appropriate sides, other irrational ones can be located, such as √7 and others.
Irrational numbers are classified into two groups:
-Algebraic
-Transcendent or transcendental
Algebraic numbers, which may or may not be irrational, are solutions of polynomial equations whose general form is:
ton xn + ton-1xn-1 + ton-2xn-2 +…. + a1x + aor = 0
An example of a polynomial equation is a quadratic equation like this:
x3 - 2x = 0
It is easy to show that the irrational number √2 is one of the solutions of this equation.
On the other hand, the transcendent numbers, although they are irrational, never arise as a solution of a polynomial equation.
The transcendent numbers found most frequently in applied mathematics are π, due to its relationship with the circumference and the number e, or Euler's number, which is the base of natural logarithms..
A gray square is placed on a black square in the position indicated in the figure. The surface of the black square is known to be 64 cmtwo. How much are the lengths of both squares?
The area of a square with side L is:
A = Ltwo
Since the black square is 64 cmtwo of area, its side should be 8 cm.
This measurement is the same as the diagonal of the gray square. Applying the Pythagorean theorem to this diagonal, and remembering that the sides of a square measure the same, we will have:
8two = Lgtwo + Lgtwo
Where Lg is the side of the gray square.
Therefore: 2Lgtwo = 8two
Applying square root to both sides of the equality:
Lg = (8 / √2) cm
Yet No Comments