The mechanical power is the rate at which work is done, which is expressed mathematically by the amount of work done per unit of time. And since the work is done at the expense of absorbed energy, it can also be stated as energy per unit of time.
Calling P to power, W to work, AND to energy and t At the same time, all of the above can be summarized in easy-to-use mathematical expressions:
O well:
Other power units used in industries are hp (horse-power or horsepower) and CV (horsepower). The origin of these units also dates back to James Watt and the Industrial Revolution, when the standard of measurement was the rate at which a horse did work..
Both hp and CV are approximately equal to ¾ kilo-W and are still widely used, especially in mechanical engineering, for example in the designation of motors..
Multiples of the watt, such as the aforementioned kilo-W = 1000 W are also often used in electrical power. This is because the joule is a relatively small unit of energy. The British system uses pound-feet / second.
Article index
The concept of power is applicable to all types of energy, whether mechanical, electrical, chemical, wind, sonic or of any kind. Time is very important in the industry, because processes must be executed as quickly as possible.
Any motor will do the necessary work as long as it has enough time, but the important thing is to do it in the shortest possible time, to increase efficiency..
A very simple application is immediately described to clarify well the distinction between work and power.
Suppose a heavy object is pulled by a rope. To do this, some external agent is required to do the necessary work. Let's say that this agent transfers 90 J of energy to the object-string system, so that it sets in motion for 10 seconds.
In such a case, the energy transfer rate is 90 J / 10 s or 9 J / s. Then we can affirm that that agent, a person or a motor, has an output power of 9 W.
If another external agent is capable of achieving the same displacement, either in less time or by transferring less amount of energy, then it is capable of developing greater power..
Another example: suppose an energy transfer of 90 J, which manages to set the system in motion for 4 seconds. The output power will be 22.5W.
Power is closely related to performance. The energy supplied to a machine is never completely transformed into useful work. An important part is usually dissipated in heat, which depends on many factors, for example the design of the machine.
That is why it is important to know the performance of the machines, which is defined as the quotient between the work delivered and the energy supplied:
η = work delivered by the machine / energy supplied
Where the greek letter η denotes the yield, a dimensionless quantity that is always less than 1. If it is also multiplied by 100, we have the yield in percentage terms.
- Humans and animals develop power during locomotion. For example, climbing stairs requires work against gravity. Comparing two people climbing a ladder, the one who climbs all the steps first, will have developed more power than the other, but both did the same job.
- Household appliances and machinery have their output power specified. An incandescent bulb suitable for lighting a room well has a power of 100 W. This means that the bulb transforms electrical energy into light and heat (most of it) at a rate of 100 J / s.
- The motor of a lawn mower can consume about 250 W and that of a car is on the order of 70 kW.
- A homemade water pump usually supplies 0.5 hp.
- The sun generates 3.6 x 10 26 Wattage.
Instantaneous power is obtained by taking an infinitesimal time: P = dW / dt. The force that produces the work causing the small infinitesimal displacement dx it is F (both are vectors), therefore dW = F ● dx. Substituting everything in the expression for the power, it remains:
People are capable of generating power of around 1500 W or 2 horsepower, at least for a short time, such as lifting weights.
On average, the daily power output (8 hours) is 0.1 hp per person. Much of which is translated into heat, roughly the same amount generated by a 75W incandescent light bulb.
An athlete in training can generate an average of 0.5 hp equivalent to approximately 350 J / s, by transforming chemical energy (glucose and fat) into mechanical energy.
When it comes to human power, it is generally preferred to measure in kilo-calories / hour, rather than watts. The necessary equivalence is:
1 kilocalorie = 1 nutritional calorie = 4186 J
A power of 0.5 hp sounds like a very small amount, and it is for many applications.
However, in 1979 a human-powered bicycle was created that could fly. Paul MacCready designed the Gossamer Albatross, that crossed the English Channel generating 190 W of average output (figure 1).
An important application is the distribution of electrical energy between users. The companies that supply electricity bill for the energy consumed, not the rate at which it is consumed. That is why those who read your bill carefully will find a very specific unit: the kilowatt-hour or kW-h.
However, when the Watt name is included in this unit it refers to energy and not power..
The kilowatt-hour is used to indicate the consumption of electrical energy, since the joule, as mentioned before, is a fairly small unit: 1 watt-hour or W-h is the work done in 1 hour using a power of 1 watt.
Therefore 1 kW-h is the work that is done in an hour working with a power of 1kW or 1000 W. Let's put the numbers to convert these amounts to joules:
1 W-h = 1 W x 3600 s = 3600 J
1 kW-h = 1000 W x 3600 s = 3.6 x 10 6 J
It is estimated that a household can consume about 200 kW-hours per month.
A farmer uses a tractor to pull a bale of hay M = 150 kg over a 15 ° incline and bring it to the barn at a constant speed of 5.0 km / h. The coefficient of kinetic friction between the hay bale and the chute is 0.45. Finding the power output of the tractor.
For this problem, it is necessary to draw a free-body diagram for the bale of hay that rises on the incline. Be F the force applied by the tractor to raise the bale, α = 15º is the angle of inclination.
In addition, the kinetic friction force is involved Ftouch that opposes movement, plus the normal N and the weight W (do not confuse the W of weight with that of work).
Newton's second law offers the following equations:
∑ Fx = F -Wx -Ftouch = 0 (since the bale rises at a constant speed)
∑Fy = N - WY = 0 (there is no movement along the x axis)
The kinetic friction force is calculated by:
Ftouch = coefficient of kinetic friction x magnitude of normal
Ftouch = 0.45. Wy = 0.45 x 150 kg x9.8 m / s2 x cos 15º = 639 N
F = Wx + Ftouch = M.g. sin α = 150 kg. 9.8 m / stwo . sin 15º + 639 N = 1019.42 N
Speed and force have the same direction and sense, therefore:
P = F ● v = F. v
It is required to transform the units of the velocity:
v = 5.0 km / h = 1.39 m / s
Substituting values, finally we get:
P = 1019.42 N x 1.39 m / s = 1417 W = 1.4 kW
The motor shown in the figure will lift the 2 kg block, starting from rest, with an acceleration of 2 m / stwo and in 2 seconds.
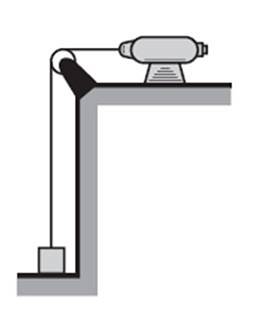
Calculate:
a) The height reached by the block in that time.
b) The power that the engine must develop to achieve this.
a) It is a uniformly varied rectilinear motion, therefore the corresponding equations will be used, with initial velocity 0. The height reached is given by:
y = ½ attwo = ½. 2 m / stwo . (2 s)two = 4 m.
b) To find the power developed by the motor, the equation can be used:
P = ΔW / Δt
And since the force exerted on the block is through the tension in the string, which is constant in magnitude:
P = (ma) .y / Δt = 2 kg x 2 m / stwo x 4 m / 2 s = 8 W
Yet No Comments