The atomic radio it is an important parameter for the periodic properties of the elements of the periodic table. It is directly related to the size of the atoms, since the greater the radius, the larger or more voluminous they are. Likewise, it is related to their electronic characteristics..
The more electrons an atom has, the larger its atomic size and radius. Both are defined by the electrons of the valence shell, because at distances beyond their orbits, the probability of finding an electron approaches zero. The opposite occurs in the vicinity of the nucleus: the probability of finding an electron increases.
The upper image represents a packing of cotton balls. Note that each one is surrounded by six neighbors, not counting another possible upper or lower row. The way in which the cotton balls are compacted will define their sizes and, therefore, their radii; just like atoms.
The elements according to their chemical nature interact with their own atoms in one way or another. Consequently, the magnitude of the atomic radius varies according to the type of bond present and the solid packing of its atoms..
Article index
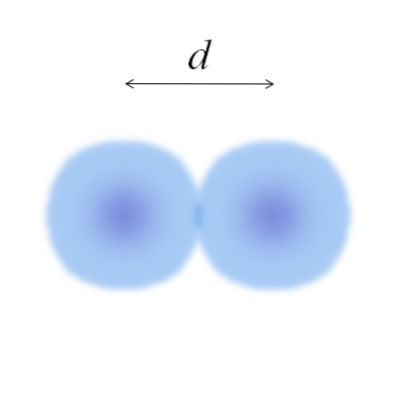
In the main image it can be easy to measure the diameter of the cotton balls, and then divide it by two. However, the sphere of an atom is not fully defined. Why? Because electrons circulate and diffuse in specific regions of space: orbitals.
Therefore, the atom can be considered as a sphere with impalpable edges, which it is impossible to say for sure how far they end. For example, in the image above, the region of the center, close to the nucleus, shows a more intense color, while its edges are blurred.
The image represents a diatomic molecule Etwo (like the Cltwo, Htwo, ORtwo, etc.). Assuming that the atoms are spherical bodies, if the distance were determined d that separates both nuclei in the covalent bond, it would then suffice to divide it into two halves (d/ 2) to obtain the atomic radius; more precisely, the covalent radius of E to Etwo.
What if E did not form covalent bonds with itself, but was a metallic element? Then d it would be indicated by the number of neighbors that surround E in its metallic structure; that is, by the coordination number (N.C) of the atom inside the packaging (remember the cotton balls in the main image).
To determine d, which is the internuclear distance for two atoms in a molecule or packaging, physical analysis techniques are required.
One of the most widely used is X-ray diffraction. In it, a beam of light is irradiated through a crystal, and the diffraction pattern resulting from the interactions between electrons and electromagnetic radiation is studied. Depending on the packing, different diffraction patterns can be obtained and, therefore, other values of d.
If the atoms are "tight" in the crystal lattice, they will present different values of d compared to what they would have if they were "comfortable." Also, these internuclear distances could fluctuate in values, so the atomic radius actually consists of an average value of such measurements..
How are the atomic radius and the coordination number related? V. Goldschmidt established a relationship between the two, in which for an N.C of 12, the relative value is 1; 0.97 for a packing where the atom has N.C equal to 8; 0.96, for an N.C equal to 6; and 0.88 for an N.C of 4.
From the values for N.C equal to 12, many of the tables have been constructed where the atomic radii of all the elements of the periodic table are compared.
As not all elements form such compact structures (N.C less than 12), the V. Goldschmidt relation is used to calculate their atomic radii and express them for the same packing. In this way the measurements of the atomic radii are standardized..
But in what units are they expressed? Given the d is of very small magnitude, we must resort to the angstrom units Å (10 ∙ 10-10m) or also widely used, the picometer (10 ∙ 10-12m).
The atomic radii determined for metallic elements are called metallic radii, while for non-metallic elements, covalent radii (such as phosphorus, P4, or sulfur, S8). However, between the two types of spokes there is a more prominent distinction than that of the name.
From left to right in the same period, the nucleus adds protons and electrons, but the latter are confined to the same energy level (principal quantum number). As a consequence, the nucleus exerts an increasing effective nuclear charge on the valence electrons, which contracts the atomic radius..
In this way, non-metallic elements in the same period tend to have smaller atomic (covalent) radii than metals (metallic radii).
As you descend through a group, new energy levels are enabled, which allow the electrons to have more space. Thus, the electronic cloud covers greater distances, its blurred periphery ends up moving further away from the nucleus, and therefore, the atomic radius expands..
The electrons in the inner shell help shield the effective nuclear charge on the valence electrons. When the orbitals that make up the inner shells have many "holes" (nodes), as occurs with the f orbitals, the nucleus strongly contracts the atomic radius due to their poor shielding effect..
This fact is evidenced in the lanthanide contraction in period 6 of the periodic table. From La to Hf there is a considerable contraction of the atomic radius product of the f orbitals, which “fill up” as the f block is traversed: that of the lanthanoids and actinoids.
A similar effect can also be observed with the elements of block p from period 4. This time, product of the weak shielding effect of the d orbitals that fill up when passing through the transition metal periods..
For period 2 of the periodic table the atomic radii of its elements are:
-Li: 257 pm
-Be: 112 pm
-B: 88 pm
-C: 77 pm
-N: 74 pm
-Or: 66 pm
-F: 64 pm
Note that lithium metal has the largest atomic radius (257 pm), while fluorine, located at the extreme right of the period, is the smallest of them all (64 pm). The atomic radius descends from left to right in the same period, and the listed values show it.
Lithium, when forming metallic bonds, its radius is metallic; and fluorine, as it forms covalent bonds (F-F), its radius is covalent.
What if you want to express the atomic radii in units of angstrom? Simply divide them by 100: (257/100) = 2.57Å. And so on with the rest of the values.
Yet No Comments