
History triangles, elements, classification, properties

The triangles They are flat and closed geometric figures, consisting of three sides. A triangle is determined by three lines that intersect two by two, forming three angles with each other. The triangular shape, full of symbolism, is present in countless objects and as an element of construction.
The origin of the triangle is lost in history. From archaeological evidence it is known that primitive humanity knew it well, as archaeological remains confirm that it was used in tools and weapons.

It is also clear that the ancient Egyptians had a solid knowledge of geometry and in particular of the triangular shape. They were reflected in the architectural elements of its monumental buildings.
In the Rhind papyrus are formulas for the calculation of areas of triangles and trapezoids, as well as some volumes and other concepts of rudimentary trigonometry.
For their part, it is known that the Babylonians were able to calculate the area of the triangle and other geometric figures, which they used for practical purposes, such as land divisions. They were also knowledgeable about many properties of triangles.
However, it was the ancient Greeks who systematized many of the geometric concepts prevalent today, although much of this knowledge was not exclusive, since it was surely shared with these other ancient civilizations..
Article index
- 1 Elements of the triangle
- 1.1 Notation
- 2 Types of triangles
- 2.1 Congruence of triangles
- 2.2 Similarity of triangles
- 3 Properties
- 4 Theorems
- 4.1 Thales' First Theorem
- 4.2 Thales' second theorem
- 4.3 The Pythagorean theorem
- 5 The area of a triangle
- 6 Examples of triangles
- 6.1 Example 1
- 6.2 Example 2
- 6.3 Example 3
- 7 Exercises
- 7.1 Exercise 1
- 7.2 Exercise 2
- 8 References
Triangle elements
The elements of any triangle are indicated in the following figure. There are three: vertices, sides and angles.
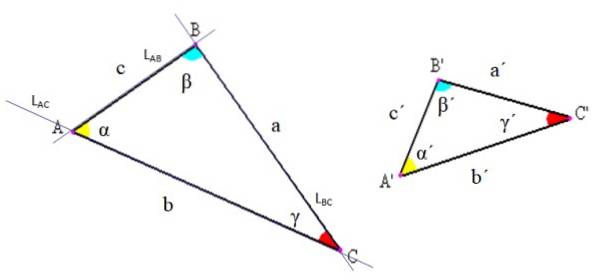
-Vertices: are the points of intersection of the lines whose segments determine the triangle. In the figure above, for example, the line LAC containing segment AC, intersects line LAB containing segment AB just at point A.
-Sides: between each pair of vertices a line segment is drawn that constitutes one side of the triangle. This segment can be denoted by the end letters or by using a specific letter to call it. In the example in figure 2, side AB is also called "c".
-Angles: Between each side with a common vertex an angle originates, whose vertex coincides with that of the triangle. Generally the angle is denoted with a Greek letter, as it was said at the beginning.
To build a particular triangle, with a given shape and size, just have one of the following data sets:
-All three sides, quite obvious for a triangle.
-Two sides and the angle between them, and immediately the remaining side is drawn.
-Two (internal) angles and the side between them. By extension the two missing sides are drawn and the triangle is ready.
Notation
Generally, in triangle notation, the following conventions are used: vertices are indicated by uppercase Latin letters, sides by lowercase Latin letters, and angles by Greek letters (see figure 2).
In this way the triangle is named according to its vertices. For example, the triangle on the left in figure 2 is triangle ABC, and the one on the right is triangle A'B'C '.
It is also possible to use other notations; for example, the angle α in Figure 2 is denoted as BAC. Note that the letter of the vertex goes in the middle and the letters are written in an anti-clockwise direction.
Other times a caret is placed to denote the angle:
α = ∠A
Types of triangles
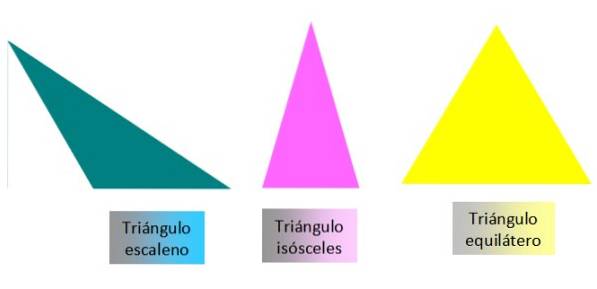
There are several criteria for classifying triangles. The most usual thing is to classify them according to the measure of their sides or according to the measure of their angles. Depending on the measure of their sides, the triangles can be: scalenes, isosceles or equilateral:
-Scalene: its three sides are different.
-Isosceles: has two equal sides and one different side.
-Equilateral: all three sides are equal.

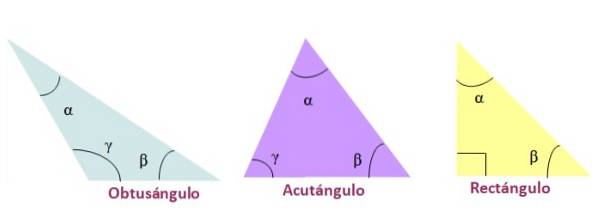
According to the measure of their angles, the triangles are named like this:
-Obtuse angle, if one of the internal angles is greater than 90º.
-Acute angle, when the three internal angles of the triangle are acute, that is, less than 90º
-Rectangle, in case one of its internal angles is 90º. The sides that form 90º are called legs and the side opposite the right angle is the hypotenuse.
Congruence of triangles
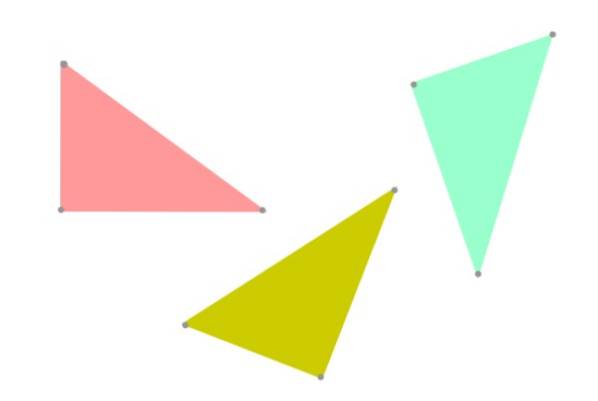
When two triangles have the same shape and are the same size, they are said to be congruent. Of course congruence is related to equality, so why in geometry do we speak of "two congruent triangles" instead of "two equal triangles"?
Well, it is preferred to use the term "congruence" to stick to the truth, since two triangles can have the same shape and size, but be oriented differently in the plane (see figure 3). From the point of view of geometry, they would no longer be strictly the same.
Congruence criteria
Two triangles are congruent if any of the following occurs:
-All three sides measure the same (again this is the most obvious).
-They have two identical sides and with the same angle between them.
-Both have two identical internal angles and the side between these angles measures the same.
As can be seen, it is about the two triangles meeting the necessary conditions so that when they are built, their shape and size are exactly the same..
The congruence criteria are very useful, since in practice, innumerable pieces and mechanical parts must be manufactured in series, in such a way that their measurements and shape are exactly the same.
Similarity of triangles
A triangle is similar to another if they have the same shape, even if they are of different sizes. To make sure that the shape is the same, it is required that the internal angles have the same value and that the sides be proportional..
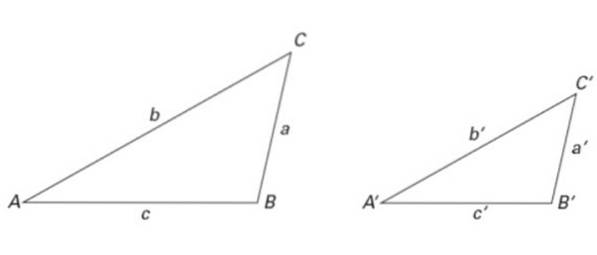
The triangles in figure 2 are also similar, as are those in figure 6. In this way:
∠ A = ∠ A ', ∠ B = ∠ B 'and ∠ C = ∠ C '
As for the sides, the following similarity ratios hold:
a / a '= b / b' = c / c '
Properties
The fundamental properties of triangles are as follows:
-The sum of the interior angles of any triangle is always 180º.
-For any triangle, the sum of its exterior angles is equal to 360 °.
- An external angle of a triangle is equal to the sum of the two interior angles not adjacent to said angle.
Theorems
Thales' First Theorem
They are attributed to the Greek philosopher and mathematician Thales of Miletus, who developed several theorems related to geometry. The first of them establishes the following:
If several parallel lines intersect two transverse lines, segments that are proportional are determined in them.
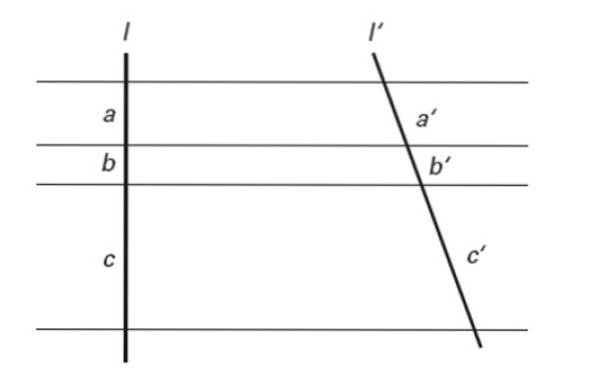
In other words:
a / a '= b / b' = c / c '
Thales' first theorem is applicable to a triangle, for example we have the blue triangle ABC on the left, which is cut by the red parallels on the right:
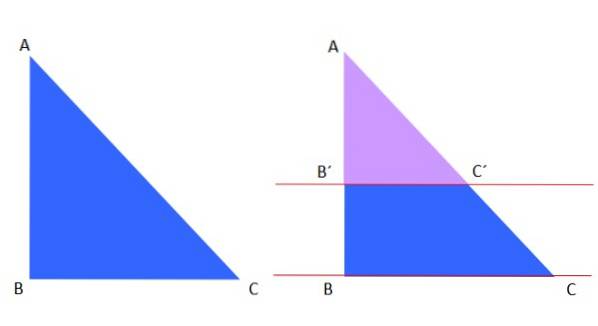
The violet triangle AB'C 'is similar to the blue triangle ABC, therefore, according to Thales' theorem, the following can be written:
AB '/ AC' = AB / AC
And it is consistent with what was explained previously in the segment of the similarity of triangles. By the way, parallel lines can also be vertical or parallel to the hypotenuse and similar triangles are obtained in the same way.
Thales' second theorem
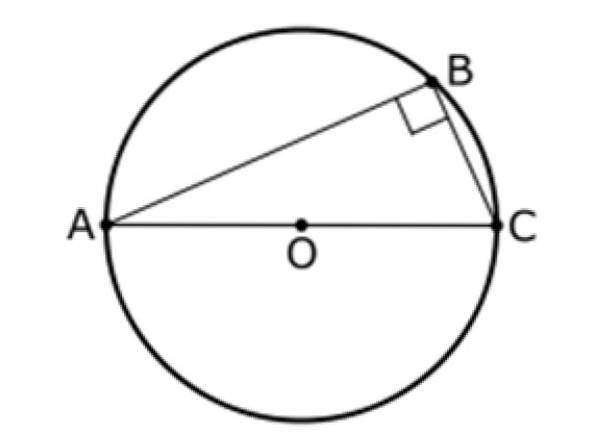
This theorem also refers to a triangle and a circle with center O, like the ones shown below. In this figure, AC is a diameter of the circumference and B is a point on it, B being different from A and B.
Thales' second theorem states that:
The angle between the segments AB and BC is always 90º, therefore the triangle ABC is right.
The Pythagorean theorem
This is one of the most famous theorems in history. It is due to the Greek mathematician Pythagoras of Samos (569 - 475 BC) and is applicable to a right triangle. Says so:
The sum of the squares of the lengths of the legs of the right triangle is equal to the length of the hypotenuse squared.
If we take as an example the blue triangle in figure 8, or the violet triangle, since both are rectangles, then it can be stated that:
ACtwo = ABtwo + BCtwo (blue triangle)
AC 'two = AB 'two + BC 'two (purple triangle)
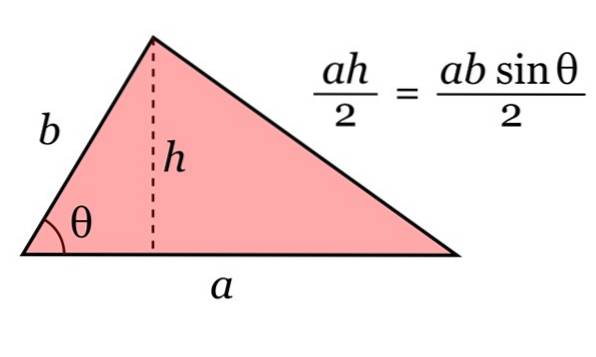
The area of a triangle
The area of the triangle is given by the product of its base to and your height h, divided by 2. And by trigonometry, this height can be written as h = b sinθ.
Examples of triangles
Example 1
It is said that by means of his first theorem, Thales managed to measure the height of the Great Pyramid in Egypt, one of the 7 wonders of the ancient world, by measuring the shadow that it projected on the ground and that projected by a stake driven into the ground..
This is the outline of the procedure followed by Tales:
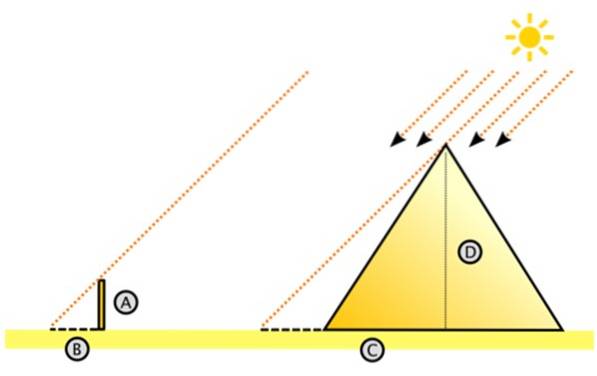
Thales correctly assumed that the sun's rays strike in parallel. With this in mind, he imagined the large right triangle on the right.
There D is the height of the pyramid and C is the distance above the ground measured from the center to the shadow cast by the pyramid on the desert floor. It may be laborious to measure C, but it is certainly easier than measuring the height of the pyramid.
On the left is the small triangle, with legs A and B, where A is the height of the stake driven vertically into the ground and B is the shadow it casts. Both lengths are measurable, as is C (C is equal to the length of the shadow + half the length of the pyramid).
So, by similarity of triangles:
A / B = D / C
And the height of the Great Pyramid turns out to be: D = C. (A / B)
Example 2
Trusses in civil construction are structures made from crisscrossed straight thin bars of wood or metal, which are used as support in many buildings. They are also known as lattices, trusses or lattices (truss in English).
In them the triangles are always present, since the bars are interconnected at points called nodes, which can be fixed or articulated..

Example 3
The method known as triangulation allows obtaining the location of inaccessible points knowing other distances that are easier to measure, provided that a triangle is formed that includes the desired location between its vertices..
For example, in the following figure we want to know where the ship is in the sea, denoted as B.
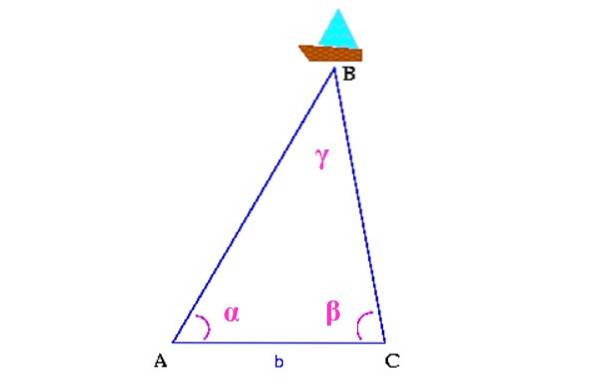
First, the distance between two points on the coast is measured, which in the figure are A and C. Next, the angles α and β must be determined, with the help of a theodolite, a device used to measure vertical and horizontal angles.
With all this information, a triangle is built in whose upper vertex is the ship. It would remain to calculate the angle γ, using the properties of the triangles and the distances AB and CB using trigonometry, to determine the position of the ship in the sea.
Training
Exercise 1
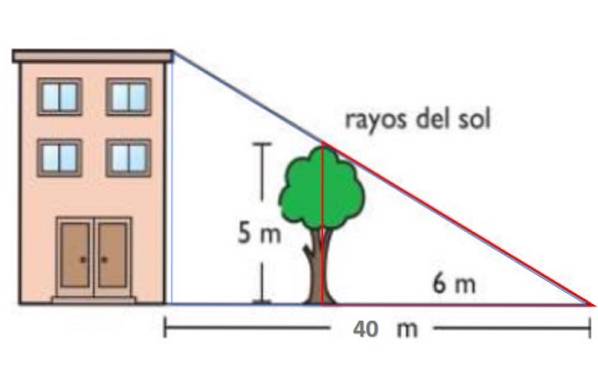
In the figure shown, the sun's rays are parallel. In this way the 5 meter tall tree casts a 6 meter shadow on the ground. At the same time, the shadow of the building is 40 meters. Following Thales' First Theorem, find the height of the building.
Solution
The red triangle has sides of 5 and 6 meters respectively, while the blue one has height H - the height of the building - and base 40 meters. Both triangles are similar, therefore:
H / 40 = 5/6 → H = 40. (5/6) m = 33.3 m
Exercise 2
You need to know the horizontal distance between two points TO Y B, but they are located on a very uneven ground.
About the midpoint (Pm) of this land stands out a prominence of 1.75 meters high. If the tape measure indicates 26 meters in length measured from A to prominence, and 27 meters from B to the same point, find the distance AB.
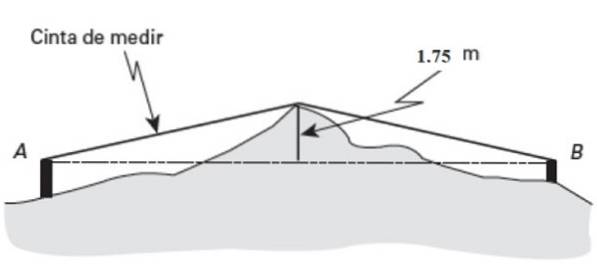
Solution
The Pythagorean theorem is applied to one of the two right triangles in the figure. Starting with the one on the left:
Hypotenuse = c = 26 meters
Height = a = 1.75 meters
APm = (26two - 1.75two)1/2 = 25.94 m
Now apply Pythagoras in the triangle on the right, this time c = 27 meters, a = 1.75 meters. With these values:
BPm= (27two - 1.75two)1/2 = 26.94 m
The distance AB is found by adding these results:
AB = 25.94 m + 26.94 m = 52.88 m.
References
- Baldor, J. A. 1973. Plane and Space Geometry. Central American Cultural.
- Barredo, D. The geometry of the triangle. Recovered from: ficus.pntic.mec.es.
- Jiménez, R. 2010. Mathematics II. Geometry and trigonometry. Second edition. Pearson.
- Wentworth, G. Plane Geometry. Recovered from: gutenberg.org.
- Wikipedia. Triangle. Recovered from: es. wikipedia.org.



Yet No Comments