
Ordinal variable


What is an ordinal variable?
An ordinal variable is one that takes values that can be ordered (or denote an order). For example, the variable height of a person can be classified into: tall, average and short.
An ordinal scale, in addition to identifying, grouping and differentiating the study units, as a nominal variable, also describes the magnitude and, therefore, is characterized by the ordering; that is, the units of study can be ordered in increasing or decreasing order in terms of the magnitude.
In this scale we speak of first, second, third, or high, medium, low; but no measure is established between classes. For example: the variable rating in a test of satisfaction with a service provided can be measured in four categories: dissatisfied, indifferent, satisfied, very satisfied.
It can be ordered according to the satisfaction expressed, but it is not known how different it is satisfied from very satisfied, nor is it known if the difference between dissatisfied and indifferent is the same as between satisfied and very satisfied.
The ordinal scale refers to measurements that only make “greater”, “less” or “equal” comparisons between consecutive measurements. Represents a classification or ordering of a set of observed values.
Ordinal variable concept and characteristics
On an ordinal scale, the observations are placed in a relative order with respect to the characteristic being evaluated. That is, the data categories are classified or ordered according to the special characteristic they possess.
If we use numbers, the magnitude of these represents the order of the rank of the observed attribute. Only the relationships "greater than", "less than" and "equal to" have meaning on an ordinal scale of measurement..
From a mathematical point of view, and like nominal scales, ordinal scales only support the calculation of proportions, percentages and ratios.
The measure of central tendency that best explains an ordinal variable is the median, which is the value that is located in the center of the data set ordered from lowest to highest.
When objects are classified by a characteristic, it is possible to establish which object has more or less of the characteristic compared to another; but you can't quantify the difference.
For example, three objects that have been ordered as 'first', 'second' and 'third', taking into account some characteristic. Second place differs from first by an amount that is not necessarily equal to the amount by which it differs from third place.
Examples of ordinal variables

Some examples of ordinal variables:
- Social class (A - High; B - medium high; C - medium; D - low; E - very low).
- Qualitative school marks (I - insufficient; A - acceptable; B - good; S - excellent; E - excellent).
- Military ranks (General, Colonel, Lieutenant Colonel, Major, Captain, etc.).
- Degree of education (primary, high school, professional technician, technologist, university, etc.).
- Stage of development of a human being (newborn, baby, child, young, adult, elderly).
- Film classification (A - All public; B - over 12 years old; C - over 18 years old; D - Over 21 years old).
- Ripening of a fruit (green, pintona, ripe, very ripe, rotten).
- Degree of satisfaction with the provision of a public service. (Very satisfied; Satisfied; Indifferent; etc.).
- Examples explained
Evaluation of a teacher by his students
The students of a certain course have the possibility of filling out an evaluation survey of the pedagogical capacity of their teacher, which is measured with an ordinal variable whose scale is: 5 - Excellent, 4 - Good, 3 - Average, 2 - Poor , 1 - Poor.
The values of the variable are ordered from highest or best to lowest or worst: excellent is better than good, good is better than average, etc. However, it is not possible to distinguish the magnitude of the differences.
Is the difference between excellent and good the same as that between bad and poor? It is not possible to affirm.
If we use numbers, they do not indicate magnitude. For example, it should not be concluded that the rating Good (rating 4) is twice as high as Bad (rating 2). It can only be said that the Good rating is better than the Bad rating, but it cannot be quantified to what extent it is better..
Acceptance level of a meal
A tasting contest evaluates foods in a cooking contest by means of the ordinal variable in acceptance level expressed in: A - Excellent, B - Good, C - Not acceptable. The use of a measurement scale ordered from highest to lowest is evidenced, but it is not possible to establish the difference between the values of the scale.
Since it is a contest, how is the winner determined? It seems that the most appropriate is the use of fashion to make the decision about the winner of the contest. Understand the mode as the name given to the highest value (the most frequent) of the counts per level. For example, 5 A's, 14 B's, 10 C's were counted; the mode is B, since it is the level that had the most opinions.
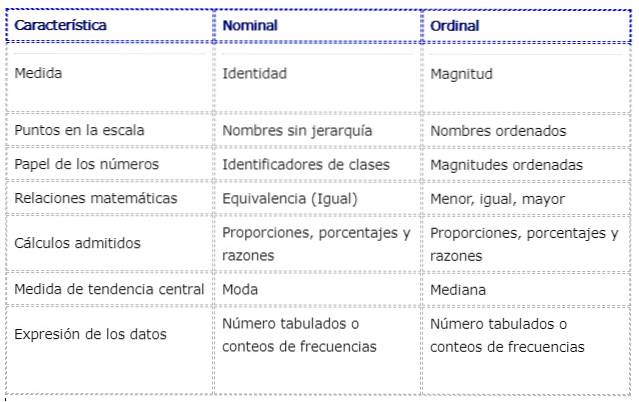
Differences with nominal variable
The following table shows some differences and similarities between the variables measured in nominal and ordinal scale:
References
- Freund, R .; Wilson, W .; Mohr, D. (2010). Statistical methods. Third ed. Academic Press-Elsevier Inc.
- Glass, G .; Stanley, J. (1996). Statistical methods not applied to the social sciences. Prentice Hall Hispanoamericana S. A.
- Cute.; Marchal, W .; Wathen, S. (2012). Statistics applied to business and economy. Fifteenth ed. McGraw-Hill / Interamericana Editores S. A.
- Orlandoni, G. (2010). Statistical measurement scales. Telos Magazine. Recovered from ojs.urbe.edu.
- Siegel, S .; Castellan, N. (1998). Nonparametric statistics applied to the behavioral sciences. Fourth ed. Editorial Trillas S. A.
- Wikipedia. (2019). Level of measurement. Recovered from en.wikipedia.org.



Yet No Comments